Published online by Cambridge University Press: 04 November 2019
For a set x, let 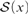 ${\cal S}\left( x \right)$ be the set of all permutations of x. We prove by the method of permutation models that the following statements are consistent with ZF:
${\cal S}\left( x \right)$ be the set of all permutations of x. We prove by the method of permutation models that the following statements are consistent with ZF:
(1) There is an infinite set x such that 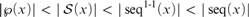 $|\wp \left( x \right)| < |{\cal S}\left( x \right)| < |se{q^{1 - 1}}\left( x \right)| < |seq\left( x \right)|$, where
$|\wp \left( x \right)| < |{\cal S}\left( x \right)| < |se{q^{1 - 1}}\left( x \right)| < |seq\left( x \right)|$, where  $\wp \left( x \right)$ is the power set of x, seq (x) is the set of all finite sequences of elements of x, and seq1-1 (x) is the set of all finite sequences of elements of x without repetition.
$\wp \left( x \right)$ is the power set of x, seq (x) is the set of all finite sequences of elements of x, and seq1-1 (x) is the set of all finite sequences of elements of x without repetition.
(2) There is a Dedekind infinite set x such that 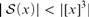 $|{\cal S}\left( x \right)| < |{[x]^3}|$ and such that there exists a surjection from x onto
$|{\cal S}\left( x \right)| < |{[x]^3}|$ and such that there exists a surjection from x onto 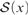 ${\cal S}\left( x \right)$.
${\cal S}\left( x \right)$.
(3) There is an infinite set x such that there is a finite-to-one function from 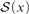 ${\cal S}\left( x \right)$ into x.
${\cal S}\left( x \right)$ into x.