Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-26T12:19:17.307Z Has data issue: false hasContentIssue false
  • English
  • Français

THE EIGHTFOLD WAY

Published online by Cambridge University Press:  01 May 2018

JAMES CUMMINGS ,
SY-DAVID FRIEDMAN ,
MENACHEM MAGIDOR ,
ASSAF RINOT  and
DIMA SINAPOVA
JAMES CUMMINGS
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES CARNEGIE MELLON UNIVERSITY PITTSBURGH, PA15213-3890, USA E-mail:[email protected]: http://www.math.cmu.edu/users/jcumming/
SY-DAVID FRIEDMAN
Affiliation:
KURT GÖDEL RESEARCH CENTER FOR MATHEMATICAL LOGIC UNIVERSITY OF VIENNAA-1090VIENNA, AUSTRIA E-mail:[email protected]: http://www.logic.univie.ac.at/∼sdf/
MENACHEM MAGIDOR
Affiliation:
EINSTEIN INSTITUTE OF MATHEMATICS THE HEBREW UNIVERSITY OF JERUSALEM JERUSALEM91904, ISRAEL E-mail:[email protected]
ASSAF RINOT
Affiliation:
DEPARTMENT OF MATHEMATICS BAR-ILAN UNIVERSITY RAMAT-GAN 5290002, ISRAEL E-mail:[email protected]: http://www.assafrinot.com
DIMA SINAPOVA
Affiliation:
DEPARTMENT OF MATHEMATICS, STATISTICS, AND COMPUTER SCIENCE UNIVERSITY OF ILLINOIS AT CHICAGO CHICAGO, IL 60607-7045, USA E-mail: [email protected]: http://homepages.math.uic.edu/∼sinapova
Get access Rights & Permissions [Opens in a new window]

Abstract

Three central combinatorial properties in set theory are the tree property, the approachability property and stationary reflection. We prove the mutual independence of these properties by showing that any of their eight Boolean combinations can be forced to hold at 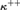${\kappa ^{ + + }}$, assuming that 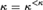$\kappa = {\kappa ^{ < \kappa }}$ and there is a weakly compact cardinal above κ.

If in addition κ is supercompact then we can force κ to be 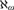${\aleph _\omega }$ in the extension. The proofs combine the techniques of adding and then destroying a nonreflecting stationary set or a ${\kappa ^{ + + }}$-Souslin tree, variants of Mitchell’s forcing to obtain the tree property, together with the Prikry-collapse poset for turning a large cardinal into 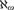${\aleph _\omega }$.

Keywords

Primary 03E35Secondary 03E55squareAronszajn treetree propertystationary reflectionapproachabilityMitchell forcingPrikry forcing
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 1 , March 2018 , pp. 349 - 371
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abraham, U., Aronszajn trees on ${\aleph _2}$ and ${\aleph _3}$. Annals of Pure and Applied Logic, vol. 24 (1983), no. 3, pp. 213–230.Google Scholar
Baumgartner, J. E., A new class of order types. Annals of Mathematical Logic, vol. 9 (1976), no. 3, 187–222.CrossRefGoogle Scholar
Baumgartner, J. E., Iterated forcing, Surveys in Set Theory (Mathias, A. R. D., editor), London Mathematical Society Lecture Note Series, vol. 87, Cambridge University Press, Cambridge, 1983, pp. 1–59.Google Scholar
Cummings, J., Notes on singular cardinal combinatorics. Notre Dame Journal of Formal Logic, vol. 46 (2005), no. 3, pp. 251–282.CrossRefGoogle Scholar
Cummings, J. and Foreman, M., The tree property. Advances in Mathematics, vol. 133 (1998), no. 1, pp. 1–32.CrossRefGoogle Scholar
Dobrinen, N. and Friedman, S.-D., The consistency strength of the tree property at the double successor of a measurable cardinal. Fundamenta Mathematicae, vol. 208 (2010), pp. 123–153.Google Scholar
Eisworth, T., Successors of singular cardinals, Handbook of Set Theory (Foreman, M. and Kanamori, A., editors), Springer, Netherlands, 2010, pp. 1229–1350.Google Scholar
Fontanella, L. and Magidor, M., Reflection of stationary sets and the tree property at the successor of a singular cardinal, this Journal, vol. 82 (2017), no. 1, pp. 272–291.Google Scholar
Foreman, M., Large cardinals and strong model theoretic transfer properties. Transactions of the American Mathematical Society, vol. 272 (1982), no. 2, pp. 427–463.Google Scholar
Foreman, M. and Magidor, M., Large cardinals and definable counterexamples to the continuum hypothesis. Annals of Pure and Applied Logic, vol. 76 (1995), no. 1, pp. 47–97.Google Scholar
Friedman, S.-D. and Halilovic, A., The tree property at ${\aleph _{\omega + 2}}$, this Journal, vol. 76 (2011), no. 2, pp. 477–490.Google Scholar
Friedman, S.-D., Honzik, R., and Stejskalová, Š., The tree property at ${\aleph _{\omega + 2}}$ with a finite gap, 2016. Preprint on webpage at http://logika.ff.cuni.cz/radek/papers/F-H-S_TP-aleph_omega-revised-2017-09-28.pdf.Google Scholar
Gitik, M., Extender based forcings, fresh sets and Aronszajn trees, Infinity, Computability and Metamathematics (Geschke, S., Koepke, P., and Schlicht, P., editors), Tributes, vol. 23, College Publications, London, 2014, pp. 183–203.Google Scholar
Gitik, M., Prikry-type forcings, Handbook of Set Theory (Foreman, M. and Kanamori, A., editors), Springer, Netherlands, 2010, pp. 1351–1447.Google Scholar
Gitik, M. and Krueger, J., Approachability at the second successor of a singular cardinal, this Journal, vol. 74 (2009), no. 4, pp. 1211–1224.Google Scholar
Gitik, M. and Sharon, A., On SCH and the approachability property. Proceedings of the American Mathematical Society, vol. 136 (2008), no. 1, pp. 311–320.Google Scholar
Harrington, L. and Shelah, S., Some exact equiconsistency results in set theory. Notre Dame Journal of Formal Logic, vol. 26 (1985), no. 2, pp. 178–188.CrossRefGoogle Scholar
Jensen, R. B., The fine structure of the constructible hierarchy. Annals of Mathematical Logic, 4 (1972), pp. 229–308; erratum, ibid. 4 (1972), 443. With a section by Jack Silver.Google Scholar
Kunen, K., Saturated ideals, this Journal, vol. 43 (1978), no. 1, pp. 65–76.Google Scholar
Magidor, M. and Shelah, S., The tree property at successors of singular cardinals. Archive for Mathematical Logic, vol. 35 (1996), no. 5–6, pp. 385–404.Google Scholar
Mitchell, W. J., Aronszajn trees and the independence of the transfer property. Annals of Pure and Applied Logic, vol. 5 (1972/73), pp. 21–46.Google Scholar
Rinot, A., A relative of the approachability ideal, diamond and nonsaturation, this Journal, vol. 75 (2010), no. 3, pp. 1035–1065.Google Scholar
Rinot, A., Higher Souslin trees and the GCH, revisited. Advances in Mathematics, vol. 311 (2017), no. C, 510–531.CrossRefGoogle Scholar
Shelah, S., On successors of singular cardinals, Logic Colloquium ’78 (Mons, 1978) (Boffa, M., van Dalen, D., and Mcaloon, K., editors), Studies in Logic and the Foundations of Mathematics, vol. 97, North-Holland, Amsterdam-New York, 1979, pp. 357–380.Google Scholar
Shelah, S., Appendix: on stationary sets (in “Classification of nonelementary classes. II. Abstract elementary classes”), Classification Theory (Baldwin, J., editor), Lecture Notes in Mathematics, vol. 1292, Springer, Berlin, 1987, pp. 483–495.Google Scholar
Shelah, S., Reflecting stationary sets and successors of singular cardinals. Archive for Mathematical Logic, vol. 31 (1991), pp. 25–53.Google Scholar
Shelah, S., Cardinal Arithmetic, Oxford Logic Guides, vol. 29, Oxford University Press, New York, 1994.CrossRefGoogle Scholar
Silver, J., The independence of Kurepa’s conjecture and two-cardinal conjectures in model theory, Axiomatic Set Theory (Scott, D. S., editor), Proceedings of Symposia in Pure Mathematics, vol. XIII, Part 1, American Mathematical Society, Providence, RI, 1971, pp. 383–390.CrossRefGoogle Scholar
Sinapova, D. and Unger, S., The tree property at ${\aleph _{{\omega ^2} + 1}}$ and ${\aleph _{{\omega ^2} + 2}}$, 2016. Preprint on webpage at http://homepages.math.uic.edu/∼sinapova/.Google Scholar
Unger, S., Aronszajn trees and the successors of a singular cardinal. Archive for Mathematical Logic, vol. 52 (2013), pp. 483–496.Google Scholar