We contribute to the study of generalizations of the Perfect Set Property and the Baire Property to subsets of spaces of higher cardinalities, like the power set ${\mathcal {P}}({\lambda })$ of a singular cardinal $\lambda $ of countable cofinality or products $\prod _{i<\omega }\lambda _i$ for a strictly increasing sequence 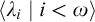$\langle {\lambda _i}~\vert ~{i<\omega }\rangle $ of cardinals. We consider the question under which large cardinal hypothesis classes of definable subsets of these spaces possess such regularity properties, focusing on rank-into-rank axioms and classes of sets definable by 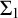$\Sigma _1$-formulas with parameters from various collections of sets. We prove that 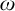$\omega $-many measurable cardinals, while sufficient to prove the perfect set property of all 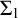$\Sigma _1$-definable sets with parameters in 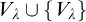$V_\lambda \cup \{V_\lambda \}$, are not enough to prove it if there is a cofinal sequence in 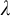$\lambda $ in the parameters. For this conclusion, the existence of an I2-embedding is enough, but there are parameters in 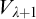$V_{\lambda +1}$ for which I2 is still not enough. The situation is similar for the Baire property: under I2 all sets that are 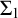$\Sigma _1$-definable using elements of 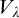$V_\lambda $ and a cofinal sequence as parameters have the Baire property, but I2 is not enough for some parameter in 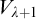$V_{\lambda +1}$. Finally, the existence of an I0-embedding implies that all sets that are $\Sigma ^1_n$-definable with parameters in 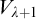$V_{\lambda +1}$ have the Baire property.