Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Saracino, Dan
and
Wood, Carol
1982.
QE nil-2 groups of exponent 4.
Journal of Algebra,
Vol. 76,
Issue. 2,
p.
337.
McNulty, George F.
1982.
Ordered Sets.
p.
299.
Schmerl, James H.
1983.
ℵ₀-categorical distributive lattices of finite breadth.
Proceedings of the American Mathematical Society,
Vol. 87,
Issue. 4,
p.
707.
Schmerl, James H.
1984.
Orders: Description and Roles - In Set Theory, Lattices, Ordered Groups, Topology, Theory of Models and Relations, Combinatorics, Effectiveness, Social Sciences, Proceedings of the Conference on Ordered Sets and their Application Château dc la Tourcttc; Ordres: Description et Rôles - En Théorie des Treillis, des Groupes Ordonnés; en Topologie, Théorie des Modèles et des Relations, Cornbinatoire, Effectivité, Sciences Sociales, Actes de la Conférence sur ies Ensembles Ordonnés et leur Applications Château de la Tourette.
Vol. 99,
Issue. ,
p.
269.
Schmerl, James H.
1985.
Graphs and Order.
p.
467.
Cherlin, Gregory
1986.
Logic, Methodology and Philosophy of Science VII, Proceedings of the Seventh International Congress of Logic, Methodology and Philosophy of Science.
Vol. 114,
Issue. ,
p.
99.
Puninskij, G. E.
1989.
The Model Completion of the Theory of All Partially Ordered Sets.
Mathematical Logic Quarterly,
Vol. 35,
Issue. 6,
p.
481.
Seese, D.
1991.
The structure of the models of decidable monadic theories of graphs.
Annals of Pure and Applied Logic,
Vol. 53,
Issue. 2,
p.
169.
Kalantari, I.
1998.
Handbook of Recursive Mathematics - Volume 1: Recursive Model Theory.
Vol. 138,
Issue. ,
p.
515.
Stukachev, Alexey
2005.
New Computational Paradigms.
Vol. 3526,
Issue. ,
p.
470.
Stukachev, Alexey
2013.
Effective Mathematics of the Uncountable.
p.
164.
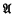 there is a linear order of A such that (
there is a linear order of A such that (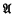 , <) is ℵ0-categorical.
, <) is ℵ0-categorical.