Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-26T08:20:13.908Z Has data issue: false hasContentIssue false
  • English
  • Français

COMPACT CARDINALS AND EIGHT VALUES IN CICHOŃ’S DIAGRAM

Published online by Cambridge University Press:  01 August 2018

JAKOB KELLNER ,
ANDA RAMONA TĂNASIE  and
FABIO ELIO TONTI
JAKOB KELLNER
Affiliation:
INSTITUTE OF DISCRETE MATHEMATICS AND GEOMETRY TECHNISCHE UNIVERSITÄT WIEN (TU WIEN) WIEN, AUSTRIAE-mail:[email protected]
ANDA RAMONA TĂNASIE
Affiliation:
INSTITUTE OF DISCRETE MATHEMATICS AND GEOMETRY TECHNISCHE UNIVERSITÄT WIEN (TU WIEN) WIEN, AUSTRIAE-mail:[email protected]
FABIO ELIO TONTI
Affiliation:
INSTITUTE OF DISCRETE MATHEMATICS AND GEOMETRY TECHNISCHE UNIVERSITÄT WIEN (TU WIEN) WIEN, AUSTRIAE-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Assuming three strongly compact cardinals, it is consistent that

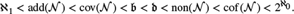$${\aleph _1} < add\left( {\cal N} \right) < cov\left( {\cal N} \right) < \mathfrakb < \mathfrakd < non\left( {\cal N} \right) < cof\left( {\cal N} \right) < {2^{{\aleph _0}}}.$$

Under the same assumption, it is consistent that

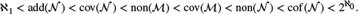$${\aleph _1} < add\left( {\cal N} \right) < cov\left( {\cal N} \right) < non\left( {\cal M} \right) < cov\left( {\cal M} \right) < non\left( {\cal N} \right) < cof\left( {\cal N} \right) < {2^{{\aleph _0}}}.$$

Keywords

03E17set theory of the realsCichoń’s diagramlarge continuumlarge cardinals
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 2 , June 2018 , pp. 790 - 803
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bartoszyński, T., Combinatorial aspects of measure and category.Fundamenta Mathematicae, vol. 127 (1987), no. 3, pp. 225–239.CrossRefGoogle Scholar
Bartoszyński, T. and Judah, H., Set Theory: On the structure of the real line, A K Peters, Ltd., Wellesley, MA, 1995.Google Scholar
Brendle, J., Larger cardinals in Cichoń’s diagram, this Journal, vol. 56 (1991), no. 3, pp. 795–810.Google Scholar
Fischer, A., Goldstern, M., Kellner, J., and Shelah, S., Creature forcing and five cardinal characteristics of the continuum. Archive for Mathematical Logic, vol. 56 (2017), pp. 1045–1103.CrossRefGoogle Scholar
Goldstern, M., Kellner, J., and Shelah, S., Cichoń’s maximum, preprint, 2017, arXiv: 1708.03691.Google Scholar
Goldstern, M., Mejía, D. A., and Shelah, S, The left side of Cichoń’s diagram. Proceedings of the American Mathematical Society, vol. 144 (2016), no. 9, pp. 4025–4042.CrossRefGoogle Scholar
Judah, H. and Shelah, S., The Kunen-Miller chart (Lebesgue measure, the Baire property, Laver reals and preservation theorems for forcing), this Journal, vol. 55 (1990), no. 3, pp. 909–927.Google Scholar
Kamburelis, A., Iterations of Boolean algebras with measure. Archive for Mathematical Logic, vol. 29 (1989), no. 1, pp. 21–28.CrossRefGoogle Scholar
Keisler, H. J. and Tarski, A., From accessible to inaccessible cardinals. Results holding for all accessible cardinal numbers and the problem of their extension to inaccessible ones. Fundamenta Mathematicae, vol. 53 (1963/1964), pp. 225–308.Google Scholar
Mansfield, R., The theory of Boolean ultrapowers. Annals of Mathematical Logic, vol. 2 (1970/71), no. 3, pp. 297–323.CrossRefGoogle Scholar
Mejía, D. A., Matrix iterations and Cichon’s diagram. Archive for Mathematical Logic, vol. 52 (2013), no. (3-4), pp. 261–278.CrossRefGoogle Scholar
Miller, A. W., A characterization of the least cardinal for which the Baire category theorem fails. Proceedings of the American Mathematical Society, vol. 86 (1982), no. 3, pp. 498–502.CrossRefGoogle Scholar
Malliaris, M. and Shelah, S., Existence of optimal ultrafilters and the fundamental complexity of simple theories. Advances in Mathematics, vol. 290 (2016), pp. 614–681.CrossRefGoogle Scholar
Raghavan, D. and Shelah, S., Boolean ultrapowers and iterated forcing, in preparation.Google Scholar