Published online by Cambridge University Press: 12 March 2014
In March 1973, W. Veldman [1] discovered that, by a slight modification of a Kripke-model, it was possible to give an intuitionistic proof of the completeness-theorem for the intuitionistic predicate calculus (IPC) with respect to modified Kripke models. The modification was the following: Let f represent absurdity, then we allow the possibility that 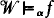 and we agree that, for all sentences ϕ,
and we agree that, for all sentences ϕ,  , if
, if 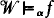 . Just one modified Kripke model
. Just one modified Kripke model 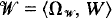 is constructed such that validity in
is constructed such that validity in 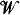 implies derivability in IPC. While usually one thinks of
implies derivability in IPC. While usually one thinks of 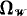 as some subset of ⋃
n
Nat
n and of
as some subset of ⋃
n
Nat
n and of  as the discrete natural ordering in ⋃
n
Nat
n, in Veldman's model
as the discrete natural ordering in ⋃
n
Nat
n, in Veldman's model 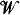 ,
, 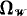 is a spread and
is a spread and  , where Γα and Γβ are sets of sentences associated with α, resp. β, is a nondiscrete ordering.
, where Γα and Γβ are sets of sentences associated with α, resp. β, is a nondiscrete ordering.
In the completeness-proofs, both for Beth and for Kripke models that we present here, we consider only models over ⋃n Nat n, with the natural discrete ordering and we need validity in all models, not just in one, to get derivability in IPC. Also we have to modify the definition of a model in a somewhat different way than Veldman did. We agree that if ∨s[M⊨s f], then M⊨sϕ for each s ∈ ⋃n Nat n and for each sentence ϕ.
One can view a single model 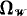 of the type constructed in [1] as the result of throwing together all the models of (the type constructed in) this paper into one big model, which has the somewhat strange properties mentioned above.
of the type constructed in [1] as the result of throwing together all the models of (the type constructed in) this paper into one big model, which has the somewhat strange properties mentioned above.