Published online by Cambridge University Press: 12 March 2014
An operator Γ mapping P(ω) to itself is inductive if Γ(A) ⊇ A for all A. For such an inductive operator Γ we define {Γα : α ∈ ORD} by letting Γ = ⌀, Γα + 1 = Γ(Γα ) for all α, and Γβ = ⋃{Γα : α < β} for limit ordinals β. The closure ordinal ∣Γ∣ of Γ is the least ordinal α such that Γα+1 = Γα and the closure 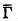 is Γ∣Γ∣.
is Γ∣Γ∣.
Let  be a class of operators over the natural numbers. The closure ordinal ∣
be a class of operators over the natural numbers. The closure ordinal ∣ ∣ of
∣ of  is the supremum of {∣Γ∣: Γ is an inductive operator and
is the supremum of {∣Γ∣: Γ is an inductive operator and 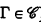 }. The closure algebra
}. The closure algebra 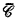 generated by
generated by  is {A ⊆ ω: A is 1-1 reducible to
is {A ⊆ ω: A is 1-1 reducible to 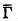 for some inductive operator
for some inductive operator 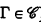 }. The inductive algebra
}. The inductive algebra  generated by
generated by  is {Γα : Γ is an inductive operator in
is {Γα : Γ is an inductive operator in  and α < ∣Γ∣}.
and α < ∣Γ∣}.
For A ∈ P(ω), 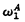 is the supremum of the ordinals of well-orderings recursive in A. For
is the supremum of the ordinals of well-orderings recursive in A. For 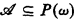 , let
, let 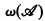 be the supremum of
be the supremum of  . For example, it is well known that ω(
. For example, it is well known that ω( ) = ω(∆1 0 = ω 1, ω(Π1 1) = ω 1 and ω(Δn 1) = δn 1 for n > 1 (the latter by definition).
) = ω(∆1 0 = ω 1, ω(Π1 1) = ω 1 and ω(Δn 1) = δn 1 for n > 1 (the latter by definition).