Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chenevier, P
and
Dolique, J M
1974.
The sheath on a plane obstacle parallel to a hypersonic collisionless plasma flow.
Plasma Physics,
Vol. 16,
Issue. 10,
p.
983.
Könemann, Bernd
1978.
The collisionless flow of unmagnetized plasmas around bodies.
Journal of Plasma Physics,
Vol. 20,
Issue. 1,
p.
17.
Šesták, B.
1980.
Potential of the test particle in the magnetic field I.
Czechoslovak Journal of Physics,
Vol. 30,
Issue. 2,
p.
134.
Weisheit, Jon C.
1989.
Advances in Atomic and Molecular Physics Volume 25.
Vol. 25,
Issue. ,
p.
101.
Peter, Thomas
1990.
Linearized potential of an ion moving through plasma.
Journal of Plasma Physics,
Vol. 44,
Issue. 2,
p.
269.
D’Avanzo, J.
Lontano, M.
and
Bortignon, P. F.
1993.
Fast-ion interaction in dense plasmas with two-ion correlation effects.
Physical Review E,
Vol. 47,
Issue. 5,
p.
3574.
Shukla, P. K.
and
Salimullah, M.
1996.
The potential around a test charge in magnetized dusty plasmas.
Physics of Plasmas,
Vol. 3,
Issue. 10,
p.
3858.
Shukla, P. K.
and
Rao, N. N.
1996.
Coulomb crystallization in colloidal plasmas with streaming ions and dust grains.
Physics of Plasmas,
Vol. 3,
Issue. 5,
p.
1770.
Shukla, P K
and
Melandsø, F
1997.
Test charge potential in the presence of electron-acoustic waves in plasmas.
Physica Scripta,
Vol. 55,
Issue. 2,
p.
239.
Melandsø, Frank
1997.
Heating and phase transitions of dust-plasma crystals in a flowing plasma.
Physical Review E,
Vol. 55,
Issue. 6,
p.
7495.
Salimullah, M.
and
Shukla, P. K.
1998.
Wake potentials in nonuniform dusty magnetoplasmas.
Physics of Plasmas,
Vol. 5,
Issue. 12,
p.
4205.
Nasim, M. H.
Mirza, Arshad M.
Qaisar, M. S.
Murtaza, G.
and
Shukla, P. K.
1998.
Energy loss of charged projectiles in dusty plasmas.
Physics of Plasmas,
Vol. 5,
Issue. 10,
p.
3581.
Frolov, A A
and
Gorbunov, L M
1999.
Current Induced by a Fast Charged Test Particle Moving into Rarefied Plasmas.
Physica Scripta,
Vol. 60,
Issue. 3,
p.
253.
Nasim, M H
Mirza, Arshad M
Murtaza, G
and
Shukla, P K
1999.
Energy Loss of a Test Charge in Dusty Plasmas: Collective and Individual Particle Contributions.
Physica Scripta,
Vol. 59,
Issue. 5,
p.
379.
Nasim, M. H.
Shukla, P. K.
and
Murtaza, G.
1999.
Effect of dust charge fluctuations on energy loss of a test dust charged particulate in a dusty plasma.
Physics of Plasmas,
Vol. 6,
Issue. 5,
p.
1409.
Konopka, U.
Morfill, G. E.
and
Ratke, L.
2000.
Measurement of the Interaction Potential of Microspheres in the Sheath of a rf Discharge.
Physical Review Letters,
Vol. 84,
Issue. 5,
p.
891.
Lemons, D. S.
Murillo, M. S.
Daughton, W.
and
Winske, D.
2000.
Two-dimensional wake potentials in sub- and supersonic dusty plasmas.
Physics of Plasmas,
Vol. 7,
Issue. 6,
p.
2306.
Nasim, M. H.
Qaisar, M. S.
Mirza, Arshad M.
Murtaza, G.
and
Shukla, P. K.
2000.
Energy loss of a test charge in partially ionized dusty plasmas.
Physics of Plasmas,
Vol. 7,
Issue. 2,
p.
762.
Lampe, Martin
Joyce, Glenn
Ganguli, Gurudas
and
Gavrishchaka, Valeriy
2000.
Interactions between dust grains in a dusty plasma.
Physics of Plasmas,
Vol. 7,
Issue. 10,
p.
3851.
Hammerberg, J.E.
Lemons, D.S.
Murillo, M.S.
and
Winske, D.
2001.
Molecular dynamics simulations of plasma crystal formation including wake effects.
IEEE Transactions on Plasma Science,
Vol. 29,
Issue. 2,
p.
247.
 along the cone surface. Outside the cone, the potential decays exponentially. A charge moving slower than the ion acoustic velocity also creates a cone, with potential decay as γ−3 outside the cone, potential wells decaying as γ−1 inside the cone, and potential wells falling off as
along the cone surface. Outside the cone, the potential decays exponentially. A charge moving slower than the ion acoustic velocity also creates a cone, with potential decay as γ−3 outside the cone, potential wells decaying as γ−1 inside the cone, and potential wells falling off as 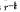 along the cone surface. In both cases a radial logarithmic singularity exists along the trailing axis. Using a mono-energetic ion distribution, the singularity is removed and an ion thermal Cerenkov cone appears. For a monoenergetic electron plasma, assuming immobile ions, a test charge moving faster than the electron thermal velocity excites a thermal Cerenkov cone. Outside the cone, the far-field potential falls off in quadrupole form as γ−3. Inside the cone, potential wells decay as γ−1.
along the cone surface. In both cases a radial logarithmic singularity exists along the trailing axis. Using a mono-energetic ion distribution, the singularity is removed and an ion thermal Cerenkov cone appears. For a monoenergetic electron plasma, assuming immobile ions, a test charge moving faster than the electron thermal velocity excites a thermal Cerenkov cone. Outside the cone, the far-field potential falls off in quadrupole form as γ−3. Inside the cone, potential wells decay as γ−1.

