2 Gyrokinetic theory

Following Helander (Reference Helander2014), Helander & Connor (Reference Helander and Connor2016), Mishchenko et al. (Reference Mishchenko, Plunk and Helander2018a ) and Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ), we will use gyrokinetic theory to analyse the stability of electron–positron–ion plasmas in this work, retaining the possibility of arbitrary degree of deviation from quasineutrality.

2.1 Dispersion relation

It is convenient to write the gyrokinetic distribution function in the form

(2.1a,b ) $$\begin{eqnarray}f_{a}=\,f_{a0}\left(1-\frac{e_{a}\unicode[STIX]{x1D711}}{T_{a}}\right)+g_{a}=\,f_{a0}+f_{a1},\quad f_{a1}=-\frac{e_{a}\unicode[STIX]{x1D711}}{T_{a}}f_{a0}+g_{a}.\end{eqnarray}$$

Here, $f_{a0}$ is a Maxwellian, $a$ is the species index with $a=e$ corresponding to electrons, $a=p$ to positrons and $a=i$ to the heavy ion species. We take care here to point out that in this work we will concern ourselves with both positively charged ions and negatively charged antiprotons, both of which will be denoted by the same subscript, the charge on species $i$ will be assumed positive unless explicitly stated otherwise; $\unicode[STIX]{x1D746}=\boldsymbol{b}\times \boldsymbol{v}/\unicode[STIX]{x1D6FA}_{a}$ is the species gyroradius and $\unicode[STIX]{x1D6FA}_{a}$ the species cyclotron frequency. The remainder of the notation is standard.

The potential function for this system is given by

(2.2) $$\begin{eqnarray}\unicode[STIX]{x1D719}=\unicode[STIX]{x1D719}_{0}+\unicode[STIX]{x1D712},\end{eqnarray}$$

where $\unicode[STIX]{x1D719}_{0}$ is the background electrostatic potential due to the non-zero equilibrium electric field in the plasma, $\unicode[STIX]{x1D712}=\unicode[STIX]{x1D711}-v_{\Vert }A_{\Vert }$ is the usual gyrokinetic potential with $\unicode[STIX]{x1D711}$ the perturbed electrostatic potential and $A_{\Vert }$ the perturbed parallel magnetic potential.

In this notation, the linearised gyrokinetic equation is

(2.3) $$\begin{eqnarray}(\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-\unicode[STIX]{x1D714}_{da}-k_{\Vert }v_{\Vert })g_{a}=\frac{e_{a}}{T_{a}}(\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-\unicode[STIX]{x1D714}_{\star a}^{T})(\unicode[STIX]{x1D711}-v_{\Vert }A_{\Vert })J_{0}\left(\frac{k_{\bot }v_{\bot }}{\unicode[STIX]{x1D6FA}_{a}}\right)f_{a0},\end{eqnarray}$$

with $J_{0}$ the Bessel function, $k_{\bot }$ the perpendicular wave number, $k_{\Vert }$ the parallel wavenumber. Other notation employed here is

(2.4a-d ) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D714}_{\star a}^{T}=\unicode[STIX]{x1D714}_{\star a}\left[1+\unicode[STIX]{x1D702}_{a}\left(\frac{v^{2}}{v_{\text{th}a}^{2}}-\frac{3}{2}\right)\right],\quad v=\sqrt{v_{\Vert }^{2}+v_{\bot }^{2}},\quad k_{\bot }=\sqrt{k_{x}^{2}+k_{y}^{2}},\quad k_{\unicode[STIX]{x1D6FC}}=\frac{k_{y}}{B} & & \displaystyle \nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$

(2.5a-d ) $$\begin{eqnarray}\unicode[STIX]{x1D714}_{\star a}=\frac{k_{\unicode[STIX]{x1D6FC}}T_{a}}{e_{a}}\frac{\text{d}\ln n_{a}}{\text{d}x},\quad \unicode[STIX]{x1D702}_{a}=\frac{\text{d}\ln T_{a}}{\text{d}\ln n_{a}},\quad v_{\text{th}a}=\sqrt{\frac{2T_{a}}{m_{a}}},\quad \unicode[STIX]{x1D714}_{da}=\boldsymbol{k}_{\bot }\boldsymbol{\cdot }\boldsymbol{v}_{da},\end{eqnarray}$$

with

(2.6) $$\begin{eqnarray}\boldsymbol{v}_{da}=\frac{v_{\bot }^{2}}{2\unicode[STIX]{x1D6FA}_{a}}\boldsymbol{b}\times \unicode[STIX]{x1D735}\ln B+\frac{v_{\Vert }^{2}}{\unicode[STIX]{x1D6FA}_{a}}\boldsymbol{b}\times (\boldsymbol{b}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\boldsymbol{b}).\end{eqnarray}$$

Here we will choose the sign convention such that $\unicode[STIX]{x1D714}_{\star i}\leqslant 0,\unicode[STIX]{x1D714}_{\star p}\leqslant 0,$ and $\unicode[STIX]{x1D714}_{\star e}\geqslant 0.$ In our slab geometry $x$ denotes the direction of any non-uniformity in the plasma profiles. For simplicity we will assume $k_{x}=0$ and $k_{\bot }=k_{y}$ throughout the paper.

The influence of the background electric field is only felt through the term

(2.7a,b ) $$\begin{eqnarray}k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }=\boldsymbol{k}_{\bot }\boldsymbol{\cdot }\boldsymbol{v}_{E0},\quad \boldsymbol{v}_{E0}=\frac{1}{B}\boldsymbol{b}\times \unicode[STIX]{x1D735}\unicode[STIX]{x1D719}_{0},\end{eqnarray}$$

a quantity which is locally constant. We will discuss the consequences of this local approximation at the end of the section.

In slab geometry $\unicode[STIX]{x1D714}_{da}=0$ and hence our equation may be trivially solved to give

(2.8) $$\begin{eqnarray}g_{a}=\frac{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-\unicode[STIX]{x1D714}_{\star a}^{T}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-k_{\Vert }v_{\Vert }}\frac{e_{a}\,f_{a0}}{T_{a}}J_{0}(\unicode[STIX]{x1D711}-v_{\Vert }A_{\Vert })f_{a0}.\end{eqnarray}$$

This equation is supplemented by Poisson’s equation and the parallel Ampere’s law for the perturbation. These equations read

(2.9a,b ) $$\begin{eqnarray}\left(\mathop{\sum }_{a}\frac{n_{a}e_{a}^{2}}{T_{a}}+\unicode[STIX]{x1D716}_{0}k_{\bot }^{2}\right)\unicode[STIX]{x1D711}=\mathop{\sum }_{a}e_{a}\int g_{a}J_{0}\,\text{d}^{3}v,\quad A_{\Vert }=\frac{\unicode[STIX]{x1D707}_{0}}{k_{\bot }^{2}}\mathop{\sum }_{a}q_{a}\int v_{\Vert }g_{a}J_{0}\,\text{d}^{3}v.\end{eqnarray}$$

For the electromagnetic dispersion relation we will find it convenient to define the function

(2.10) $$\begin{eqnarray}W_{na}=-\frac{1}{n_{a}v_{\text{th}a}^{n}}\int \frac{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-\unicode[STIX]{x1D714}_{\star a}^{T}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-k_{\Vert }v_{\Vert }}J_{0}^{2}\,f_{a0}v_{\Vert }^{n}\,\text{d}^{3}v,\end{eqnarray}$$

which may be evaluated to obtain

(2.11) $$\begin{eqnarray}\displaystyle W_{na}=\unicode[STIX]{x1D701}_{a}\left\{\left(1-\frac{\unicode[STIX]{x1D714}_{\star a}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }}\right)Z_{na}\unicode[STIX]{x1D6E4}_{0a}+\frac{\unicode[STIX]{x1D714}_{\star a}\unicode[STIX]{x1D702}_{a}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }}\left[\frac{3}{2}Z_{na}\unicode[STIX]{x1D6E4}_{0a}-Z_{na}\unicode[STIX]{x1D6E4}_{\star a}-Z_{n+2,a}\unicode[STIX]{x1D6E4}_{0a}\right]\right\}. & & \displaystyle \nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$

Here, the following notation has been used

(2.12a-d ) $$\begin{eqnarray}\frac{1}{\unicode[STIX]{x1D706}_{Da}^{2}}=\frac{q_{a}^{2}n_{a}}{\unicode[STIX]{x1D716}_{0}T_{a}},\quad \frac{1}{\unicode[STIX]{x1D706}_{D}^{2}}=\mathop{\sum }_{a}\frac{1}{\unicode[STIX]{x1D706}_{Da}^{2}},\quad b_{a}=k_{\bot }^{2}\unicode[STIX]{x1D70C}_{a}^{2},\quad \unicode[STIX]{x1D70C}_{a}=\frac{\sqrt{m_{a}T_{a}}}{|e_{a}|B},\end{eqnarray}$$

(2.13a-c ) $$\begin{eqnarray}\unicode[STIX]{x1D6E4}_{\star a}=\unicode[STIX]{x1D6E4}_{0a}-b_{a}[\unicode[STIX]{x1D6E4}_{0a}-\unicode[STIX]{x1D6E4}_{1a}],\quad \unicode[STIX]{x1D6E4}_{0a}=I_{0}(b_{a})\text{e}^{-b_{a}},\quad \unicode[STIX]{x1D6E4}_{1a}=I_{1}(b_{a})\text{e}^{-b_{a}},\end{eqnarray}$$

(2.14a,b ) $$\begin{eqnarray}Z_{na}=\frac{1}{\sqrt{\unicode[STIX]{x03C0}}}\int _{-\infty }^{\infty }\frac{x^{n}\text{e}^{-x^{2}}}{x-\unicode[STIX]{x1D701}_{a}},\quad \unicode[STIX]{x1D701}_{a}=\frac{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }}{k_{\Vert }v_{\text{th}a}}.\end{eqnarray}$$

We can substitute our equation for the gyrokinetic distribution function into each of the field equations and use the notation given above to obtain

(2.15) $$\begin{eqnarray}\displaystyle & \displaystyle (1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2})\unicode[STIX]{x1D711}+\mathop{\sum }_{a}\frac{\unicode[STIX]{x1D706}_{D}^{2}}{\unicode[STIX]{x1D706}_{Da}^{2}}(W_{0a}\unicode[STIX]{x1D711}-W_{1a}A_{\Vert }v_{\text{th}a})=0, & \displaystyle\end{eqnarray}$$

(2.16) $$\begin{eqnarray}\displaystyle & \displaystyle A_{\Vert }+\frac{1}{c^{2}}\mathop{\sum }_{a}\frac{v_{\text{th}a}}{k_{\bot }^{2}\unicode[STIX]{x1D706}_{Da}^{2}}(W_{1a}\unicode[STIX]{x1D711}-W_{2a}A_{\Vert }v_{\text{th}a})=0. & \displaystyle\end{eqnarray}$$

This gives rise to the dispersion relation

(2.17) $$\begin{eqnarray}\displaystyle & & \displaystyle \left(1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\mathop{\sum }_{a}\frac{\unicode[STIX]{x1D706}_{D}^{2}}{\unicode[STIX]{x1D706}_{Da}^{2}}W_{0a}\right)\left(1-2\mathop{\sum }_{a}\frac{\unicode[STIX]{x1D6FD}_{a}}{k_{\bot }^{2}\unicode[STIX]{x1D70C}_{a}^{2}}W_{2a}\right)\nonumber\\ \displaystyle & & \displaystyle \quad +\,2\mathop{\sum }_{a}\frac{\unicode[STIX]{x1D706}_{D}^{2}}{\unicode[STIX]{x1D706}_{Da}^{2}}W_{1a}v_{\text{th}a}\mathop{\sum }_{a}\frac{\unicode[STIX]{x1D6FD}_{a}}{k_{\bot }^{2}\unicode[STIX]{x1D70C}_{a}^{2}}\frac{W_{1a}}{v_{\text{th}a}}=0.\end{eqnarray}$$

Here $\unicode[STIX]{x1D6FD}_{a}=\unicode[STIX]{x1D707}_{0}n_{a}T_{a}/B^{2},$ the usual plasma beta. We will restrict our attention to the electrostatic limit, corresponding to $\unicode[STIX]{x1D6FD}_{a}=0$ . This dispersion relation clearly reduces to the result of Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ) in the limit of quasineutrality.

2.2 Quasilinear particle fluxes

Following Helander & Zocco (Reference Helander and Zocco2018), we define the cross-field particle flux of species $a$ to be given by

(2.18) $$\begin{eqnarray}\unicode[STIX]{x1D6E4}_{a}=\text{Re}\left\langle \int (\boldsymbol{v}_{E}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\unicode[STIX]{x1D713})f_{a}\,\text{d}^{3}v\right\rangle ,\end{eqnarray}$$

where the angular brackets denote the flux surface average

(2.19) $$\begin{eqnarray}\langle \cdots \rangle =\lim _{L\rightarrow \infty }\int _{-L}^{L}(\cdots )\frac{\text{d}l}{B(l)}/\int _{-L}^{L}\frac{\text{d}l}{B(l)},\end{eqnarray}$$

which we remark has no effect in the straight field line limit considered here.

The non-neutral drift waves reported in this paper can also lead to cross-field particle diffusion. The particle flux due to drift-wave instabilities in standard electron–ion plasmas has been studied in the aforementioned paper by Helander & Zocco (Reference Helander and Zocco2018). Here, we are able to simplify certain aspects of the calculations by the restrictions placed on the geometry whilst introducing further complications by the non-neutrality.

We have already found that the equation for the perturbed part of the distribution function is given in the local limit by (2.3). Hence, the quasilinear particle flux of species $a$ is given by

(2.20) $$\begin{eqnarray}\unicode[STIX]{x1D6E4}_{a}=\text{Re}\left\langle \int (\boldsymbol{v}_{E}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\unicode[STIX]{x1D713})f_{a}\,\text{d}^{3}v\right\rangle =\text{Im}\frac{1}{B}\left\langle \int k_{y}\unicode[STIX]{x1D711}^{\star }g_{a}J_{0}\,\text{d}^{3}v\right\rangle ,\end{eqnarray}$$

which yields

(2.21) $$\begin{eqnarray}\unicode[STIX]{x1D6E4}_{a}=\frac{k_{y}}{B}\text{Im}\left\langle \int \frac{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-\unicode[STIX]{x1D714}_{\star a}^{T}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }-k_{\Vert }v_{\Vert }}\frac{e_{a}\,f_{a0}}{T_{a}}J_{0}^{2}|\unicode[STIX]{x1D711}|^{2}f_{a0}\,\text{d}^{3}v\right\rangle =\frac{k_{y}}{B}\text{Im}\left\langle \frac{n_{a}e_{a}}{T_{a}}W_{0a}|\unicode[STIX]{x1D711}|^{2}\right\rangle .\end{eqnarray}$$

Hence, one obtains

(2.22) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6E4}_{a} & = & \displaystyle \frac{k_{y}}{B}\text{Im}\left\langle \frac{n_{a}e_{a}}{T_{a}}|\unicode[STIX]{x1D711}|^{2}\unicode[STIX]{x1D701}_{a}\left\{\left(1-\frac{\unicode[STIX]{x1D714}_{\star a}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }}\right)Z_{0a}\unicode[STIX]{x1D6E4}_{0a}\right.\right.\nonumber\\ \displaystyle & & \displaystyle +\left.\left.\frac{\unicode[STIX]{x1D714}_{\star a}\unicode[STIX]{x1D702}_{a}}{\unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }}\left[\frac{3}{2}Z_{0a}\unicode[STIX]{x1D6E4}_{0a}-Z_{0a}\unicode[STIX]{x1D6E4}_{\star a}-Z_{2,a}\unicode[STIX]{x1D6E4}_{0a}\right]\right\}\right\rangle .\end{eqnarray}$$

We remark that it is of course very simple to extend the particle flux to the electromagnetic case, however the focus of this work is exclusively on $\unicode[STIX]{x1D6FD}_{a}=0$ plasmas and hence we shall not do so here.

It is well known that gyrokinetic transport is intrinsically ambipolar (Sugama et al. Reference Sugama, Okamoto, Horton and Wakatani1998) and it is easy to verify that this result also holds true in the non-neutral case. We calculate

(2.23) $$\begin{eqnarray}\mathop{\sum }_{a}e_{a}\unicode[STIX]{x1D6E4}_{a}=\unicode[STIX]{x1D716}_{0}k_{y}|\unicode[STIX]{x1D711}|^{2}\text{Im}\left(\mathop{\sum }_{a}\frac{W_{0a}}{\unicode[STIX]{x1D706}_{Da}^{2}}\right)=0,\end{eqnarray}$$

where the final equality follows immediately from the electrostatic limit of the dispersion relation (2.17).

In this work, we will numerically calculate the quantity

(2.24) $$\begin{eqnarray}\unicode[STIX]{x1D6EC}_{a}=\frac{e_{a}\unicode[STIX]{x1D6E4}_{a}}{\unicode[STIX]{x1D716}_{0}|\unicode[STIX]{x1D711}|^{2}}.\end{eqnarray}$$

Here, $|\unicode[STIX]{x1D711}|^{2}$ is a scaling factor associated with the saturation amplitude of the fluctuations, which does not need to be determined explicitly as we are primarily interested in the directions of the fluxes and any interesting behaviour exhibited. To this end, we remark that $\unicode[STIX]{x1D6EC}_{a}$ is simply the particle current up to an unknown positive constant. We are able to calculate the quantity $\unicode[STIX]{x1D6EC}_{a}$ for the entire parameter range but note here that we only expect these to make some physical sense in the parameter ranges where the growth rate $\unicode[STIX]{x1D6FE}>0$ i.e. where there is actually an instability present. It is worth remarking that usually one expects $|\unicode[STIX]{x1D711}|^{2}=0$ in the stable domain and hence the quasilinear fluxes $\unicode[STIX]{x1D6E4}_{a}=0$ in these domains. This rule of thumb is generally, but not always true and it may be the case that even in linearly stable domains, there is a particle transport driven by subcritical turbulence, i.e. the system is formally stable to small perturbations, but, given a large enough initial perturbation, it transitions to a turbulent state.

2.3 Physical assumptions

In the local limit considered here, we have relegated the effect of the background electric field into a Doppler shift of the complex frequency $\unicode[STIX]{x1D714}\rightarrow \unicode[STIX]{x1D714}-k_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D719}_{0}^{\prime }.$ This result has a simple physical interpretation, namely that in the frame rotating with the $\boldsymbol{E}\times \boldsymbol{B}$ velocity, the nascent electric field generated by the plasma is identically zero. Hence in this rotating frame we achieve precisely the result of Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ). We note that in our model, the Doppler shift is arbitrary. The reason for this is that $\unicode[STIX]{x1D719}_{0}$ does not appear explicitly in the zeroth-order Poisson equation for the length scales considered here: $\unicode[STIX]{x1D719}_{0}^{\prime \prime }/\unicode[STIX]{x1D719}_{0}\ll k_{\bot }^{2}$ . It may appear at first glance that this trivialises the dynamics of non-neutral plasmas insofar as one might expect the problem reduces exactly to Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ). This is not the case.

Despite the relatively straightforward, physically pleasing relationship between the dispersion relation for non-neutral and quasineutral plasmas in the local limit, there is more subtle difference at play. We recall that in Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ) it was necessary for there to be symmetry breaking due to either the temperature profiles or ion contamination for instabilities to be excited. In a non-quasineutral plasma, there is another degree of freedom in the system as it permissible to violate the quasineutrality condition. A key stability parameter for three component non-neutral plasmas was the species fraction

(2.25) $$\begin{eqnarray}\unicode[STIX]{x1D708}_{a}=\frac{n_{a}}{n_{e}},\end{eqnarray}$$

where we note that a quasineutral plasma must satisfy the quasineutrality constraint

(2.26) $$\begin{eqnarray}\mathop{\sum }_{a}\unicode[STIX]{x1D708}_{a}=2.\end{eqnarray}$$

For a non-neutral plasma we have no such constraint and $\sum _{a}\unicode[STIX]{x1D708}_{a}$ may be arbitrary. Indeed, it is now possible to break the symmetry of the density profiles of even a simple pair plasma. This leads to a diversification in the types of gyrokinetic modes which can arise in such plasmas. Indeed, it is precisely this symmetry breaking (allowing us to take in pair plasma $n_{e}\neq n_{p}$ for example) that gives rise to instabilities.

Here we consider a local theory including ambient electric field, always present in a non-neutral plasma, but neglect the shear of this field. This is an important approximation which will be relaxed in future work. Indeed, it must be relaxed for the diocotron instability (Davidson Reference Davidson1974). It is well known that the global diocotron mode, an analogue of the shearing Kelvin–Helmholtz instability, plays a pivotal role in the dynamics of non-neutral plasmas. We plan to address this more complex question with a global gyrokinetic code in the future. In this paper we will consider only the local limit and concentrate solely on drift mode dynamics.

It is also pertinent to comment on the use of Ampere’s law in our derivation above. One ought to question whether there is a need to include the displacement current on the basis that we have included Debye shielding in Poisson’s equation. Here, Debye shielding is important due to having a sufficiently small plasma $\unicode[STIX]{x1D6FD}_{a}$ (which here means that $\unicode[STIX]{x1D6FD}_{a}\lesssim v_{\text{th}a}^{2}/c^{2}$ ). This makes it necessary to include Debye shielding effects as $\unicode[STIX]{x1D706}_{Da}^{2}/\unicode[STIX]{x1D70C}_{a}^{2}=(1/2\unicode[STIX]{x1D6FD}_{a})(v_{\text{th}a}^{2}/c^{2})$ and hence we expect the Debye length to be comparable to the electron Larmor radius. However, as pointed out by Barnes, Abiuso & Dorland (Reference Barnes, Abiuso and Dorland2018) we note that even when $\unicode[STIX]{x1D706}_{Da}^{2}/\unicode[STIX]{x1D70C}_{a}^{2}\sim 1$ , the displacement current appearing in Ampere’s law is negligible in the gyrokinetic ordering compared to the plasma current. In this ordering the displacement current must only be retained when taking the divergence of Ampere’s law.

3 Gyrokinetic stable modes

We first consider the case of a conventional electrostatic electron–hydrogen plasma, whilst dropping the usual assumption of quasineutrality. In this case, our dispersion relation (2.17) reduces to

(3.1) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\frac{\unicode[STIX]{x1D706}_{D}^{2}}{\unicode[STIX]{x1D706}_{De}^{2}}W_{0e}+\frac{\unicode[STIX]{x1D706}_{D}^{2}}{\unicode[STIX]{x1D706}_{Di}^{2}}W_{0i}=0.\end{eqnarray}$$

In the absence of density and temperature gradients and assuming that both species have equal temperatures, i.e.  $T_{i}=T_{e},$ we can further simplify the dispersion relation to obtain

(3.2) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\frac{1}{\unicode[STIX]{x1D708}_{i}+1}[\unicode[STIX]{x1D708}_{i}\unicode[STIX]{x1D701}_{i}Z_{0i}\unicode[STIX]{x1D6E4}_{0i}+\unicode[STIX]{x1D701}_{e}Z_{0e}\unicode[STIX]{x1D6E4}_{0e}]=0.\end{eqnarray}$$

In a quasineutral hydrogen plasma, the additional constraint enforces the relation $\unicode[STIX]{x1D708}_{i}=1$ and we trivially recover equation (3.3) (e.g. Fried & Gould Reference Fried and Gould1961; Yegorenkov & Stepanov Reference Yegorenkov and Stepanov1988), which describes the plasma stability in the absence of density and temperature gradients and assuming $T_{i}=T_{e}$

(3.3) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+{\textstyle \frac{1}{2}}[\unicode[STIX]{x1D701}_{i}Z_{0i}\unicode[STIX]{x1D6E4}_{0i}+\unicode[STIX]{x1D701}_{e}Z_{0e}\unicode[STIX]{x1D6E4}_{0e}]=0.\end{eqnarray}$$

This equation has an infinite number of solutions which can be of either the ion type with $\unicode[STIX]{x1D701}_{i}\geqslant 1$ and $\unicode[STIX]{x1D701}_{e}\ll 1,$ or the electron type with $\unicode[STIX]{x1D701}_{e}\geqslant 1.$ Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ) investigate these sound wave solutions for quasineutral hydrogen plasmas, electron–positron plasmas and electron–positron–ion plasmas.

For non-neutral plasmas, we can make analytical progress for sound waves of the ion type, satisfying $\unicode[STIX]{x1D701}_{i}\gg 1$ and $\unicode[STIX]{x1D701}_{e}\ll 1$ . In this regime the following asymptotic forms of the plasma dispersion function can be used:

(3.4a,b ) $$\begin{eqnarray}Z_{0}(\unicode[STIX]{x1D701}_{i})\approx 2\text{i}\sqrt{\unicode[STIX]{x03C0}}\exp (-\unicode[STIX]{x1D701}_{i}^{2})-\frac{1}{\unicode[STIX]{x1D701}_{i}}-\frac{1}{2\unicode[STIX]{x1D701}_{i}^{3}},\quad Z_{0}(\unicode[STIX]{x1D701}_{e})=i\sqrt{\unicode[STIX]{x03C0}}-2\unicode[STIX]{x1D701}_{e},\end{eqnarray}$$

which lead to the approximated dispersion relation for sound waves of the ion type

(3.5) $$\begin{eqnarray}1+\left(\frac{\unicode[STIX]{x1D708}_{i}}{\unicode[STIX]{x1D708}_{i}+1}2\text{i}\unicode[STIX]{x1D701}_{i}\sqrt{\unicode[STIX]{x03C0}}\exp (-\unicode[STIX]{x1D701}_{i}^{2})-\frac{\unicode[STIX]{x1D708}_{i}}{\unicode[STIX]{x1D708}_{i}+1}\right)\unicode[STIX]{x1D6E4}_{0i}+O\left(\unicode[STIX]{x1D701}_{e},\frac{1}{\unicode[STIX]{x1D701}_{i}}^{2}\right)=0.\end{eqnarray}$$

For simplicity, we will neglect FLR effects implying $\unicode[STIX]{x1D6E4}_{0i}=1.$ We are also at liberty to neglect the small contributions $1/(4\unicode[STIX]{x1D701}_{i}^{2})\ll 1$ relative to the other terms. We then obtain the dispersion relation in the form

(3.6) $$\begin{eqnarray}2\text{i}\unicode[STIX]{x1D708}_{i}\unicode[STIX]{x1D701}_{i}\sqrt{\unicode[STIX]{x03C0}}\exp (-\unicode[STIX]{x1D701}_{i}^{2})+1=0.\end{eqnarray}$$

Using the notation $\unicode[STIX]{x1D701}=x-\text{i}y$ and assuming $x=\pm (y+\unicode[STIX]{x1D6E5})$ with $\unicode[STIX]{x1D6E5}\ll 1$ , we arrive at

(3.7) $$\begin{eqnarray}2\unicode[STIX]{x1D708}_{i}y\sqrt{2\unicode[STIX]{x03C0}}\text{e}^{-2y\unicode[STIX]{x1D6E5}}\exp \left[2\text{i}y^{2}-\frac{3\unicode[STIX]{x03C0}\text{i}}{4}\right]=1=\exp (2\unicode[STIX]{x03C0}\text{i}m),\quad m\in \mathbb{N}.\end{eqnarray}$$

From this, we can write down an infinite family of solutions for sound waves of the ion type as

(3.8a-c ) $$\begin{eqnarray}y_{m}=\sqrt{\unicode[STIX]{x03C0}m+\frac{3\unicode[STIX]{x03C0}}{8}}\approx \sqrt{\unicode[STIX]{x03C0}m},\quad \unicode[STIX]{x1D6E5}_{m}=\frac{1}{2y_{m}}\ln (2\unicode[STIX]{x1D708}_{i}y_{m}\sqrt{2\unicode[STIX]{x03C0}}),\quad x_{m}=y_{m}+\unicode[STIX]{x1D6E5}_{m}.\end{eqnarray}$$

One sees that $\unicode[STIX]{x1D6E5}_{m}$ increases with $\unicode[STIX]{x1D708}_{i}>1$ , i.e. the real part of the frequency increases at $\unicode[STIX]{x1D708}_{i}>1$ . The condition $\unicode[STIX]{x1D6E5}_{m}\ll 1$ is violated when $\unicode[STIX]{x1D708}_{i}$ is large enough and the asymptotic relations hitherto employed are rendered invalid.

The waves described above are simply Landau damped sound waves propagating in an electron–ion plasma slab and are stable in both non-neutral and quasineutral plasmas, a well-established result in the quasineutral case. We note that the calculations in this section are independent of the species charge and hence the same Landau damped sound waves can propagate in an electron–antiproton plasma slab. This idea has been further explored by Dubin (Reference Dubin2010) who found that such waves can propagate with only very weak Landau damping provided that the density of the heavy species is large compared to that of the light species i.e.  $\unicode[STIX]{x1D708}_{i}\gg 1.$ In the analytic model considered here, such an ordering renders the previous assumptions invalid and the asymptotic limit analysis breaks down.

However, one can of course perform a similar analysis for the case where the species fraction of the large species is much greater than one. i.e.  $\unicode[STIX]{x1D708}_{i}\gg 1.$ In this case, one employs the notation $\unicode[STIX]{x1D709}=x(1-\text{i}\unicode[STIX]{x1D6E5}).$ We obtain the results

(3.9a-c ) $$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{m}=-\frac{\unicode[STIX]{x03C0}m}{\ln (2\unicode[STIX]{x1D708}_{i}\sqrt{\unicode[STIX]{x03C0}})^{2}},\quad \unicode[STIX]{x1D714}=\ln (2\unicode[STIX]{x1D708}_{i}\sqrt{\unicode[STIX]{x03C0}}),\quad \unicode[STIX]{x1D6FE}=-\frac{\unicode[STIX]{x03C0}m}{\ln (2\unicode[STIX]{x1D708}_{i}\sqrt{\unicode[STIX]{x03C0}})^{2}},\end{eqnarray}$$

where the lowest-order mode, $m=1,$ corresponds to the sound wave solution. Hence, we obtain the result that indeed for sufficiently large ion fraction, sound waves can propagate in electron–antiproton plasmas with only weak Landau damping, in qualitative agreement with Dubin (Reference Dubin2010).

4 Density-gradient-driven modes

We now turn out attention to unstable modes by allowing gradients in the plasma profiles. Universal modes are plasma modes which can be driven unstable by density gradients. For simplicity we assume throughout this section that the temperature profiles of all species are flat and equal. We will later relax this constraint when we consider modes driven by temperature gradients. We will also once again focus our attention on electron–ion plasmas to highlight the difference between quasineutral and non-neutral plasmas in a perhaps more familiar setting.

The dispersion relation in two-component non-neutral plasma (one light species and one heavy) has the form,

(4.1) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\frac{1}{\unicode[STIX]{x1D708}_{i}+1}\mathop{\sum }_{a}\unicode[STIX]{x1D708}_{a}\unicode[STIX]{x1D701}_{a}\left(1-\frac{\unicode[STIX]{x1D714}_{\star a}}{\unicode[STIX]{x1D714}}\right)Z_{0a}\unicode[STIX]{x1D6E4}_{0a}=0,\end{eqnarray}$$

where, as introduced above, we have employed the notation $\unicode[STIX]{x1D708}_{a}=n_{a}/n_{e}$ for each species. We once again highlight the difference between quasineutral plasmas, in which $\unicode[STIX]{x1D708}_{i}=1,$ and the non-neutral plasmas considered here where $\unicode[STIX]{x1D708}_{i}$ can be completely arbitrary. Taking the limit $k_{\Vert }v_{\text{th}i}\ll \unicode[STIX]{x1D714}\ll k_{\Vert }v_{\text{th}e}$ we obtain the leading-order approximations to the plasma dispersion functions,

(4.2a,b ) $$\begin{eqnarray}Z_{0i}\approx -\frac{1}{\unicode[STIX]{x1D701}_{i}},\quad Z_{0e}\approx \text{i}\sqrt{\unicode[STIX]{x03C0}}.\end{eqnarray}$$

Hence to lowest order we obtain the dispersion relation

(4.3) $$\begin{eqnarray}(1+\unicode[STIX]{x1D708}_{i})(1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2})-\unicode[STIX]{x1D708}_{i}\left(1-\frac{\unicode[STIX]{x1D714}_{\ast i}}{\unicode[STIX]{x1D714}}\right)\unicode[STIX]{x1D6E4}_{0i}+\text{i}\unicode[STIX]{x1D708}_{e}\unicode[STIX]{x1D701}_{e}\sqrt{\unicode[STIX]{x03C0}}\left(1-\frac{\unicode[STIX]{x1D714}_{\ast e}}{\unicode[STIX]{x1D714}}\right)\unicode[STIX]{x1D6E4}_{0e}=0.\end{eqnarray}$$

Solution of this equation for $\unicode[STIX]{x1D714}=\unicode[STIX]{x1D714}_{r}+\text{i}\unicode[STIX]{x1D6FE}$ assuming $\unicode[STIX]{x1D6FE}\ll \unicode[STIX]{x1D714}_{r}$ is

(4.4a,b ) $$\begin{eqnarray}\unicode[STIX]{x1D714}_{r}=-\frac{\unicode[STIX]{x1D708}_{i}\unicode[STIX]{x1D714}_{\ast i}\unicode[STIX]{x1D6E4}_{0i}}{(1+\unicode[STIX]{x1D708}_{i})(1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2})-\unicode[STIX]{x1D708}_{i}\unicode[STIX]{x1D6E4}_{0i}},\quad \unicode[STIX]{x1D6FE}=\frac{\unicode[STIX]{x1D708}_{e}\unicode[STIX]{x1D714}_{r}\sqrt{\unicode[STIX]{x03C0}}}{k_{z}v_{\text{th}e}}(\unicode[STIX]{x1D714}_{\ast e}-\unicode[STIX]{x1D714}_{r})\unicode[STIX]{x1D6E4}_{0e}.\end{eqnarray}$$

One sees that the frequency is determined by the density gradient of the heavy species (ions) and the growth rate by the density gradient of the light species (electrons). One requires density gradients of both species to have an instability, implying that both $\unicode[STIX]{x1D714}_{r}\sim |\unicode[STIX]{x1D714}_{\ast i}|>0$ and $\unicode[STIX]{x1D6FE}\sim \unicode[STIX]{x1D714}_{\ast e}-\unicode[STIX]{x1D714}_{r}>0$ .

In the quasineutral case, the additional restriction of $\unicode[STIX]{x1D708}_{e}+\unicode[STIX]{x1D708}_{i}=2$ renders the growth rate monotonic as in Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ). This behaviour is shown in the numerical solution of the dispersion relation (2.17) in the quasineutral case shown in figure 1 where we plot the growth rate and frequency of the universal mode as a function of $\unicode[STIX]{x1D705}_{ni}$ . Here, we use the parameters $\unicode[STIX]{x1D706}_{D}/\unicode[STIX]{x1D70C}_{i}=\unicode[STIX]{x1D705}_{Te}\unicode[STIX]{x1D70C}_{i}=\unicode[STIX]{x1D705}_{Tp}\unicode[STIX]{x1D70C}_{i}=\unicode[STIX]{x1D705}_{Ti}=0,$ $k_{y}\unicode[STIX]{x1D70C}_{i}=2,k_{\Vert }\unicode[STIX]{x1D70C}_{i}=7.4\times 10^{-4}$ with the notation

(4.5a,b ) $$\begin{eqnarray}\unicode[STIX]{x1D705}_{na}=-\frac{\text{d}\ln n_{a}}{\text{d}\ln x},\quad \unicode[STIX]{x1D705}_{Ta}=-\frac{\text{d}\ln T_{a}}{\text{d}\ln x}.\end{eqnarray}$$

We note that in the quasineutral case we are forced to set the electron density gradient through the quasineutrality condition $\unicode[STIX]{x1D708}_{e}\unicode[STIX]{x1D714}_{\star e}+\unicode[STIX]{x1D708}_{i}\unicode[STIX]{x1D714}_{\star i}=0.$ In the non-neutral case however, we have another free parameter in that we may set $\unicode[STIX]{x1D705}_{ni}$ and $\unicode[STIX]{x1D705}_{ne}$ independently.

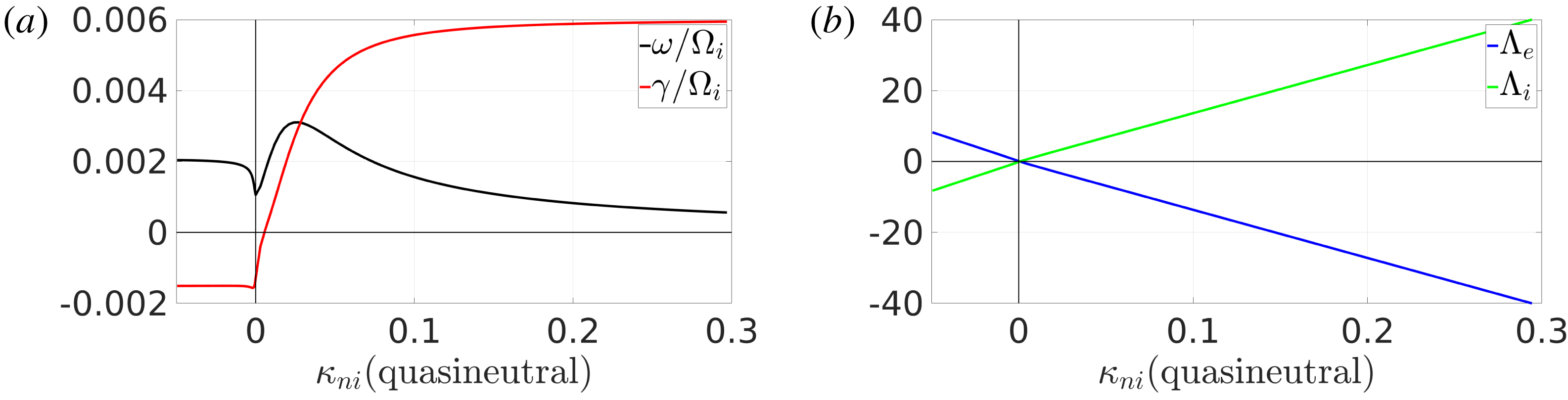
Figure 1. The frequency $\unicode[STIX]{x1D714},$ and growth rate $\unicode[STIX]{x1D6FE},$ of the universal mode (a) and the associated scaled quasilinear particle fluxes as defined by (2.24) (b) as a function of the ion density gradient $\unicode[STIX]{x1D705}_{ni}$ in a quasineutral electron–ion plasma. One sees that the ion density gradient must be larger than some threshold for the mode to become unstable. The growth rate increases monotonically with the ion density gradient. Parameters as given in the text.

Figure 2. The frequency $\unicode[STIX]{x1D714},$ and growth rate $\unicode[STIX]{x1D6FE},$ of the universal mode (a) and the associated scaled quasilinear particle fluxes (b) as a function of the electron density gradient $\unicode[STIX]{x1D705}_{ne}$ in a non-neutral electron–ion plasma. The dependence of the growth rate on the density gradient becomes non-monotonic so that a second threshold at large density gradients appears. Parameters as given in the text.

Figure 3. The frequency $\unicode[STIX]{x1D714},$ and growth rate $\unicode[STIX]{x1D6FE},$ of the universal mode (a) and the associated scaled quasilinear particle fluxes (b) as a function of the ion density gradient $\unicode[STIX]{x1D705}_{ni}$ in a non-neutral electron–ion plasma. The dependence of the growth rate on the density gradient becomes non-monotonic so that a second threshold at large density gradients appears. Parameters as given in the text.

Figure 4. The frequency $\unicode[STIX]{x1D714},$ and growth rate $\unicode[STIX]{x1D6FE},$ of the universal mode (a) and the associated scaled quasilinear particle fluxes (b) as a function of the antiproton density gradient $\unicode[STIX]{x1D705}_{ni}$ in a non-neutral electron–antiproton plasma. The dependence of the growth rate on the density gradient becomes non-monotonic so that a second threshold at large density gradients appears. Parameters as given in the text.

As such, in non-neutral plasmas, the behaviour of the instability is more interesting. We have adopted the convention that, $\unicode[STIX]{x1D714}_{\star i}<0$ and hence one expects the frequency of the universal modes to remain positive as the density profile steepens. This result lead to a monotonic growth rate in a quasineutral plasma as the growth rate was proportional to $-\unicode[STIX]{x1D714}_{r}\unicode[STIX]{x1D714}_{\star i}.$ In non-neutral plasmas, however, the growth rate depends nonlinearly on the frequency and is proportional to $\unicode[STIX]{x1D714}_{r}(\unicode[STIX]{x1D714}_{\star e}-\unicode[STIX]{x1D714}_{r}),$ so that if $\unicode[STIX]{x1D714}_{r}>\unicode[STIX]{x1D714}_{\star e}$ the growth rate decreases, which leads to a second stability threshold for the universal mode. This can be seen in figures 2 and 3 where the full dispersion relation is solved for the same parameters as above but with the assumption of quasineutrality relaxed. One would need to revisit the asymptotic analysis and include resonant contributions etc. to find the analytic stability threshold, however this can easily be found numerically when required. It is important to note that no such second stability threshold exists in standard quasineutral plasmas and that this feature is unique to non-neutral plasmas. We see a similar pattern with a sufficiently large density gradient stabilising the universal mode for electron–antiproton plasmas as shown in figure 4. It is interesting to note that instability in antiproton–electron plasmas requires $\unicode[STIX]{x1D705}_{ni}\unicode[STIX]{x1D705}_{ne}<0,$ this condition is only necessary for plasmas where each species has the same sign of charge. This result agrees with those obtained by Dubin (Reference Dubin2010) using an analytic model in a cylindrical geometry. It is also interesting to note that these modes have a different sign of frequency compared to the modes in plasmas where the different species have different signs of charge.

The scaled fluxes $\unicode[STIX]{x1D6EC}_{a}$ are also shown in figures 1–4. We immediately note once again by inspection that the quasilinear transport is ambipolar, as proven previously.

5 ETG instability

We now turn our attention to the case where temperature gradients are present in the plasma, this is likely to be of importance to the PAX/APEX investigations. In the aforementioned experiments, it is planned to confine an electron–positron plasma in a vacuum vessel using a levitated coil. In order to accomplish this goal, the electrons are to be injected with an electron gun whereas the positrons will be supplied from the research neutron source at the Technical University of Munich. This separate injection may afford different temperature profiles to the two species.

For simplicity we will now consider flat density profiles. We will find it convenient to define $\unicode[STIX]{x1D714}_{Ta}=\unicode[STIX]{x1D702}_{a}\unicode[STIX]{x1D714}_{\star a}=k_{y}T_{a}/(e_{a}B)\,\text{d}\ln T_{a}/\text{d}x,$ which is finite also at zero density gradient.

We will allow symmetry breaking not only through relaxation of the quasineutrality condition, but also by allowing each plasma species to have different temperature profiles. To this end, we introduce the notation

(5.1) $$\begin{eqnarray}\hat{\unicode[STIX]{x1D708}}_{a}=\frac{2\unicode[STIX]{x1D708}_{a}/\unicode[STIX]{x1D70F}_{a}}{\mathop{\sum }_{a^{\prime }}\unicode[STIX]{x1D708}_{a^{\prime }}/\unicode[STIX]{x1D70F}_{a^{\prime }}},\end{eqnarray}$$

where $\unicode[STIX]{x1D708}_{a}=n_{a}/n_{e}$ and $\unicode[STIX]{x1D70F}_{a}=T_{a}/T_{e}.$ It is important to note that quasineutral plasmas satisfy both $\sum _{a}\unicode[STIX]{x1D708}_{a}=2$ and $\sum _{a}\hat{\unicode[STIX]{x1D708}}_{a}=2$ whereas in non-neutral plasmas these quantities are both arbitrary. If the temperatures of all species are equal $(\unicode[STIX]{x1D70F}_{a}=1)$ in such plasmas then $\hat{\unicode[STIX]{x1D708}}_{a}=\unicode[STIX]{x1D708}_{a}.$

We seek to use our machinery to examine the behaviour of electron and positron temperature-gradient-driven modes. Using this notation and restricting our attention to the case where the only gradients present are electron and positron temperature gradients, the dispersion relation reduces to

(5.2) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\mathop{\sum }_{a=p,e,i}\frac{\hat{\unicode[STIX]{x1D708}}_{a}}{2}\unicode[STIX]{x1D701}_{a}Z_{0a}\unicode[STIX]{x1D6E4}_{0a}+\mathop{\sum }_{a=p,e}\frac{\hat{\unicode[STIX]{x1D708}}_{a}}{2}\unicode[STIX]{x1D701}_{a}\frac{\unicode[STIX]{x1D714}_{Ta}}{\unicode[STIX]{x1D714}}\left(\frac{3}{2}Z_{0a}\unicode[STIX]{x1D6E4}_{0a}-Z_{0a}\unicode[STIX]{x1D6E4}_{\star a}-Z_{2a}\unicode[STIX]{x1D6E4}_{0a}\right)=0.\end{eqnarray}$$

We assume that $k_{\bot }\unicode[STIX]{x1D70C}_{i}\gg 1$ but $k_{\bot }\unicode[STIX]{x1D70C}_{e,p}\ll 1,$ which yields

(5.3a-d ) $$\begin{eqnarray}\unicode[STIX]{x1D6E4}_{0i}=0,\quad \unicode[STIX]{x1D6E4}_{\star i}=0,\quad \unicode[STIX]{x1D6E4}_{0(e,p)}=1,\quad \unicode[STIX]{x1D6E4}_{\star (e,p)}=1,\end{eqnarray}$$

and also make the assumption of large frequencies $\unicode[STIX]{x1D714}\gg k_{\Vert }v_{\text{th}(e,p)},$ allowing us to use an asymptotic form of the plasma dispersion function. Namely, we can make use of the relations

(5.4a,b ) $$\begin{eqnarray}Z_{0}(\unicode[STIX]{x1D701}_{e,p})\approx -\frac{1}{\unicode[STIX]{x1D701}_{i}}-\frac{1}{2\unicode[STIX]{x1D701}_{i}^{3}},\quad Z_{2}(\unicode[STIX]{x1D701}_{i})\approx -\frac{1}{2\unicode[STIX]{x1D701}}-\frac{3}{4\unicode[STIX]{x1D701}^{3}},\end{eqnarray}$$

where the second expansion follows immediately from the recurrence relation $Z_{2}(\unicode[STIX]{x1D701})=\unicode[STIX]{x1D701}+\unicode[STIX]{x1D701}^{2}Z_{0}(\unicode[STIX]{x1D701})$ which itself follows straightforwardly from (2.14).

These simplifications reduce the dispersion relation in the leading order to

(5.5) $$\begin{eqnarray}\left(1-\frac{\hat{\unicode[STIX]{x1D708}}_{e}+\hat{\unicode[STIX]{x1D708}}_{p}}{2}+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}\right)+\frac{\hat{\unicode[STIX]{x1D708}}_{e}\unicode[STIX]{x1D70F}_{e}\unicode[STIX]{x1D714}_{Te}+\hat{\unicode[STIX]{x1D708}}_{p}\unicode[STIX]{x1D70F}_{p}\unicode[STIX]{x1D714}_{Tp}}{4\unicode[STIX]{x1D714}\unicode[STIX]{x1D701}_{e}^{2}}=0.\end{eqnarray}$$

This dispersion relation is valid in plasmas of arbitrary neutrality since the ion response is negligible due to $\unicode[STIX]{x1D6E4}_{0i}\approx 0$ at large $k_{\bot }\unicode[STIX]{x1D70C}_{i}$ . One can solve this leading-order approximation to the dispersion relation analytically to obtain the unstable branch

(5.6) $$\begin{eqnarray}\unicode[STIX]{x1D714}=\frac{1}{2^{1/3}}\left(\frac{\hat{\unicode[STIX]{x1D708}}_{e}\unicode[STIX]{x1D70F}_{e}\unicode[STIX]{x1D714}_{Te}+\hat{\unicode[STIX]{x1D708}}_{p}\unicode[STIX]{x1D70F}_{p}\unicode[STIX]{x1D714}_{Tp}}{2(1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2})-\hat{\unicode[STIX]{x1D708}}_{e}-\hat{\unicode[STIX]{x1D708}}_{p}}\right)^{1/3}\left(\frac{1}{2}+\text{i}\frac{\sqrt{3}}{2}\right).\end{eqnarray}$$

This equation immediately leads to the first interesting result that even pure electron plasmas can sustain unstable electron temperature-gradient-driven (ETG) modes. This is easily seen by simply noting even when $\unicode[STIX]{x1D708}_{i}=\unicode[STIX]{x1D708}_{p}=0$ the unstable branch still exists.

Another interesting result here is the existence of such modes in pair plasma with no ion contamination. In Mishchenko et al. (Reference Mishchenko, Zocco, Helander and Könies2018b ) it was found that temperature-gradient-driven instabilities can exist in pair plasmas in a slab only if the temperatures of the two species differed. However, in non-neutral plasmas the ETG mode can also be unstable even in a pure pair plasma where the electrons and positrons have the same temperature profiles provided that $\unicode[STIX]{x1D708}_{p}\neq 1.$ Again this is also seen from the asymptotic solution to the dispersion relation.

Figure 5. The frequency $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the ETG instability (a) and the associated scaled quasilinear particle fluxes (b) as a function of ion fraction $\unicode[STIX]{x1D708}_{i},$ in a non-neutral electron–ion plasma. Parameters as given in the text.

Figure 6. The frequency $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the ETG instability (a) and the associated scaled quasilinear particle fluxes (b) as a function of the positron fraction $\unicode[STIX]{x1D708}_{p},$ in non-neutral pair plasma. We note that in a non-neutral pure pair plasma (i.e. with no ion contamination) it is still possible to have temperature-gradient-driven instabilities as there is no requirement for the electron and positron contributions to cancel. Parameters as given in the text.

Figure 7. The frequency, $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the PTG instability (a) and the associated scaled quasilinear particle fluxes (b) as a function of the positron fraction $\unicode[STIX]{x1D708}_{p},$ in non-neutral pair plasma. We note that in a non-neutral pure pair plasma (i.e. with no ion contamination) it is still possible to have temperature-gradient-driven instabilities as there is no requirement for the electron and positron contributions to cancel. Parameters as given in the text.

Figure 8. The frequency, $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the ETG instability (a) and the associated scaled quasilinear particle fluxes (b) as a function of the antiproton fraction $\unicode[STIX]{x1D708}_{i},$ in non-neutral electron–antiproton plasma. Parameters as given in the text.

These behaviours are clearly seen in figures 5–7 where the full dispersion relation (2.17) is solved for the parameters $\unicode[STIX]{x1D706}_{D}/\unicode[STIX]{x1D70C}_{i}=0.1,\unicode[STIX]{x1D705}_{Te}\unicode[STIX]{x1D70C}_{i}=\unicode[STIX]{x1D705}_{Tp}\unicode[STIX]{x1D70C}_{i}=0.1,\unicode[STIX]{x1D705}_{Ti}=0,k_{y}\unicode[STIX]{x1D70C}_{i}=12$ and $k_{\Vert }\unicode[STIX]{x1D70C}_{i}=7.4\times 10^{-4},$ With different numbers and types of species being shown in each figure.

For non-neutral electron–ion plasmas, the ETG mode still exists and shows little deviation as the ion fraction is varied. This is seen in figure 5 where we note that ETG modes are unstable through a large swathe of ion fractions ranging from a quasineutral electron–ion plasma (right most point) through to a pure electron plasma (left most point). Again we see from this solution of the full dispersion relation that unstable ETG modes can exist even in pure electron plasmas. The ETG mode can also be unstable in non-neutral pair plasmas for $\unicode[STIX]{x1D708}_{p}<\unicode[STIX]{x1D708}_{e}$ (see figure 6) and the positron-temperature-gradient (PTG) driven instability can appear for $\unicode[STIX]{x1D708}_{p}>\unicode[STIX]{x1D708}_{e}$ (see figure 7). This PTG mode propagates in the opposite direction to the ETG modes.

Similarly the ETG mode also exists in electron–antiproton plasmas as shown in figure 8. The left most point in this figures of corresponds to a pure electron plasma is in agreement with the pure electron plasma limit of the previous figures.

Interestingly, both the ETG and PTG modes are stable for pair plasmas which are ‘close’ to quasineutrality, this is easily seen in on figure 6(b) and figure 7(a) where the growth rate is negative.

One area of investigation which is important experimentally is the effect of large Debye length on this class of instabilities. In fusion plasmas this effect is usually negligible as the Debye length is much smaller than the ion Larmor radius for fusion relevant parameters. However, for the pair plasma experiment under construction, this will not be the case. The Debye length of such plasmas is expected to become comparable to the proton gyroradius. One can see from (5.6) that large Debye length can have a strongly stabilising effect on the ETG and PTG instabilities in a non-neutral pair plasma.

Once again we note that the quasilinear particle fluxes obtained numerically are ambipolar. Furthermore, focussing our attention on figure 6 we can obtain a useful sanity check on our model. We note that, even though the growth rate is large, the scaled particle flux (and hence the actual quasilinear particle flux) of both species tends to zero as $\unicode[STIX]{x1D708}_{p}\rightarrow 0.$ This is again a consequence of ambipolarity.

6 ITG instability

In analogy to the ETG instability, the ITG mode can exist in non-neutral plasma with a sufficiently large ion fraction $\unicode[STIX]{x1D708}_{i}>\unicode[STIX]{x1D708}_{e}$ , see figure 9. Interestingly, the ITG mode needs some finite fraction of electrons to be unstable, in contrast to the ETG instability which we found could also exist in pure electron plasma and did not need a finite ion or positron fraction.

We restrict our attention to a non-neutral electron–ion plasma where the only gradients present are now ion temperature gradients. The dispersion relation becomes

(6.1) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}+\frac{1}{2}[\hat{\unicode[STIX]{x1D708}}_{i}\unicode[STIX]{x1D701}_{i}Z_{0i}\unicode[STIX]{x1D6E4}_{0i}+\hat{\unicode[STIX]{x1D708}}_{e}\unicode[STIX]{x1D701}_{e}Z_{0e}\unicode[STIX]{x1D6E4}_{0e}]+\frac{\hat{\unicode[STIX]{x1D708}}_{i}\unicode[STIX]{x1D714}_{Ti}\unicode[STIX]{x1D701}_{i}}{2\unicode[STIX]{x1D714}}\left(\frac{3}{2}Z_{0i}\unicode[STIX]{x1D6E4}_{0i}-Z_{0i}\unicode[STIX]{x1D6E4}_{\star i}-Z_{2i}\unicode[STIX]{x1D6E4}_{0i}\right)=0.\end{eqnarray}$$

We consider the long wavelength limit $\unicode[STIX]{x1D6E4}_{0a}=\unicode[STIX]{x1D6E4}_{\star a}=1$ for all particle species. For the ITG instability, we can assume that $k_{\Vert }v_{\text{th}i}\ll \unicode[STIX]{x1D714}\ll k_{\Vert }v_{\text{th}(e,p)}.$ Then, the plasma dispersion function can be expanded as

(6.2a-c ) $$\begin{eqnarray}Z_{0}(\unicode[STIX]{x1D701}_{i})\approx -\frac{1}{\unicode[STIX]{x1D701}_{i}}-\frac{1}{2\unicode[STIX]{x1D701}_{i}^{3}}-\frac{3}{4\unicode[STIX]{x1D701}_{i}^{5}},\quad Z_{2}(\unicode[STIX]{x1D701}_{i})\approx -\frac{1}{2\unicode[STIX]{x1D709}}-\frac{3}{4\unicode[STIX]{x1D701}^{3}},\quad Z_{0}(\unicode[STIX]{x1D701}_{e})\approx \text{i}\sqrt{\unicode[STIX]{x03C0}}.\end{eqnarray}$$

To leading order, we obtain the dispersion relation

(6.3) $$\begin{eqnarray}1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2}-\frac{\hat{\unicode[STIX]{x1D708}}_{i}}{2}=-\frac{\hat{\unicode[STIX]{x1D708}}_{i}\unicode[STIX]{x1D714}_{Ti}}{4\unicode[STIX]{x1D714}^{3}}k_{\Vert }^{2}v_{\text{th}i}^{2}.\end{eqnarray}$$

Noting that by convention $\unicode[STIX]{x1D714}_{Ti}<0,$ we obtain the unstable branch of the ITG mode

(6.4) $$\begin{eqnarray}\unicode[STIX]{x1D714}=\frac{1}{2^{1/3}}\left(\frac{\hat{\unicode[STIX]{x1D708}}_{i}|\unicode[STIX]{x1D714}_{Ti}|k_{\Vert }^{2}v_{\text{th}i}^{2}}{2(1+k_{\bot }^{2}\unicode[STIX]{x1D706}_{D}^{2})-\hat{\unicode[STIX]{x1D708}}_{i}}\right)^{1/3}\left(-\frac{1}{2}+\text{i}\frac{\sqrt{3}}{2}\right).\end{eqnarray}$$

We can see immediately from this equation that the ITG frequency is negative, as expected. One also sees, exactly the same as in the quasineutral case, the factor $(\hat{\unicode[STIX]{x1D708}}_{i}\unicode[STIX]{x1D714}_{Ti})^{1/3}$ appearing in the numerator of the growth rate. However, the dependence of the growth rate on $(\hat{\unicode[STIX]{x1D708}}_{i}\unicode[STIX]{x1D714}_{Ti})^{1/3}$ is no longer monotonic as we are no longer restricted to the range $\unicode[STIX]{x1D708}_{i}\in [0,1]$ as we were in the quasineutral case. One can also see that the ITG mode is stabilised for sufficiently large ion fraction, this can be seen from the asymptotic solution of the dispersion relation. This behaviour is seen in the numerical solution of the full dispersion relation (2.17) as shown in figure 9. Here, the dispersion relation is solved for the parameters $\unicode[STIX]{x1D706}_{D}/\unicode[STIX]{x1D70C}_{i}=0.1,$ $\unicode[STIX]{x1D705}_{T_{i}}\unicode[STIX]{x1D70C}_{i}=\unicode[STIX]{x1D705}_{T_{e}}\unicode[STIX]{x1D70C}_{i}=0.02,\unicode[STIX]{x1D705}_{n(i,e)}=0,k_{y}\unicode[STIX]{x1D70C}_{i}=0.3,k_{\Vert }\unicode[STIX]{x1D70C}_{i}=7.4\times 10^{-4}.$

There is also non-monotonic behaviour displayed by the scaled quasilinear fluxes. As seen in figure 9 there is a change in the direction of particle transport for sufficiently large ion fraction.

The antiproton temperature-gradient instability in electron–antiproton plasmas can be seen in figure 10. It is interesting to note that frequency of these waves are positive, propagating in the opposite direction to ITG-driven waves in electron–ion plasmas. Similar to the ITG instability, a finite number of antiprotons are required for the modes to become unstable.

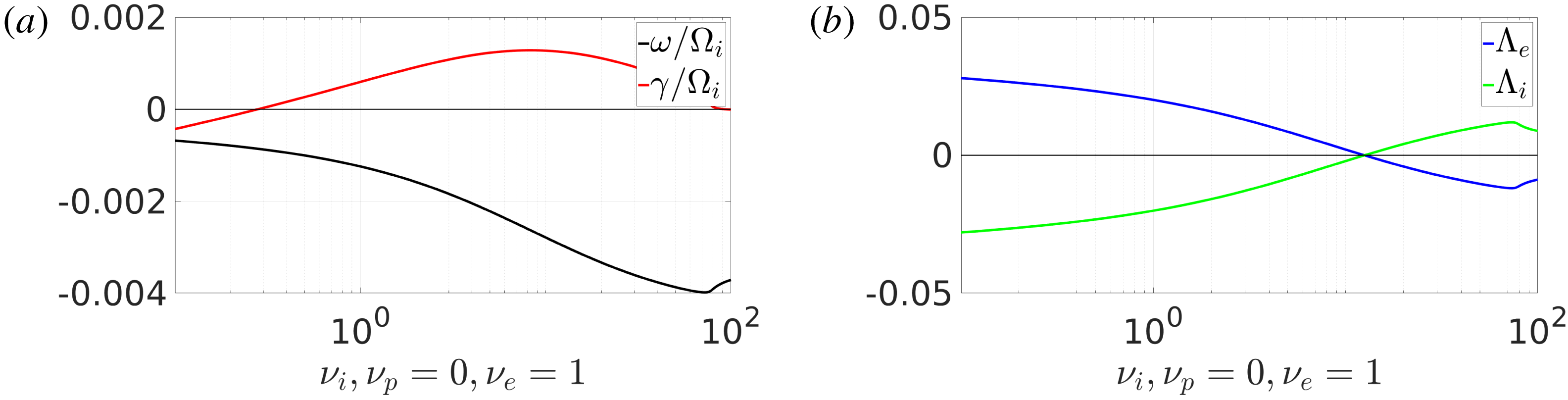
Figure 9. The frequency, $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the ITG instability (a) and associated scaled quasilinear particle fluxes (b) as a function of the ion fraction $\unicode[STIX]{x1D708}_{i},$ in non-neutral electron–ion plasma. Parameters as given in the text.

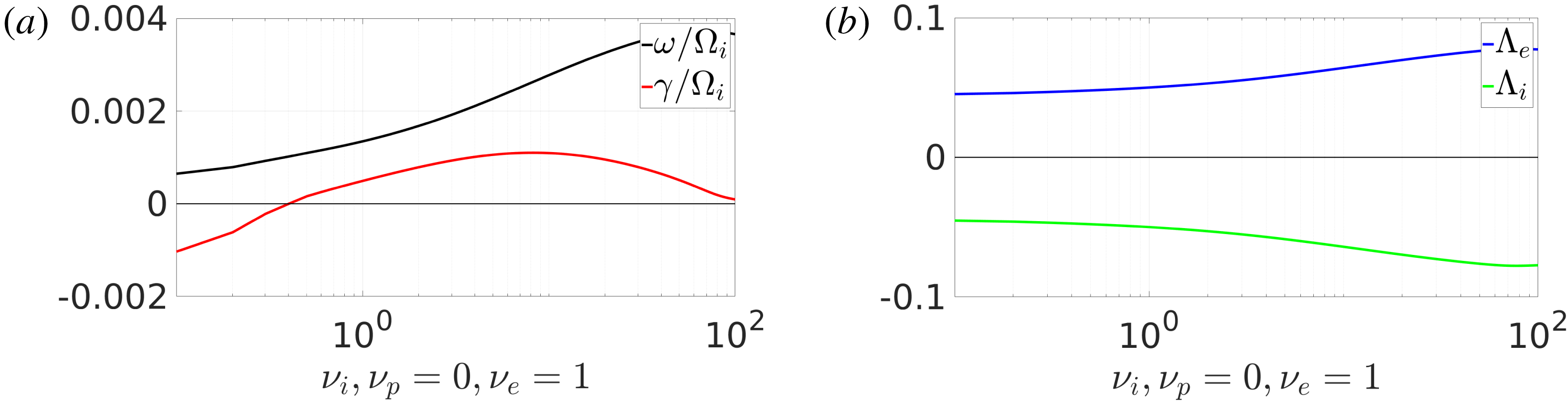
Figure 10. The frequency, $\unicode[STIX]{x1D714},$ and the growth rate $\unicode[STIX]{x1D6FE},$ of the ITG instability (a) and associated scaled quasilinear particle fluxes (b) as a function of the ion fraction $\unicode[STIX]{x1D708}_{i},$ in non-neutral electron–antiproton plasma. Parameters as given in the text.

7 Summary and discussion

In this paper, we have studied the gyrokinetic stability of non-neutral electron–positron–ion plasmas by solving, both analytically and numerically, the dispersion relation (2.17) in a slab geometry and relaxing the quasineutrality condition. It has been found that, much like their quasineutral counterparts, such non-neutral plasmas can support the gyrokinetic ITG, ETG, PTG, antiproton temperature-gradient and universal instabilities even in slab geometry. However, we found that in most cases the physics of these instabilities was different in non-neutral plasmas. We note here some of the major differences between these gyrokinetic instabilities in quasineutral and non-neutral plasmas as well as a summary of some qualitative differences which is shown in table 1.

Table 1. Qualitative differences and similarities between the different types of gyrokinetic modes arising in both quasineutral and non-neutral plasmas.

Quasilinear cross-field particle flux was investigated for each instability and it was found that the quasilinear particle flux was intrinsically ambipolar as it is in standard quasineutral gyrokinetics. We were also able to verify this numerically in each case and plot a scaled version of the particle current up to an unknown positive constant.

We found many differences between the unstable modes arising in quasineutral and non-neutral plasmas. Similarly to quasineutral plasmas, we found that non-neutral electron–ion plasmas can support the universal instability driven by a density gradient. However, in non-neutral plasmas the universal instability has a second stability threshold for large density gradients that does not exist in the quasineutral case. We found that non-neutral plasmas can also support electron and positron temperature-gradient-driven instabilities. Contrary to the case for quasineutral plasmas, we found that non-neutral pair plasmas can support both ETG and PTG modes even when each species has the same temperature and without the need for ion contamination. We also found that the ETG instability can exist even in pure electron plasmas and it can hence be reasoned that the PTG instability should also exist in a pure positron plasma. It was found that similarly to their quasineutral counterparts, the Debye length has a stabilising effect on temperature-gradient-driven instabilities. It was found that the quasilinear particle fluxes were ambipolar in each instance.

It is worth remarking that instabilities which exist even in quasineutral plasmas e.g. ETG in pure pair plasma with different species temperatures ( $T_{e}\neq T_{i}$ ) might somehow be of more importance in the non-neutral setting. In a quasineutral plasma, different electron and positron temperature profiles are unlikely in steady state, since the characteristic time of energy exchange between species is comparable to the Maxwellisation time. However, in non-neutral plasmas, such scenarios become much more physically realisable. So it might be more likely that this instability manifests experimentally in non-neutral plasmas. These results may have particular interest in the upcoming PAX/APEX experiments to investigate the stability of electron–positron plasmas. Indeed, in the aforementioned experiments it will be necessary to confine pure electron and pure positron plasmas and hence the stability of such systems is of great importance.

The ITG mode also exists in non-neutral electron–ion plasmas. However, the growth rate is no longer simply monotonic. We also found a change in the flux direction of both particle species for sufficiently large ion fraction. We also found that temperature-gradient-driven modes exist even when the heavy species and light species carry the same sign of charge. We found unstable antiproton temperature-gradient-driven modes in electron–antiproton plasmas.

We were able to use our model to investigate drift-wave instabilities in fully un-neutralised multi-species plasmas, that is, plasmas in which all species have the same type of charge, paying particular attention to electron–antiproton plasmas. We were able to make contact with some existing work on this subject such as the results of Dubin (Reference Dubin2010) who found similar results using a fluid model with a more realistic geometry.

We once again remark on some of simplifications invoked in this work. Particularly our use of a local theory including ambient electric field, always present in non-neutral plasma, but neglecting the shear of this field assuming the shear length exceeds the characteristic length of the modes discussed here. Furthermore, the slab geometry also neglects the centrifugal effect of the $\boldsymbol{E}\times \boldsymbol{B}$ plasma rotation in our stability calculations. It is pertinent to comment that experimentally these effects can and do have a destabilising influence in addition to the destabilising density- and temperature-gradient effects considered here. This area also warrants further investigation using a more sophisticated model. To claim that this model captures all the non-neutral plasma dynamics would be an egregious oversight, but hopefully the results presented here do allow us some physical insight into non-neutral plasmas, in particular highlighting some of the important distinctions between the nature of such instabilities in quasineutral and non-neutral plasmas. We plan to address the more complex systems including a shearing electric field in the future.