Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T04:53:58.119Z Has data issue: false hasContentIssue false
  • English
  • Français

Kinetic-ballooning-mode turbulence in low-average-magnetic-shear equilibria

Part of: Focus on Fusion

Published online by Cambridge University Press:  15 June 2021

I.J. McKinney Open the ORCID record for I.J. McKinney [Opens in a new window] ,
M.J. Pueschel ,
B.J. Faber Open the ORCID record for B.J. Faber [Opens in a new window] ,
C.C. Hegna ,
A. Ishizawa  and
P.W. Terry
I.J. McKinney*
Affiliation:
Department of Engineering Physics, University of Wisconsin-Madison, Madison, WI53706, USA
M.J. Pueschel
Affiliation:
Eindhoven University of Technology, 5600 MBEindhoven, The Netherlands Dutch Institute for Fundamental Energy Research, 5612 AJEindhoven, The Netherlands Institute for Fusion Studies, University of Texas at Austin, Austin, TX78712, USA
B.J. Faber
Affiliation:
Department of Engineering Physics, University of Wisconsin-Madison, Madison, WI53706, USA
C.C. Hegna
Affiliation:
Department of Engineering Physics, University of Wisconsin-Madison, Madison, WI53706, USA
A. Ishizawa
Affiliation:
Graduate School of Energy Science, Kyoto University, Uji, Kyoto611-0011, Japan
P.W. Terry
Affiliation:
Department of Physics, University of Wisconsin-Madison, Madison, WI53706, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Kinetic-ballooning-mode (KBM) turbulence is studied via gyrokinetic flux-tube simulations in three magnetic equilibria that exhibit small average magnetic shear: the Helically Symmetric eXperiment (HSX), the helical-axis Heliotron-J and a circular tokamak geometry. For HSX, the onset of KBM being the dominant instability at low wavenumber occurs at a critical value of normalized plasma pressure $\beta ^{\rm KBM}_{\rm crit}$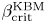 that is an order of magnitude smaller than the magnetohydrodynamic (MHD) ballooning limit $\beta ^{\rm MHD}_{\rm crit}$ 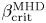when a strong ion temperature gradient (ITG) is present. However, $\beta ^{\rm KBM}_{\rm crit}$ 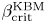increases and approaches the MHD ballooning limit as the ITG tends to zero. For these configurations, $\beta ^{\rm KBM}_{\rm crit}$ 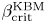also increases as the magnitude of the average magnetic shear increases, regardless of the sign of the normalized magnetic shear. Simulations of Heliotron-J and a circular axisymmetric geometry display behaviour similar to HSX with respect to $\beta ^{\rm KBM}_{\rm crit}$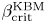. Despite large KBM growth rates at long wavelengths in HSX, saturation of KBM turbulence with $\beta > \beta _{\rm crit}^{\rm KBM}$ is achievable in HSX and results in lower heat transport relative to the electrostatic limit by a factor of roughly five. Nonlinear simulations also show that KBM transport dominates the dynamics when KBMs are destabilized linearly, even if KBM growth rates are subdominant to ITG growth rates.

Keywords

plasma instabilitiesplasma nonlinear phenomenafusion plasma
Type
Research Article
Information
Journal of Plasma Physics , Volume 87 , Issue 3 , June 2021 , 905870311
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aleynikova, K. & Zocco, A. 2017 Quantitative study of kinetic ballooning mode theory in simple geometry. Phys. Plasmas 24, 092106.CrossRefGoogle Scholar
Aleynikova, K., Zocco, A., Xanthopoulos, P., Helander, P. & Nührenberg, C. 2018 Kinetic ballooning modes in tokamaks and stellarators. J. Plasma Phys. 84, 745840602.CrossRefGoogle Scholar
Antonsen, T.M. Jr., Drake, J.F., Guzdar, P.N., Hassam, A.B., Lau, Y.T., Liu, C.S. & Novakovskii, S.V. 1996 Physical mechanism of enhanced stability from negative shear in tokamaks: implications for edge transport and the L-H transition. Phys. Plasmas 3 (6), 2221–2223.CrossRefGoogle Scholar
Antonsen, T.M. Jr. & Lane, B. 1980 Kinetic equations for low frequency instabilities in inhomogeneous plasmas. Phys. Fluids 23, 1205–1214.CrossRefGoogle Scholar
Ball, J., Brunner, S. & Ajay, C.J. 2020 Eliminating turbulent self-interaction through the parallel boundary condition in local gyrokinetic simulations. J. Plasma Phys. 86 (2), 905860207.CrossRefGoogle Scholar
Boozer, A.H. 1983 Transport and isomorphic equilibria. Phys. Fluids 26 (2), 496–499.CrossRefGoogle Scholar
Brizard, A.J. & Hahm, T.S. 2007 Foundations of nonlinear gyrokinetic theory. Rev. Mod. Phys. 79 (2), 421–468.CrossRefGoogle Scholar
Canik, J.M., Anderson, D.T., Anderson, F.S.B., Likin, K.M., Talmadge, J.N. & Zhai, K. 2007 Experimental demonstration of improved neoclassical transport with quasihelical symmetry. Phys. Rev. Lett. 98 (8), 085002.CrossRefGoogle ScholarPubMed
Cheng, C.Z. 1982 Kinetic theory of collisionless ballooning modes. Phys. Fluids 25, 1020–1026.CrossRefGoogle Scholar
Chen, W., Ma, R.R., Li, Y.Y., Shi, Z.B., Du, H.R., Jiang, M., Yu, L.M., Yuan, B.S., Li, Y.G., Yang, Z.C., et al. 2016 Alfvénic ion temperature gradient activities in a weak magnetic shear plasma. Europhys. Lett. 116, 45003.CrossRefGoogle Scholar
Chen, W., Yu, D.L., Ma, R.R., Shi, P.W., Li, Y.Y., Shi, Z.B., Du, H.R., Ji, X.Q., Jiang, M., Yu, L.M., et al. 2018 Kinetic electromagnetic instabilities in an ITB plasma with weak magnetic shear. Nucl. Fusion 58, 056004.CrossRefGoogle Scholar
Connor, J.W., Hastie, R.J. & Taylor, J.B. 1978 Shear, periodicity, and plasma ballooning modes. Phys. Rev. Lett. 40, 396–399.CrossRefGoogle Scholar
Coppi, B. 1965 ‘Universal’ instabilities from plasma moment equations. Phys. Lett. 14 (3), 172–174.CrossRefGoogle Scholar
Coppi, B., Rosenbluth, M.N. & Sadgeev, R.Z. 1967 Instabilities due to temperature gradients in complex magnetic field configurations. Phys. Fluids 10 (3), 582–587.CrossRefGoogle Scholar
Dong, J.Q., Guzdar, P.N. & Lee, Y.C. 1987 Finite beta effects on ion temperature gradient driven modes. Phys. Fluids 30, 2694–2702.CrossRefGoogle Scholar
Faber, B.J., Pueschel, M.J., Proll, J.H.E., Xanthopoulos, P., Terry, P.W., Hegna, C.C., Weir, G.M., Likin, K.M. & Talmadge, J.N. 2015 Gyrokinetic studies of trapped electron mode turbulence in Helically Symmetric eXperiment stellarator. Phys. Plasmas 22 (7), 072305.CrossRefGoogle Scholar
Faber, B.J., Pueschel, M.J., Terry, P.W., Hegna, C.C. & Roman, J.E. 2018 Stellarator microinstabilities and turbulence at low magnetic shear. J. Plasma Phys. 84 (5), 905840503.CrossRefGoogle Scholar
Gates, D.A., Anderson, D.T., Anderson, F.S.B., Zarnstorf, M., Spong, D.A., Weitzner, H., Neilson, G.H., Ruzic, D.N., Andruczyk, D., Harris, J.H., et al. 2018 Stellarator research opportunities: a report on the National Stellarator Coordinating Committee. J. Fusion Energy 37 (1), 51–94.CrossRefGoogle Scholar
Greene, J.M. & Chance, M.S. 1981 The second region of stability against ballooning modes. Nucl. Fusion 21, 453–464.CrossRefGoogle Scholar
Grimm, R.C., Greene, J.M. & Johnson, J.L. 1976 Computation of the magnetohydrodynamic spectrum in axisymmetric toroidal confinement systems. Meth. Comput. Phys. 16, 253–280.Google Scholar
Hall, L.S. & McNamara, B. 1975 Three-dimensional equilibrium of the anisotropic, finite-pressure guiding-center plasma: theory of the magnetic plasma. Phys. Fluids 18 (5), 552–565.CrossRefGoogle Scholar
Hegna, C.C., Terry, P.W. & Faber, B.J. 2018 Theory of ITG turbulent saturation in stellarators: identifying mechanisms to reduce turbulent transport. Phys. Plasmas 25 (2), 022511.CrossRefGoogle Scholar
Hirose, A. & Elia, M. 1996 Kinetic ballooning mode with negative shear. Phys. Rev. Lett. 76 (4), 628–631.CrossRefGoogle ScholarPubMed
Hirose, A., Zhang, L. & Elia, M. 1995 Ion temperature gradient-driven ballooning mode in tokamaks. Phys. Plasmas 2, 859–875.CrossRefGoogle Scholar
Hirsch, M., Baldzuhn, J., Beidler, C., Brakel, R., Burhenn, R., Dinklage, A., Ehmler, H., Endler, M., Erckmann, V., Feng, Y., et al. 2008 Major results from the stellarator Wendelstein 7-AS. Plasma Phys. Control. Fusion 50, 053001.CrossRefGoogle Scholar
Hirshman, S.P., van Rij, W.I. & Merkel, P. 1986 Three-dimensional free boundary calculations using a spectral Green's function method. Comput. Phys. commun. 43 (1), 143–155.CrossRefGoogle Scholar
Horton, W. Jr., Choi, D.-I. & Tang, W.M. 1981 Toroidal drift modes driven by ion temperature gradients. Phys. Fluids 24 (6), 1077–1085.CrossRefGoogle Scholar
Ishizawa, A., Kishimoto, Y., Watanabe, T.-H., Sugama, H., Tanaka, K., Satake, S., Kobayashi, S., Nagasaki, K. & Nakamura, Y. 2017 Multi-machine analysis of turbulent transport in helical systems via gyrokinetic simulation. Nucl. Fusion 57, 066010.CrossRefGoogle Scholar
Ishizawa, A., Maeyama, S., Watanabe, T.-H., Sugama, H. & Nakajima, N. 2013 Gyrokinetic turbulence simulations of high-beta tokamak and helical plasmas with full-kinetic and hybrid models. Nucl. Fusion 53, 053007.CrossRefGoogle Scholar
Ishizawa, A., Urano, D., Nakamura, Y., Maeyama, S. & Watanabe, T.-H. 2019 Persistence of ion temperature gradient turbulent transport at finite normalized pressure. Phys. Rev. Lett. 123 (2), 025003.CrossRefGoogle ScholarPubMed
Jarmén, A., Anderson, J. & Malinov, P. 2015 Effects of parallel ion motion on electromagnetic toroidal ion temperature gradient modes in a fluid model. Phys. Plasmas 22 (8), 082508.CrossRefGoogle Scholar
Jenko, F. 2000 Massively parallel Vlasov simulation of electromagnetic drift-wave turbulence. Compur. Phys. Commun. 125, 196–209.CrossRefGoogle Scholar
Kadomtsev, B.B. & Pogutse, O.P. 1971 Trapped particles in toroidal magnetic systems. Nucl. Fusion 11, 67–92.CrossRefGoogle Scholar
Kim, J.Y., Horton, W. & Dong, J.Q. 1993 Electromagnetic effect on the toroidal ion temperature gradient mode. Phys. Fluids B 5, 4030–4039.CrossRefGoogle Scholar
Kotschenreuther, M. 1986 Compressibility effects on ideal and kinetic ballooning modes and elimination of finite Larmor radius stabilization. Phys. Fluids 29 (9), 2898–2913.CrossRefGoogle Scholar
Maeyama, S., Ishizawa, A., Watanabe, T.-H., Nakajima, N., Tsuji-lio, S. & Tsutsui, H. 2013 Numerical techniques for parallel dynamics in electromagnetic gryokinetic Vlasov simulations. Comput. Phys. Commun. 184 (11), 2462–2473.CrossRefGoogle Scholar
McKinney, I.J., Pueschel, M.J., Faber, B.J., Hegna, C.C., Talmadge, J.N., Anderson, D.T., Mynick, H.E. & Xanthopoulos, P. 2019 A comparison of turbulent transport in a quasi-helical and a quasi-axisymmetric stellarator. J. Plasma Phys. 85, 905850503.CrossRefGoogle Scholar
Mikhailov, M.I., Shafranov, V.D., Subbotin, A.A., Yu Isaev, M., Nührenberg, J., Zille, R. & Cooper, W.A. 2002 Improved $\alpha$-particle confinement in stellarators with poloidally closed contours of the magnetic field strength. Nucl. Fusion 42, L23–L26.CrossRefGoogle Scholar
Mynick, H.E. 2006 Transport optimization in stellarators. Phys. Plasmas 13 (5), 058102.CrossRefGoogle Scholar
Mynick, H.E., Pomphrey, N. & Xanthopoulos, P. 2010 Optimizing stellarators for turbulent transport. Phys. Rev. Lett. 105 (9), 095004.CrossRefGoogle ScholarPubMed
Neilson, G.H., Zarnstorff, M.C., Lyon, J.F. & The NCSX Team 2002 Quasi-symmetry in stellarator research 5. Status of physics design of quasi-axisymmetry stellarators 5.1 Physics design of the National Compact Stellarator Experiment. J. Plasma Fusion Res. 78 (3), 214–219.CrossRefGoogle Scholar
Nührenberg, J. & Zille, R. 1988 Quasi-helically symmetric toroidal stellarators. Phys. Lett. A 129 (2), 113–117.CrossRefGoogle Scholar
Obiki, T., Sano, F., Wakatani, M., Kondo, K., Mizuuchi, T., Hanatani, K., Nakamura, Y., Nagasaki, K., Okada, H, Nakasuga, M., et al. 2000 Goals and status of Heliotron-J. Plasma Phys. Control. Fusion 42, 115–1164.CrossRefGoogle Scholar
Palumbo, D. 1968 Some considerations on closed configurations of magnetohydrostatic equilibrium. Nuovo Cimento B 53, 507–511.CrossRefGoogle Scholar
Pearlstein, L.D. & Berk, H.L. 1969 Universal eigenmode in a strongly sheared magnetic field. Phys. Rev. Lett. 23 (5), 220–222.CrossRefGoogle Scholar
Pueschel, M.J., Dannert, T. & Jenko, F. 2010 On the role of the numerical dissipation in gyrokinetic Vlasov simulations of plasma microturbulence. Comput. Phys. Commun. 181 (8), 1428–1437.CrossRefGoogle Scholar
Pueschel, M.J., Hatch, D.R., Ernst, D.R., Guttenfelder, W., Terry, P.W., Citrin, J. & Connor, J.W. 2019 On microinstabilities and turbulence in steep-gradient regions of fusion devices. Plasma Phys. Control. Fusion 61 (3), 034002.CrossRefGoogle Scholar
Pueschel, M.J. & Jenko, F. 2010 Transport properties of finite-$\beta$ microturbulence. Phys. Plasmas 17, 062307.CrossRefGoogle Scholar
Pueschel, M.J., Jenko, F., Told, D. & Buchner, J. 2011 Gyrokinetic simulations of magnetic reconnection. Phys. Plasmas 18 (11), 112102.CrossRefGoogle Scholar
Pueschel, M.J., Kammerer, M. & Jenko, F. 2008 Gyrokinetic turbulence simulations at high plasma beta. Phys. Plasmas 15, 102310.CrossRefGoogle Scholar
Pu, Y.-K. & Migliuolo, S. 1985 Finite beta stabilization of the kinetic ion mixing mode. Phys. Fluids 28, 1722–1726.CrossRefGoogle Scholar
Rodríguez, E., Helander, P. & Bhattacharjee, A. 2020 Necessary and sufficient conditions for quasisymmetry. Phys. Plasmas 27 (6), 062501.CrossRefGoogle Scholar
Ross, D.W. & Mahajan, S.M. 1978 Are drift-wave eigenmodes unstable? Phys. Rev. Lett. 40 (5), 324–327.CrossRefGoogle Scholar
Rudakov, L.I. & Sagdeev, R.Z. 1961 On the instability of a nonuniform rarefied plasma in a strong magnetic field. Dokl. Akad. Nauk SSR 138 (3), 581–583.Google Scholar
Snyder, P.B. 1999 Gyrofluid theory and simulation of electromagnetic turbulence and transport in tokamak plasmas. Princeton University. PhD thesis.Google Scholar
Snyder, P.B., Hammett, G.W., Beer, M.A. & Dorland, W. 1999 Simulations of electromagnetic turbulence and transport in tokamak plasmas. In Proc. 26th EPS Conf. on Contr. Fusion and Plasmas Physics, Europhysics Conference Abstracts (ECA) series 23J, p. 1685.Google Scholar
Strauss, H.R. 1979 Finite beta trapped electron fluid mode. Phys. Fluids 22, 1079–1081.CrossRefGoogle Scholar
Tang, W.M. 1978 Microinstability theory in tokamaks. Nucl. Fusion 18 (8), 1089–1160.CrossRefGoogle Scholar
Whelan, G.G., Pueschel, M.J. & Terry, P.W. 2018 Nonlinear electromagnetic stabilization of plasma microturbulence. Phys. Rev. Lett. 120, 175002.CrossRefGoogle ScholarPubMed
Whelan, G.G., Pueschel, M.J., Terry, P.W., Citrin, J., McKinney, I.J., Guttenfelder, W. & Doerk, H. 2019 Saturation and nonlinear electromagnetic stabilization of ITG turbulence. Phys. Plasmas 26 (8), 082302.CrossRefGoogle Scholar
Wolf, R.C., Alonso, A., Äkäslompolo, S., Baldzuhn, J., Beurskens, M., Beidler, C.D., Biedermann, C., Bosch, H.-S., Bozhenkov, S., Brakel, R., et al. 2019 Performance of Wendelstein 7-X stellarator plasmas during the first divertor operation phase. Phys. Plasmas 26, 082504.CrossRefGoogle Scholar
Xanthopoulos, P., Cooper, W.A., Jenko, F., Turkin, Y., Runov, A. & Geiger, J. 2009 A geometry interface for gyrokinetic microturbulence investigations in toroidal configurations. Phys. Plasmas 16 (8), 082303.CrossRefGoogle Scholar
Xanthopoulos, P. & Jenko, F. 2007 Gyrokinetic analysis of linear microinstabilities for the stellarator Wendelstein 7-X. Phys. Plasmas 14 (4), 042501.CrossRefGoogle Scholar
Xanthopoulos, P., Mynick, H.E., Helander, P., Turkin, Y., Plunk, G.G., Jenko, F., Görler, T., Told, D., Bird, T. & Proll, J.H.E. 2014 Controlling turbulence in present and future stellarators. Phys. Rev. Lett. 113 (15), 155011.CrossRefGoogle ScholarPubMed
Yu Isaev, M., Nührenberg, J., Mikhailov, M.I., Cooper, W.A., Watanabe, K.Y., Yokoyama, M., Yamazaki, K., Subbotin, A.A. & Shafranov, V.D. 2003 A new class of quasi-omnigenous configurations. Nucl. Fusion 43, 1066–1071.CrossRefGoogle Scholar
Zonca, F., Chen, L., Dong, J.Q. & Santoro, R.A. 1999 Existence of ion temperature gradient driven shear Alfvén instabilities in tokamaks. Phys. Plasmas 6, 1917–1924.CrossRefGoogle Scholar