Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Merlo, Gabriele
Dicorato, Mattia
Allen, Bryce
Dannert, Tilman
Germaschewski, Kai
and
Jenko, Frank
2023.
On the effect of negative triangularity on ion temperature gradient turbulence in tokamaks.
Physics of Plasmas,
Vol. 30,
Issue. 10,
Mackenbach, R.J.J.
Proll, J.H.E.
Snoep, G.
and
Helander, P.
2023.
Available energy of trapped electrons in Miller tokamak equilibria.
Journal of Plasma Physics,
Vol. 89,
Issue. 5,
Rodríguez, E.
and
Mackenbach, R.J.J.
2023.
Trapped-particle precession and modes in quasisymmetric stellarators and tokamaks: a near-axis perspective.
Journal of Plasma Physics,
Vol. 89,
Issue. 5,
Lee, W.
Zou, Q.
Wu, N.
Choi, G.J.
Xu, J.Q.
Geng, K.N.
and
Kin, F.
2024.
A summary of the 10th Asia-Pacific Transport Working Group (APTWG) meeting.
Nuclear Fusion,
Vol. 64,
Issue. 12,
p.
127001.
Mariani, A.
Balestri, A.
Mantica, P.
Merlo, G.
Ambrosino, R.
Balbinot, L.
Brioschi, D.
Casiraghi, I.
Castaldo, A.
Frassinetti, L.
Fusco, V.
Innocente, P.
Sauter, O.
and
Vlad, G.
2024.
First-principle based predictions of the effects of negative triangularity on DTT scenarios.
Nuclear Fusion,
Vol. 64,
Issue. 4,
p.
046018.
Garbet, X.
Donnel, P.
De Gianni, L.
Qu, Z.
Melka, Y.
Sarazin, Y.
Grandgirard, V.
Obrejan, K.
Bourne, E.
and
Dif-Pradalier, G.
2024.
The effect of shaping on trapped electron mode stability: an analytical model.
Nuclear Fusion,
Vol. 64,
Issue. 10,
p.
106055.
Bernard, T N
Halpern, F D
Francisquez, M
Hammett, G W
and
Marinoni, A
2024.
Plasma edge and scrape-off layer turbulence in gyrokinetic simulations of negative triangularity plasmas.
Plasma Physics and Controlled Fusion,
Vol. 66,
Issue. 11,
p.
115017.
Balestri, A
Ball, J
Coda, S
Cruz-Zabala, D J
Garcia-Munoz, M
and
Viezzer, E
2024.
Physical insights from the aspect ratio dependence of turbulence in negative triangularity plasmas.
Plasma Physics and Controlled Fusion,
Vol. 66,
Issue. 7,
p.
075012.
Vanovac, B
Dunne, M
Pütterich, T
Happel, T
Hobirk, J
Silvagni, D
Faitsch, M
Lee, K
Conway, G D
Bielajew, R
Yoo, C
White, A E
Bernert, M
David, P
Fischer, R
and
Stieglitz, D
2024.
Pedestal properties of negative triangularity discharges in ASDEX Upgrade.
Plasma Physics and Controlled Fusion,
Vol. 66,
Issue. 11,
p.
115005.
Li, Jessica L.
Chang, C. S.
Hager, Robert
Reiman, Allan
and
Zarnstorff, Michael C.
2024.
Verification of the kinetic electron role in the microinstabilities in a negative triangularity model equilibrium.
Physics of Plasmas,
Vol. 31,
Issue. 10,
Singh, Rameswar
Diamond, P.H.
and
Marinoni, A.
2025.
How does negative triangularity mitigate ITG turbulence and transport?.
Nuclear Fusion,
Vol. 65,
Issue. 2,
p.
026016.
Hoffmann, A C D
Balestri, A
and
Ricci, P
2025.
Investigation of triangularity effects on tokamak edge turbulence through multi-fidelity gyrokinetic simulations.
Plasma Physics and Controlled Fusion,
Vol. 67,
Issue. 1,
p.
015031.
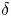 and magnetic shear $s$
and magnetic shear $s$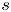 , in both ion temperature gradient and trapped electron mode dominated plasmas. Magnetic shear turns out to influence in a non-trivial way the effect of negative triangularity. Also, $\delta <0$
, in both ion temperature gradient and trapped electron mode dominated plasmas. Magnetic shear turns out to influence in a non-trivial way the effect of negative triangularity. Also, $\delta <0$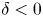 is found to lead to a reduction of fluxes when $s>1$
is found to lead to a reduction of fluxes when $s>1$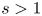 but not anymore when shear is reduced. In certain parameter regimes, this effect can be inverted, up to obtaining higher fluxes when $\delta <0$
but not anymore when shear is reduced. In certain parameter regimes, this effect can be inverted, up to obtaining higher fluxes when $\delta <0$ with respect to its $\delta >0$
with respect to its $\delta >0$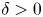 counterpart.
counterpart.