Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-29T13:20:43.208Z Has data issue: false hasContentIssue false
  • English
  • Français

Electron interactions and quantum plasma physics†

Published online by Cambridge University Press:  13 March 2009

Elizabeth A. Rauscher
Elizabeth A. Rauscher
Affiliation:
Lawrence Radiation Laboratory, University of California, Livermore, California
Get access Rights & Permissions [Opens in a new window]

Abstract

The ‘quantum electrodynamics’ of the scattering of radiation from a fully ionized, interacting plasma is to be considered.

The plasma particle interaction must properly be treated quantum mechanically when the electron plasma wave phonon energies are comparable to or greater than the mean random electron energies and/or when the phonon momenta are of the order of magnitude or greater than the average electron momenta in the plasma.

In these two circumstances or either one of them, the plasma particle inter action must be treated in a quantum mechanical manner. The ‘solid-state’ plasma recently discussed by D.E.McCumber is an example of a quantum plasma.

Whether a classical or a quantum plasma is considered, the collective properties, as well as the single-particle properties, need to be considered. The collective properties of the plasma become important when it interacts with a radiation field in the case where the electron plasma frequency, ωp, is of the same order of magnitude, or exceeds, the operating radiation frequency ω, i.e. ωp≥ω.

A criterion to distinguish the properties of a plasma as to whether it is classical or quantum mechanical in nature can be defined in terms of three fundamental lengths of the electron gas. These definitions hold for a one-component plasma. They are: the classical length βe2, the Debye screening length 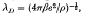and the thermal de Broglie wavelength 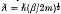defined as 1/kT. From these three quantities, we can define two dimensionless parameters. They are the classical parameter 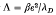and the quantum parameter δ=λ/λD which is a measure of the quantum effects. For a quantum plasma δ>1 and in the classical limit (h = 0),δ=0, Λ<1.

When we take into account the collective behaviour characterized by the plasma oscillations, screening effects are an automatic aspect of the electron plasma gas.

It is hoped that the present review article will provide the background material for general understanding of the field and easy access to the current literature. It is also hoped that the present discussion will establish greater interest in this subject.

As an example of a calculation of plasma properties, a calculation of the generalized dielectric constant for both a low-density plasma in the classical limit and a high-density plasma in the quantum mechanical limit is performed and compared in a suitable manner.

Type
Research Article
Information
Journal of Plasma Physics , Volume 2 , Issue 4 , December 1968 , pp. 517 - 541
Copyright
Copyright © Cambridge University Press 1968

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adams, J. B. & Ruderman, M. A. 1962 Neutrino Pair Emission by a Stellar Plasma, UCRL-10343.Google Scholar
Balescu, R. 1961 Phys. Fluids 4, 94.CrossRefGoogle Scholar
Bardeen, J. & Pines, B. 1955 Phys. Rev. 99, 1140.CrossRefGoogle Scholar
Bekefi, C. 1966 Radiation Processes in Plasmas. New York: John Wiley and Sons Inc.Google Scholar
Bohm, D. & Gross, E. P. 1949 a Phys. Rev. 75, 1851.CrossRefGoogle Scholar
Bohm, D. & Gross, E. P. 1949 b Phys. Rev. 75, 1864.CrossRefGoogle Scholar
Bohm, D. & Pines, D. 1951 Phys. Rev. 82, 625.CrossRefGoogle Scholar
Brout, R. 1961 J. Nucl. Energy, part C; Plasma Phys. 2, 46.CrossRefGoogle Scholar
Chappell, W. R. 1966 J. Math. Phys. 7, 1153.CrossRefGoogle Scholar
Cheng, H. & Lee, Y. C. 1966 Phys. Rev. 142, 104.CrossRefGoogle Scholar
Dawson, J. & Oberman, C. 1962 Phys. Fluids 5, 517.CrossRefGoogle Scholar
Denisse, J. F. & Delcroix, J. L. 1963 Plasma Waves, Interscience Publishers Inc.Google Scholar
DeWitt, H. 1968 Plasma Transport Theory (to be published).Google Scholar
Dong, N. Q. 1966 Phys. Rev. 148, 148.CrossRefGoogle Scholar
DuBois, D. F. 1959 Ann. Phys. 7, 174.CrossRefGoogle Scholar
DuBois, D. F. 1959 Ann. Phys. 8, 24.CrossRefGoogle Scholar
DuBois, D. F. & Gilinsky, V. 1964 Phys. Rev. 133, A 1317.CrossRefGoogle Scholar
DuBois, D. F., Gilinsky, V. & Kivelson, M. G. 1962 Phys. Rev. Lett. 8, 419.CrossRefGoogle Scholar
DuBois, D. F., Gilinsky, V. & Kivelson, M. G. 1963 Phys. Rev. 129, 2376.CrossRefGoogle Scholar
Dworin, L. 1966 Ann. Phys. 39, 43.CrossRefGoogle Scholar
Dyson, F. J. 1949 Phys. Rev. 75, 1736.CrossRefGoogle Scholar
Dyson, F. J. 1951 Phys. Rev. 83, 608.CrossRefGoogle Scholar
Fain, B. V. M. & Khanin, Ya. I. 1967 Quantum Electrons. New York: Pergamon Press Inc.Google Scholar
Feynman, R. P. 1949 Phys. Rev. 76, 769.CrossRefGoogle Scholar
Garrison, J.C. 1964 UCRL-12158.Google Scholar
Gartenhaus, S. 1964 Elements of Plasma Physics. New York: Holt, Rinehart and Winston.Google Scholar
Gell-Mann, M. 1957 Phys. Rev. 106, 369.CrossRefGoogle Scholar
Gell-Mann, M. & Brueckner, K. A. 1957 Phys. Rev. 106, 364.CrossRefGoogle Scholar
Glassgold, A. E. 1961 J. Nucl. Energy, part C; Plasma Phys. 2, 51.CrossRefGoogle Scholar
Goldman, M. V. 1966 a Ann. Phys. 38, 95.CrossRefGoogle Scholar
Goldman, M. V. 1966 b Ann. Phys. 38, 117.CrossRefGoogle Scholar
Goldman, R. & Oster, L. 1963 Phys. Rev. 129, 1469.CrossRefGoogle Scholar
Gorman, D. & Montgomery, D. 1963 Phys. Rev. 131, 7.CrossRefGoogle Scholar
Gould, H. A. & DeWitt, H. E. 1967 Phys. Rev. 155, 68.CrossRefGoogle Scholar
Grandy, W. T. Jr, & Mohling, F. 1965 Ann. Phys. 34, 424.CrossRefGoogle Scholar
Guyer, R. A. & Krumhaush, J. A. 1966 Phys. Rev. 148, 766.CrossRefGoogle Scholar
Horing, N. J. 1965 Ann. Phys. 31, 1.CrossRefGoogle Scholar
Ichimaru, S. 1962 Ann. Phys. 20, 78.CrossRefGoogle Scholar
Kidder, R. E. & DeWitt, H. E. 1961 J. Nucl. Energy, part C; Plasma Phys. 2, 218.CrossRefGoogle Scholar
Kirzhnits, D. A. 1967 Field Theoretical Methods in Many-Body Systems. New York: Pergamon Press Inc.CrossRefGoogle Scholar
Leontovich, M. A. (Editor) 1965 Reviews of Plasma Physics, volume 1. New York: Consultants Bureau.Google Scholar
Levine, H. B. 1961 J. Nucl. Energy, part C; Plasma Phys. 2, 206.CrossRefGoogle Scholar
Mallozzi, P. & Margenaw, H. 1966 Ann. Phys. 38, 177.CrossRefGoogle Scholar
Masterton, K. S. Jr, & Sawada, K. 1964 Phys. Rev. 133, A 1234.CrossRefGoogle Scholar
McCumber, D. E. 1966 Rev. Mod. Phys. 38, 494.CrossRefGoogle Scholar
McCumber, D. E. 1967 Phys. Rev. 154, 790.CrossRefGoogle Scholar
Mermin, N. D. & Canel, E. 1964 Ann. Phys. 26, 247.CrossRefGoogle Scholar
Northrop, T. G. 1963 The Adiabatic Motion of Charged Particles. New York: Interscience Publishers Inc.CrossRefGoogle Scholar
Perel, V. I. & Ellasberg, G. M. 1962 Soviet Phys. JETP 14, 633.Google Scholar
Pines, D. 1961 a The many-body problem. In Frontiers in Physics, W. A. Benjamin Inc.Google Scholar
Pines, D. 1961 b J. Nucl. Energy, part C; Plasma Phys. 2, 5.CrossRefGoogle Scholar
Pradhan, T. 1962 Ann. Phys. 17, 418.CrossRefGoogle Scholar
Pytte, A. & Blanken, R. 1964 Phys. Rev. 133, A 668.CrossRefGoogle Scholar
Quinn, J. J. & Ferrell, R. A. 1961 J. Nucl. Energy, part C; Plasma Phys. 2, 18.CrossRefGoogle Scholar
Rand, S. 1964 Phys. Fluids 7, 64.CrossRefGoogle Scholar
Rand, S. 1965 Phys. Fluids 8, 143.CrossRefGoogle Scholar
Rice, T. M. 1965 Ann. Phys. 31, 100.CrossRefGoogle Scholar
Ron, A. & Tzoar, N. 1962 Phys. Rev. 131, 12.CrossRefGoogle Scholar
Ron, A. & Tzoar, N. 1963 a Phys. Rev. 131, 1943.CrossRefGoogle Scholar
Ron, A. & Tzoar, N. 1963 b Phys. Rev. Lett. 10. 45.CrossRefGoogle Scholar
Ron, A. & Tzoar, N. 1964 Phys. Rev. 133, A 1378.CrossRefGoogle Scholar
Sawada, K. 1957 Phys. Rev. 106, 372.CrossRefGoogle Scholar
Schmidt, C. 1961 J. Nucl. Energy, part C; Plasma Phys. 3, 156.CrossRefGoogle Scholar
Schweber, S. S. 1961 Introduction to Relativistic Quantum Field Theory. Illinois: Row, Peterson and Co.Google Scholar
Spitzer, L. Jr, 1962 Physics of Fully Ionized Gases. New York: Interscience Publishers Inc.Google Scholar
Stack, J. D. & Sessler, A. M. 1963 UCRL-10628.Google Scholar
Theimer, O. 1963 Ann. Phys. 22, 102.CrossRefGoogle Scholar
Thouless, D. J. 1961 The Quantum Mechanics of Many-body Systems (Pure and Applied Physics, London, England).Google Scholar
Tsytovich, V. N. 1961 Soviet Phys. JETP 13, 1249.Google Scholar
Weinberg, S. 1962 Phys. Rev. 126, 1899.CrossRefGoogle Scholar
Ziman, J. M. 1960 Electrons and Phonons. Oxford University Press.Google Scholar