Article contents
Comparative study of dust ion acoustic Korteweg–de Vries and modified Korteweg–de Vries solitons in dusty plasmas with variable temperatures
Published online by Cambridge University Press: 05 October 2017
Abstract
In this plasma model, consisting of ions and electrons with pressure variations in both the components in the presence of stationary dust, both compressive and rarefactive Korteweg–de Vries (KdV) solitons of interesting character are established. Based on high dust charge, characteristics of soliton growth are found to be amplified for various pairs of ion and electron streaming speeds. It is noteworthy to mention that for some pairs of ion and electron initial streaming speeds, only compressive KdV solitons with either decreasing or increasing growth are shown to reflect. Contrary to this, for some other pairs of ion and electron streaming speeds, the amplitudes of both rarefactive and compressive solitons are seen to be produced, changing from rarefactive to compressive growth. At the stationary background of the massive dust particles, the lighter particles suffer appreciable initial drifts (backwards streaming) which characteristically change the growth of solitons. For inclusion of higher-order nonlinearity, only compressive modified Korteweg–de Vries (MKdV) solitons of much higher amplitude are found to exist whereas for the same set of parameter values both compressive and rarefactive KdV solitons are found to exist. Smaller values of electron streaming speed are seen to produce high amplitude MKdV solitons. We also observe that due to higher-order nonlinearity, the nonlinear monotonic growth of amplitudes of MKdV solitons is supported by the almost equal streaming speed pairs of ions and electrons for relatively small values of 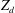 $Z_{d}$, where
$Z_{d}$, where 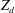 $Z_{d}$ is the number of charges in a dust particle
$Z_{d}$ is the number of charges in a dust particle
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2017
References
- 12
- Cited by


