Published online by Cambridge University Press: 06 September 2019
Stellarators are a promising route to steady-state fusion power. However, to achieve the required confinement, the magnetic geometry must be highly optimized. This optimization requires navigating high-dimensional spaces, often necessitating the use of gradient-based methods. The gradient of the neoclassical fluxes is expensive to compute with classical methods, requiring 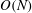 $O(N)$ flux computations, where
$O(N)$ flux computations, where  $N$ is the number of parameters. To reduce the cost of the gradient computation, we present an adjoint method for computing the derivatives of moments of the neoclassical distribution function for stellarator optimization. The linear adjoint method allows derivatives of quantities which depend on solutions of a linear system, such as moments of the distribution function, to be computed with respect to many parameters from the solution of only two linear systems. This reduces the cost of computing the gradient to the point that the finite-collisionality neoclassical fluxes can be used within an optimization loop. With the neoclassical adjoint method, we compute solutions of the drift kinetic equation and an adjoint drift kinetic equation to obtain derivatives of neoclassical quantities with respect to geometric parameters. When the number of parameters in the derivative is large (
$N$ is the number of parameters. To reduce the cost of the gradient computation, we present an adjoint method for computing the derivatives of moments of the neoclassical distribution function for stellarator optimization. The linear adjoint method allows derivatives of quantities which depend on solutions of a linear system, such as moments of the distribution function, to be computed with respect to many parameters from the solution of only two linear systems. This reduces the cost of computing the gradient to the point that the finite-collisionality neoclassical fluxes can be used within an optimization loop. With the neoclassical adjoint method, we compute solutions of the drift kinetic equation and an adjoint drift kinetic equation to obtain derivatives of neoclassical quantities with respect to geometric parameters. When the number of parameters in the derivative is large (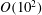 $O(10^{2})$), this adjoint method provides up to a factor of 200 reduction in cost. We demonstrate adjoint-based optimization of the field strength to obtain minimal bootstrap current on a surface. With adjoint-based derivatives, we also compute the local sensitivity to magnetic perturbations on a flux surface and identify regions where tight tolerances on error fields are required for control of the bootstrap current or radial transport. Furthermore, the solve for the ambipolar electric field is accelerated using a Newton method with derivatives obtained from the adjoint method.
$O(10^{2})$), this adjoint method provides up to a factor of 200 reduction in cost. We demonstrate adjoint-based optimization of the field strength to obtain minimal bootstrap current on a surface. With adjoint-based derivatives, we also compute the local sensitivity to magnetic perturbations on a flux surface and identify regions where tight tolerances on error fields are required for control of the bootstrap current or radial transport. Furthermore, the solve for the ambipolar electric field is accelerated using a Newton method with derivatives obtained from the adjoint method.