Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T14:13:48.519Z Has data issue: false hasContentIssue false
  • English
  • Français

Particle acceleration in neutron star ultra-strong electromagnetic fields

Part of: The Many Facets of the Vlasov Equation Focus on Plasma Astrophysics

Published online by Cambridge University Press:  25 August 2020

Ivan Tomczak Open the ORCID record for Ivan Tomczak [Opens in a new window]  and
Jérôme Pétri Open the ORCID record for Jérôme Pétri [Opens in a new window]
Ivan Tomczak*
Affiliation:
Université de Strasbourg, CNRS, Observatoire astronomique de Strasbourg, UMR 7550, F-67000Strasbourg, France
Jérôme Pétri*
Affiliation:
Université de Strasbourg, CNRS, Observatoire astronomique de Strasbourg, UMR 7550, F-67000Strasbourg, France
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we discuss the results of a new particle pusher in realistic ultra-strong electromagnetic fields such as those encountered around rotating neutron stars. After presenting the results of this algorithm in simple fields and comparing them to expected exact analytical solutions, we present new simulations for a rotating magnetic dipole in vacuum for a millisecond pulsar by using the Deutsch solution. Particles are injected within the magnetosphere, neglecting radiation reaction, interaction among them and their feedback on the fields. Our simulations are therefore not yet fully self-consistent because the Maxwell equations are not solved according to the current produced by these particles. The code highlights the symmetrical behaviour of particles of opposite charge to mass ratio, $q/m$, with respect to the north and south hemispheres. The relativistic Lorentz factor 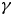$\gamma$ of the accelerated particles is proportional to this ratio $q/m$: protons reach up to 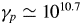$\gamma _p \simeq 10^{10.7}$, whereas electrons reach up to 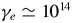$\gamma _e \simeq 10^{14}$. Our simulations show that particles could either be captured by the neutron star, trapped around it or ejected far from it, well outside the light cylinder. Actually, for a given charge to mass ratio, particles follow similar trajectories. These particle orbits show some depleted directions, especially at high magnetic inclination with respect to the rotation axis for positive charges and at low inclination for negative charges because of symmetry. Other directions are preferred and loaded with a high density of particles, some directions concentrating the highest or lowest acceleration efficiencies.

Keywords

astrophysical plasmasplasma simulation
Type
Research Article
Information
Journal of Plasma Physics , Volume 86 , Issue 4 , August 2020 , 825860401
Copyright
Copyright © The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Arefiev, A. V., Cochran, G. E., Schumacher, D. W., Robinson, A. P. L. & Chen, G. 2015 Temporal resolution criterion for correctly simulating relativistic electron motion in a high-intensity laser field. Phys. Plasmas 22 (1), 013103.CrossRefGoogle Scholar
Arfken, G. B. & Weber, H.-J. 2005 Mathematical Methods for Physicists, 6th edn. Elsevier.Google Scholar
Boris, J. P. 1970 Relativistic plasma simulation-optimization of a hybrid code. In Proceeding of Fourth Conference on Numerical Simulations of Plasmas.Google Scholar
Brambilla, G., Kalapotharakos, C., Timokhin, A. N., Harding, A. K. & Kazanas, D. 2018 Electron-positron pair flow and current composition in the pulsar magnetosphere. Astrophys. J. 858 (2), 81.CrossRefGoogle Scholar
Cerutti, B., Philippov, A., Parfrey, K. & Spitkovsky, A. 2015 Particle acceleration in axisymmetric pulsar current sheets. Mon. Not. R. Astron. Soc. 448, 606–619.CrossRefGoogle Scholar
Deutsch, A. J. 1955 The electromagnetic field of an idealized star in rigid rotation in vacuo. Ann. Astrophys. 18, 1.Google Scholar
Gourgoulhon, E. 2010 Relativité restreinte: Des particules à l'astrophysique. EDP Sciences.Google Scholar
Guépin, C., Cerutti, B. & Kotera, K. 2019 Proton acceleration in pulsar magnetospheres. arXiv:1910.11387.CrossRefGoogle Scholar
Higuera, A. V. & Cary, J. R. 2017 Structure-preserving second-order integration of relativistic charged particle trajectories in electromagnetic fields. Phys. Plasmas 24 (5), 052104.CrossRefGoogle Scholar
Kalapotharakos, C., Brambilla, G., Timokhin, A., Harding, A. K. & Kazanas, D. 2018 Three-dimensional kinetic pulsar magnetosphere models: connecting to gamma-ray observations. Astrophys. J. 857 (1), 44.CrossRefGoogle Scholar
Krause-Polstorff, J. & Michel, F. C. 1985 Electrosphere of an aligned magnetized neutron star. Mon. Not. R. Astron. Soc. 213, 43P–49P.CrossRefGoogle Scholar
Lapenta, G. & Markidis, S. 2011 Particle acceleration and energy conservation in particle in cell simulations. Phys. Plasmas 18 (7), 072101.CrossRefGoogle Scholar
Laue, H. & Thielheim, K. O. 1986 Acceleration of protons and electrons in the electromagnetic field of a rotating orthogonal magnetic dipole. Astrophys. J. Suppl. 61, 465–478.CrossRefGoogle Scholar
Li, J., Spitkovsky, A. & Tchekhovskoy, A. 2012 Resistive solutions for pulsar magnetospheres. Astrophys. J. 746 (1), 60.CrossRefGoogle Scholar
Michel, F. C. & Li, H. 1999 Electrodynamics of neutron stars. Phys. Rep. 318 (6), 227–297.CrossRefGoogle Scholar
Pétri, J. 2017 A fully implicit scheme for numerical integration of the relativistic particle equation of motion. J. Plasma Phys. 83 (2).CrossRefGoogle Scholar
Pétri, J. 2020 a Electrodynamics and radiation from rotating neutron star magnetospheres. Mon. Not. R. Astron. Soc. 6 (1), 15.Google Scholar
Pétri, J. 2020 b Radiative pulsar magnetospheres: aligned rotator. Mon. Not. R. Astron. Soc. 491, L46–L50.CrossRefGoogle Scholar
Pétri, J. 2020 c A relativistic particle pusher for ultra-strong electromagnetic fields. arXiv:1910.04591.Google Scholar
Pétri, J., Heyvaerts, J. & Bonazzola, S. 2002 Global static electrospheres of charged pulsars. Astron. Astrophys. 384, 414–432.CrossRefGoogle Scholar
Philippov, A. A. & Spitkovsky, A. 2014 Ab initio pulsar magnetosphere: three-dimensional particle-in-cell simulations of axisymmetric pulsars. Astrophys. J. Lett. 785 (2), L33.CrossRefGoogle Scholar
Qin, H., Zhang, S., Xiao, J., Liu, J., Sun, Y. & Tang, W. M. 2013 Why is Boris algorithm so good? Phys. Plasmas 20 (8), 084503.CrossRefGoogle Scholar
Ripperda, B., Bacchini, F., Teunissen, J., Xia, C., Porth, O., Sironi, L., Lapenta, G. & Keppens, R. 2018 A comprehensive comparison of relativistic particle integrators. Astrophys. J. Suppl. 235 (1), 21.CrossRefGoogle Scholar
Umeda, T. 2018 A three-step Boris integrator for Lorentz force equation of charged particles. Comput. Phys. Commun. 228, 1–4.CrossRefGoogle Scholar
Uzan, J.-P. & Deruelle, N. 2014 Théories de la Relativité. Belin.Google Scholar
Vay, J.-L. 2008 Simulation of beams or plasmas crossing at relativistic velocity. Phys. Plasmas 15 (5), 056701.CrossRefGoogle Scholar
Verboncoeur, J. P. 2005 Particle simulation of plasmas: review and advances. Plasma Phys. Control. Fusion 47 (5A), A231.CrossRefGoogle Scholar
Vranic, M., Martins, J. L., Fonseca, R. A. & Silva, L. O. 2016 Classical radiation reaction in particle-in-cell simulations. Comput. Phys. Commun. 204, 141–151.CrossRefGoogle Scholar
Zenitani, S. & Umeda, T. 2018 On the Boris solver in particle-in-cell simulation. arXiv:1809.04378.CrossRefGoogle Scholar
Zhang, R., Liu, J., Qin, H., Wang, Y., He, Y. & Sun, Y. 2015 Volume-preserving algorithm for secular relativistic dynamics of charged particles. Phys. Plasmas 22 (4), 044501.CrossRefGoogle Scholar