Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhu, Hongxuan
Zhou, Yao
Ruiz, D. E.
and
Dodin, I. Y.
2018.
Wave kinetics of drift-wave turbulence and zonal flows beyond the ray approximation.
Physical Review E,
Vol. 97,
Issue. 5,
Zhu, Hongxuan
Zhou, Yao
and
Dodin, I. Y.
2018.
On the structure of the drifton phase space and its relation to the Rayleigh–Kuo criterion of the zonal-flow stability.
Physics of Plasmas,
Vol. 25,
Issue. 7,
Zhu, Hongxuan
Zhou, Yao
and
Dodin, I. Y.
2018.
On the Rayleigh–Kuo criterion for the tertiary instability of zonal flows.
Physics of Plasmas,
Vol. 25,
Issue. 8,
Parker, Jeffrey B.
2018.
Numerical simulation of the geometrical-optics reduction of CE2 and comparisons to quasilinear dynamics.
Physics of Plasmas,
Vol. 25,
Issue. 5,
Qi, Di
Majda, Andrew J.
and
Cerfon, Antoine J.
2019.
A flux-balanced fluid model for collisional plasma edge turbulence: Numerical simulations with different aspect ratios.
Physics of Plasmas,
Vol. 26,
Issue. 8,
Zhu, Hongxuan
Zhou, Yao
and
Dodin, I Y
2019.
Nonlinear saturation and oscillations of collisionless zonal flows.
New Journal of Physics,
Vol. 21,
Issue. 6,
p.
063009.
Zielinski, J.
Becoulet, M.
Smolyakov, A. I.
Garbet, X.
Huijsmans, G. T. A.
Beyer, P.
and
Benkadda, S.
2020.
Global ITG eigenmodes: From ballooning angle and radial shift to Reynolds stress and nonlinear saturation.
Physics of Plasmas,
Vol. 27,
Issue. 7,
Zhou, Yao
Zhu, Hongxuan
and
Dodin, I Y
2020.
Solitary zonal structures in subcritical drift waves: a minimum model.
Plasma Physics and Controlled Fusion,
Vol. 62,
Issue. 4,
p.
045021.
Qi, Di
and
Majda, Andrew J.
2020.
Nonlinear interaction and turbulence transition in the limiting regimes of plasma edge turbulence.
Research in the Mathematical Sciences,
Vol. 7,
Issue. 3,
Qi, Di
and
Majda, Andrew J.
2020.
Flux-balanced two-field plasma edge turbulence in a channel geometry.
Physics of Plasmas,
Vol. 27,
Issue. 3,
Ivanov, Plamen G.
Schekochihin, A. A.
Dorland, W.
Field, A. R.
and
Parra, F. I.
2020.
Zonally dominated dynamics and Dimits threshold in curvature-driven ITG turbulence.
Journal of Plasma Physics,
Vol. 86,
Issue. 5,
Zhu, Hongxuan
Zhou, Yao
and
Dodin, I. Y.
2020.
Theory of the Tertiary Instability and the Dimits Shift from Reduced Drift-Wave Models.
Physical Review Letters,
Vol. 124,
Issue. 5,
Qi, Di
Majda, Andrew J.
and
Cerfon, Antoine J.
2020.
Dimits shift, avalanche-like bursts, and solitary propagating structures in the two-field flux-balanced Hasegawa–Wakatani model for plasma edge turbulence.
Physics of Plasmas,
Vol. 27,
Issue. 10,
Zhu, Hongxuan
Zhou, Yao
and
Dodin, I. Y.
2020.
Theory of the tertiary instability and the Dimits shift within a scalar model.
Journal of Plasma Physics,
Vol. 86,
Issue. 4,
Hallenbert, Axel
and
Plunk, Gabriel G.
2021.
Predicting the Dimits shift through reduced mode tertiary instability analysis in a strongly driven gyrokinetic fluid limit.
Journal of Plasma Physics,
Vol. 87,
Issue. 5,
Pueschel, M.J.
Li, P.-Y.
and
Terry, P.W.
2021.
Predicting the critical gradient of ITG turbulence in fusion plasmas.
Nuclear Fusion,
Vol. 61,
Issue. 5,
p.
054003.
Li, P.-Y.
Terry, P. W.
Whelan, G. G.
and
Pueschel, M. J.
2021.
Saturation physics of threshold heat-flux reduction.
Physics of Plasmas,
Vol. 28,
Issue. 10,
Zhu, Hongxuan
and
Dodin, I. Y.
2021.
Wave-kinetic approach to zonal-flow dynamics: Recent advances.
Physics of Plasmas,
Vol. 28,
Issue. 3,
Terry, P. W.
Li, P.-Y.
Pueschel, M. J.
and
Whelan, G. G.
2021.
Threshold Heat-Flux Reduction by Near-Resonant Energy Transfer.
Physical Review Letters,
Vol. 126,
Issue. 2,
Ivanov, Plamen G.
Schekochihin, A.A.
and
Dorland, W.
2022.
Dimits transition in three-dimensional ion-temperature-gradient turbulence.
Journal of Plasma Physics,
Vol. 88,
Issue. 5,
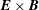 $\boldsymbol{E}\times \boldsymbol{B}$ nonlinearity that can efficiently transfer energy non-locally to length scales of the order of the sound radius. The size of the shift for the nonlinear system is heuristically calculated and found to be in excellent agreement with numerical solutions. The existence of the Dimits shift for this system is then understood as an ability of the unstable primary modes to efficiently couple to stable modes at smaller scales, and the shift ends when these stable modes eventually destabilize as the density gradient is increased. This non-local mechanism of energy transfer is argued to be generically important even for more physically complete systems.
$\boldsymbol{E}\times \boldsymbol{B}$ nonlinearity that can efficiently transfer energy non-locally to length scales of the order of the sound radius. The size of the shift for the nonlinear system is heuristically calculated and found to be in excellent agreement with numerical solutions. The existence of the Dimits shift for this system is then understood as an ability of the unstable primary modes to efficiently couple to stable modes at smaller scales, and the shift ends when these stable modes eventually destabilize as the density gradient is increased. This non-local mechanism of energy transfer is argued to be generically important even for more physically complete systems.

