Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-24T02:58:51.988Z Has data issue: false hasContentIssue false
  • English
  • Français

The electromotive force in multi-scale flows at high magnetic Reynolds number

Part of: Macroscopic Randomness

Published online by Cambridge University Press:  21 September 2015

Steven M. Tobias  and
Fausto Cattaneo
Steven M. Tobias*
Affiliation:
Department of Applied Mathematics, University of Leeds, Leeds LS8 1DS, UK
Fausto Cattaneo
Affiliation:
Department of Astronomy and Astrophysics, University of Chicago, 5640 South Ellis Avenue, Chicago, IL 60637, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Recent advances in dynamo theory have been made by examining the competition between small- and large-scale dynamos at high magnetic Reynolds number 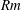$\mathit{Rm}$ . Small-scale dynamos rely on the presence of chaotic stretching whilst the generation of large-scale fields occurs in flows lacking reflectional symmetry via a systematic electromotive force (EMF). In this paper we discuss how the statistics of the EMF (at high  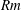$\mathit{Rm}$ ) depend on the properties of the multi-scale velocity that is generating it. In particular, we determine that different scales of flow have different contributions to the statistics of the EMF, with smaller scales contributing to the mean without increasing the variance. Moreover, we determine when scales in such a flow act independently in their contribution to the EMF. We further examine the role of large-scale shear in modifying the EMF. We conjecture that the distribution of the EMF, and not simply the mean, largely determines the dominant scale of the magnetic field generated by the flow.

Type
Research Article
Information
Journal of Plasma Physics , Volume 81 , Issue 6 , December 2015 , 395810601
Copyright
© Cambridge University Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Augustson, K., Brun, A. S., Miesch, M. & Toomre, J. 2015 Grand minima and equatorward propagation in a cycling stellar convective dynamo. Astrophys. J. 809, 149.Google Scholar
Boldyrev, S., Cattaneo, F. & Rosner, R. 2005 Magnetic-field generation in helical turbulence. Phys. Rev. Lett. 95 (25), 255001.Google Scholar
Brandenburg, A. & Subramanian, K. 2005 Astrophysical magnetic fields and nonlinear dynamo theory. Phys. Rep. 417, 1–209.Google Scholar
Cattaneo, F. & Tobias, S. M. 2005 Interaction between dynamos at different scales. Phys. Fluids 17 (12), 127105,1–6.Google Scholar
Cattaneo, F. & Tobias, S. M. 2014 On large-scale dynamo action at high magnetic Reynolds number. Astrophys. J. 789, 70.CrossRefGoogle Scholar
Childress, S. & Gilbert, A. D. 1995 Stretch, twist, fold. In The Fast Dynamo, XI, Lecture Notes in Physics, vol. 37, p. 406. Springer.Google Scholar
Courvoisier, A., Hughes, D. W. & Tobias, S. M. 2006 ${\it\alpha}$ effect in a family of chaotic flows. Phys. Rev. Lett. 96 (3), 034503.Google Scholar
Courvoisier, A. & Kim, E.-J. 2009 Kinematic ${\it\alpha}$ effect in the presence of a large-scale motion. Phys. Rev. E 80 (4), 046308.Google Scholar
Cowling, T. G. 1933 The magnetic field of sunspots. Mon. Not. R. Astron. Soc. 94, 39–48.Google Scholar
Finn, J. M. & Ott, E. 1988 Chaotic flows and fast magnetic dynamos. Phys. Fluids 31, 2992–3011.Google Scholar
Galloway, D. J. & Proctor, M. R. E. 1992 Numerical calculations of fast dynamos in smooth velocity fields with realistic diffusion. Nature 356, 691–693.Google Scholar
Hargittai, I. 2013 Buried Glory: Portraits of Soviet Scientists. Oxford University Press.Google Scholar
Käpylä, P. J., Korpi, M. J. & Brandenburg, A. 2010 The ${\it\alpha}$ effect in rotating convection with sinusoidal shear. Mon. Not. R. Astron. Soc. 402, 1458–1466.CrossRefGoogle Scholar
Krause, F. & Raedler, K. H. 1980 Mean-Field Magnetohydrodynamics and Dynamo Theory. Pergamon.Google Scholar
Moffatt, H. K. 1978 Magnetic Field Generation in Electrically Conducting Fluids. Cambridge University Press.Google Scholar
Plunian, F. & Rädler, K.-H. 2002 Subharmonic dynamo action in the Roberts flow. Geophys. Astrophys. Fluid Dyn. 96, 115–133.Google Scholar
Roberts, G. O. 1972 Dynamo action of fluid motions with two-dimensional periodicity. Phil. Trans. R. Soc. Lond. A 271, 411–454.Google Scholar
Steenbeck, M., Krause, F. & Rädler, K.-H. 1966 Berechnung der mittleren LORENTZ-Feldstärke für ein elektrisch leitendes medium in turbulenter, durch CORIOLIS-Kräfte beeinflußter Bewegung. Z. Naturforsch. Teil A 21, 369.Google Scholar
Sunyaev, R. A.(Ed.) 2004 Zeldovich Reminiscences. Chapman & Hall.Google Scholar
Tobias, S. M. & Cattaneo, F. 2008a Dynamo action in complex flows: the quick and the fast. J. Fluid Mech. 601 (1), 101–122.Google Scholar
Tobias, S. M. & Cattaneo, F. 2008b Limited role of spectra in dynamo theory: coherent versus random dynamos. Phys. Rev. Lett. 101 (12), 125003.Google Scholar
Tobias, S. M. & Cattaneo, F. 2013 Shear-driven dynamo waves at high magnetic Reynolds number. Nature 497, 463–465.Google Scholar
Tobias, S. M., Cattaneo, F. & Boldyrev, S. 2013 Ten Chapters in Turbulence (ed. Davidson, P. A., Kaneda, Y. & Sreenivasan, K. R.), pp. 351–404. Cambridge University Press.Google Scholar
Tobias, S. M., Cattaneo, F. & Brummell, N. H. 2008 Convective dynamos with penetration, rotation, and shear. Astrophys. J. 685, 596–605.Google Scholar
Vainshtein, S. I. & Kichatinov, L. L. 1986 The dynamics of magnetic fields in a highly conducting turbulent medium and the generalized Kolmogorov–Fokker–Planck equations. J. Fluid Mech. 168, 73–87.Google Scholar
Zel’dovich, Y. B. 1957 The magnetic field in the two-dimensional motion of a conducting turbulent fluid. Sov. Phys. JETP 4, 460–462.Google Scholar