Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-27T14:49:16.307Z Has data issue: false hasContentIssue false
  • English
  • Français

A 3-D MHD equilibrium description of nonlinearly saturated ideal external kink/peeling structures in tokamaks

Part of: PLA Tribute to V D Shafranov: 1929-2014 Focus on Fusion

Published online by Cambridge University Press:  01 December 2015

W. A. Cooper ,
J. P. Graves ,
B. P. Duval ,
L. Porte ,
H. Reimerdes ,
O. Sauter  and
T.-M. Tran
W. A. Cooper*
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
J. P. Graves
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
B. P. Duval
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
L. Porte
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
H. Reimerdes
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
O. Sauter
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
T.-M. Tran
Affiliation:
Ecole Polytechnique Fédérale de Lausanne, Centre de Recherches en Physique des Plasmas, CH1015 Lausanne, Switzerland
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Novel free boundary magnetohydrodynamic equilibrium states with spontaneous three-dimensional (3-D) deformations of the plasma–vacuum interface are computed. The structures obtained look like saturated ideal external kink/peeling modes. Large edge pressure gradients yield toroidal mode number 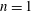$n=1$ distortions when the edge bootstrap current is large and higher $n$ corrugations when this current is small. Linear ideal MHD stability analyses confirm the nonlinear saturated ideal kink equilibrium states produced and we can identify the Pfirsch–Schlüter current as the main linear instability driving mechanism when the edge pressure gradient is large. The dominant non-axisymmetric component of this Pfirsch–Schlüter current drives a near resonant helical parallel current density ribbon that aligns with the near vanishing magnetic shear region caused by the edge bootstrap current. This current ribbon is a manifestation of the outer mode previously found on JET (Solano 2010). We claim that the equilibrium corrugations describe structures that are commonly observed in quiescent H-mode tokamak discharges.

Type
Research Article
Information
Journal of Plasma Physics , Volume 81 , Issue 6 , December 2015 , 515810605
Copyright
© EUROfusion Consortium Research Institutions 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, D. V., Cooper, W. A., Gruber, R., Merazzi, S. & Schwenn, U. 1990 Methods for the efficient calculation of the magnetohydrodynamic (MHD) stability properties of magnetically confined fusion plasmas. Int. J. Supercomp. Appl. 4, 34–47.Google Scholar
Boozer, A. H. 1980 Guiding center drift equations. Phys. Fluids 23, 904–908.Google Scholar
Burrell, K. H., West, W. P., Doyle, E. J., Austin, M. E., Casper, T. A., Gohil, P., Greenfield, C. M., Groebner, R. J., Hyatt, A. W., Jayakumar, R. J. et al. 2005 Advances in understanding quiescent H-mode plasmas in DIII-D. Phys. Plasmas 12, 056121.Google Scholar
Chapman, I. T., Hua, M.-D., Pinches, S., Akers, R. J., Field, A. R., Graves, J. P., Hastie, R. J., Michael, C. A.& the MAST Team 2010 Saturated ideal modes in advanced tokamak regimes in mast. Nucl. Fusion 50, 045007.Google Scholar
Cooper, W. A. 1992 Variational formulation of the linear MHD stability of 3D plasmas with noninteracting hot electrons. Plasma Phys. Control. Fusion 34, 1011–1036.Google Scholar
Cooper, W. A. 1997 Normal curvature, local magnetic shear and parallel current density in tokamaks and torsatrons. Phys. Plasmas 4, 153–161.Google Scholar
Cooper, W. A., Graves, J. P., Pochelon, A., Sauter, O. & Villard, L. 2010 Tokamak magnetohydrodynamic equilibrium states with axisymmetric boundary and a 3D helical core. Phys. Rev. Lett. 105, 035003.Google Scholar
Cooper, W. A., Graves, J. P. & Sauter, O. 2011 JET snake magnetohydrodynamic equilibria. Nucl. Fusion 51, 072002.Google Scholar
Dewar, R. L., Monticello, D. A. & Sy, W. N.-C. 1984 Magnetic coordinates for equilibria with a continuous symmetry. Phys. Fluids 27, 1723–1732.CrossRefGoogle Scholar
Grad, H. & Rubin, H. 1958 Hydromagnetic equilibria and force-free fields. In Proceedings of 2nd UN Conference on the Peaceful Uses of Atomic Energy, vol. 31, p. 190. IAEA.Google Scholar
Greene, J. M. 1996 A new form of magnetohydrodynamic potential energy. Phys. Plasmas 3, 8–9.Google Scholar
Greene, J. M. & Johnson, J. L. 1968 Interchange instabilities in ideal magnetohydrodynamic theory. Phys. Plasmas 10, 729–745.Google Scholar
Hegna, C. C. 2000 Local three-dimensional equilibria. Phys. Plasmas 7, 3921–3928.CrossRefGoogle Scholar
Hirshman, S. P., van Rij, W. I. & Merkel, P. 1986 Three-dimensional free boundary calculations using a spectral green’s function method. Comput. Phys. Commun. 43, 143–154.Google Scholar
Hirshman, S. P. & Whitson, J. C. 1983 Steepest-descent moment method for three-dimensional magnetohydrodynamic equilibria. Phys. Fluids 26, 3553–3568.Google Scholar
Hu, J. S., Sun, Z., Guo, H. Y., Li, J. G., Wan, B. H., Wang, H. Q., Ding, S. Y., Xu, G. S., Liang, Y. F., Mansfield, D. K. et al. 2015 New steady-state quiescent high-confinement plasma in an experimental advanced superconducting tokamak. Phys. Rev. Lett. 114, 055001.Google Scholar
Krebs, I., Hölzl, M., Lackner, K. & Günter, S. 2013 Nonlinear excitation of low-n harmonics in reduced magnetohydrodynamic simulations of edge-localized modes. Phys. Plasmas 20, 082506.Google Scholar
Liu, F., Huijsmans, G. T. A., Loarte, A., Garofalo, A. M., Solomon, W. M., Snyder, P. B., Hoelzl, M. & Zheng, L. 2015 Nonlinear MHD simulations of quiescent H-mode plasmas in DIII-D. Nucl. Fusion 55, 113002.Google Scholar
Shafranov, V. D. 1966 Plasma equilibrium in a magnetic field. In Reviews of Plasma Physics (ed. Leontovich, M. A.), vol. 2, pp. 103–151. Consultants Bureau.Google Scholar
Snyder, P. B., Groebner, R. J., Leonard, A. W., Osborne, T. H. & Wilson, H. R. 2009 Development and validation of a predictive model for the pedestal height. Phys. Plasmas 16, 056118.Google Scholar
Solano, E. R., Lomas, P. J., Alper, B., Xu, G. S., Andrew, Y., Arnoux, G., Boboc, A., Barrera, L., Belo, P., Beurskens, M. N. A. et al. 2010 Observation of confined current ribbon in JET plasmas. Phys. Rev. Lett. 104, 018503.Google Scholar
Solomon, W. M., Snyder, P. B., Burrell, K. H., Fenstermacher, M. E., Garofalo, A. M., Grierson, B. A., Loarte, A., McKee, G. R., Nazikian, R. & Osborne, T. H. 2014 Access to a new plasma edge state with high density and pressures using quiescent H mode. Phys. Rev. Lett. 113, 135001.Google Scholar
Suttrop, W., Hynönen, V., Kurki-Suonio, T., Lang, P. T., Maraschek, M., Neu, R., Stbler, A., Conway, G. D., Hacquin, S., Kempenaars, M. et al. 2005 ‘Quiescent H-mode’ regime in ASDEX Upgrade and JET. Nucl. Fusion 45, 721–730.Google Scholar
Wagner, F., Becker, G., Behringer, K., Campbell, D., Eberhagen, A., Engelhardt, W., Fussmann, G., Gehre, G., Gernhardt, J., v. Gierke, G. et al. 1982 A regime of improved confinement and high beta in neutral beam heated divertor discharges of ASDEX. Phys. Rev. Lett. 49, 1408–1412.CrossRefGoogle Scholar
Wenninger, R. P., Reimerdes, R., Sauter, O. & Zohm, H. 2013 Non-linear magnetic perturbations during edge-localized modes in TCV dominated by low n mode components. Nucl. Fusion 53, 113004.Google Scholar
Zohm, H. 1992 Edge localized modes (ELMS). Plasma Phys. Control. Fusion 38, 105.Google Scholar