No CrossRef data available.
Article contents
The cocked hat: formal statements and proofs of the theorems
Published online by Cambridge University Press: 15 February 2021
Abstract
Navigators have been taught for centuries to estimate the location of their craft on a map from three lines of position, for redundancy. The three lines typically form a triangle, called a cocked hat. How is the location of the craft related to the triangle? For more than 80 years navigators have also been taught that, if each line of position is equally likely to pass to the right and to the left of the true location, then the likelihood that the craft is in the triangle is exactly 1/4. This is stated in numerous reputable sources, but was never stated or proved in a mathematically formal and rigorous fashion. In this paper we prove that the likelihood is indeed 1/4 if we assume that the lines of position always intersect pairwise. We also show that the result does not hold under weaker (and more reasonable) assumptions, and we prove a generalisation to 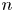 $n$ lines.
$n$ lines.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © The Royal Institute of Navigation 2021



