Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-05T05:08:27.910Z Has data issue: false hasContentIssue false
  • English
  • Français

Original in situ observations of creep during indentation and recovery of the residual imprint on amorphous polymer

Published online by Cambridge University Press:  13 December 2011

Thibaud Chatel ,
Hervé Pelletier ,
Vincent Le Houérou ,
Christian Gauthier ,
Damien Favier  and
Robert Schirrer
Thibaud Chatel
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
Hervé Pelletier*
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
Vincent Le Houérou
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
Christian Gauthier
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
Damien Favier
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
Robert Schirrer
Affiliation:
Institut Charles Sadron, CNRS UPR22, F-67034 Strasbourg Cedex2, France
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

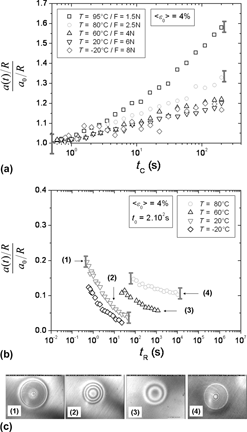
Creep during loading and recovery phases after load removal are studied using a homemade experimental device that allows us to record in situ the evolution of the true contact area and of the residual imprint versus the time. Indentation tests are performed using a spherical indenter with a tip radius R = 400 μm onto amorphous polymeric surface poly(methylmethacrylate) (PMMA) at different contact durations (10–105 s) and controlled temperatures varying between −20 and 100 °C. Original experimental results are presented about the true evolution of the contact area during creep and recovery phases. An interesting experimental parameter, defined by the ratio a(t)/a0, (with a(t), evolution of the contact radius with creep or relaxation time, and a0, the initial value of the contact radius at the end of the loading phase or at the end of the creep phase) has been introduced to describe the evolution of imposed strain during indentation. As a function of the temperature and of the initial average strain imposed at the end of the loading phase, some nonlinear phenomena can be observed. Using two-dimensional axisymmetric finite element modeling, assuming only viscoelastic behavior, creep and recovering phases during indentation have been reproduced. The simulation results indicate that (i) the test is mainly controlled by the imposed strain and not by the contact pressure, and (ii) some plasticity could appear in the contact zone and as a function of the location and the size of the volume where the strain is maximal, the recovery is more or less limited.

Keywords

PolymerViscoelasticityNanoindentation
Type
Reviews
Information
Journal of Materials Research , Volume 27 , Issue 1: Focus Issue: Instrumented Indentation , 14 January 2012 , pp. 12 - 19
Copyright
Copyright © Materials Research Society 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1.Pharr, G.M.: Measurement of mechanical properties by ultra-low-load indentation. Mater. Sci. Eng., A 253, 151 (1998).Google Scholar
2.Oliver, W.C. and Pharr, G.M.: An improved technique for determining hardness and elastic-modulus using load and displacement sensing indentation experiments. J. Mater. Res. 7, 1564 (1992).Google Scholar
3.Oliver, W.C. and Pharr, G.M.: Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 19, 3 (2004).Google Scholar
4.Chudoba, T. and Richter, F.: Investigation of creep behaviour under load during indentation experiments and its influence on hardness and modulus results. Surf. Coat. Tech. 148, 191 (2001).Google Scholar
5.Oyen, M.L. and Cook, R.F.: Load-displacement behavior during sharp indentation of viscous-elastic-plastic materials. J. Mater. Res. 18, 139 (2003).Google Scholar
6.Oyen, M.L.: Spherical indentation creep following ramp loading. J. Mater. Res. 20, 2094 (2005).Google Scholar
7.Fischer-Cripps, A.C.: A simple phenomenological approach to nanoindentation creep. Mater. Sci. Eng., A 385, 74 (2004).Google Scholar
8.Ngan, A.H.W., Wang, H.T., Tang, B., and Sze, K.Y.: Correcting power-law viscoelastic effects in elastic modulus measurement using depth-sensing indentation. Int. J. Solids Struct. 42, 1831 (2005).Google Scholar
9.Goodall, R. and Clyne, T.W.: A critical appraisal of the extraction of creep parameters from nanoindentation data obtained at room temperature. Acta Mater. 54, 5489 (2006).Google Scholar
10.Cheng, L., Xia, X., Scriven, L.E., and Gerberich, W.W.: Spherical-tip indentation of viscoelastic material. Mech. Mater. 37, 213 (2005).Google Scholar
11.Kaufman, J.D. and Klapperich, C.M.: Surface detection errors cause overestimation of the modulus in nanoindentation on soft materials. J. Mech. Behav. Biomed. Mater. 2, 312 (2009).Google Scholar
12.Liao, Q., Huang, J., Zhu, T., Xiong, C., and Fang, J.: A hybrid model to determine mechanical properties of soft polymers by nanoindentation. Mech. Mater. 42, 1043 (2010).CrossRefGoogle Scholar
13.Tweedie, C.A. and Van Vliet, K.J.: On the indentation recovery and fleeting hardness of polymers. J. Mater. Res. 21, 3029 (2006).Google Scholar
14.Oyen, M.L.: Analytical techniques for indentation of viscoelastic materials. Philos. Mag. 86, 5625 (2006).Google Scholar
15.Tabor, D.: The hardness of solids. Rev. Phys. Technol. 1, 145 (1970).CrossRefGoogle Scholar
16.Gauthier, C. and Schirrer, R.: Time and temperature dependence of the scratch properties of poly(methylmethacrylate) surfaces. J. Mater. Sci. 35, 2121 (2000).Google Scholar
17.Gauthier, C., Lafaye, S., and Schirrer, R.: Elastic recovery of a scratch in a polymeric surface: Experiments and analysis. Tribol. Int. 34, 469 (2001).CrossRefGoogle Scholar
18.Chatel, T., Gauthier, C., Pelletier, H., Le Houerou, V., Favier, D., and Schirrer, R.: Creep of the contact with a spherical tip and recovery of the imprint on amorphous polymer surfaces. J Phys D: Appl. Phys. 44, 375403, doi:10.1088/0022-3727/44/37/375403 (2011).Google Scholar
19.Pelletier, H., Gauthier, C., and Schirrer, R.: Experimental measurement and numerical simulation of the plastic strain during indentation and scratch tests on polymeric surfaces. J. Mater. Res. 24, 1184 (2009).Google Scholar
20.Johnson, K.L.: Contact Mechanics (Cambridge University Press, Cambridge, U.K., 1987).Google Scholar
21.Samyn, P., Van Schepdael, L., Leendertz, J.S., Gerber, A., Van Paepegem, W., Degrieck, J., and De Baets, P.: Full-scale analysis of deformation and stress distribution for constrained composite bearing elements under compressive yielding conditions. Mater. Des. 28, 2450 (2007).Google Scholar