Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yang, Fuqian
and
Li, J.C.M.
1997.
Newtonian viscosity measured by impression test.
Journal of Non-Crystalline Solids,
Vol. 212,
Issue. 2-3,
p.
126.
Yang, Fuqian
and
Li, J.C.M.
1997.
Viscosity of selenium measured by impression test.
Journal of Non-Crystalline Solids,
Vol. 212,
Issue. 2-3,
p.
136.
Shao, C.B.
and
Zhang, J.G.
1998.
Mechanical properties and fretting behavior of sulfide film.
p.
34.
Lucas, B. N.
Oliver, W. C.
and
Swindeman, J. E.
1998.
The Dynamics of Frequency-Specific, Depth-Sensing Indentation Testing.
MRS Proceedings,
Vol. 522,
Issue. ,
Shimizu, S.
Yanagimoto, T.
and
Sakai, M.
1999.
Pyramidal indentation load–depth curve of viscoelastic materials.
Journal of Materials Research,
Vol. 14,
Issue. 10,
p.
4075.
Wolf, B.
and
Paufler, P.
1999.
Mechanical Properties of Icosahedral AlPdMn Probed by Indentation at Variable Temperatures.
physica status solidi (a),
Vol. 172,
Issue. 2,
p.
341.
Lucas, B. N.
and
Oliver, W. C.
1999.
Indentation power-law creep of high-purity indium.
Metallurgical and Materials Transactions A,
Vol. 30,
Issue. 3,
p.
601.
Sangwal, K.
Gorostiza, P.
and
Sanz, F.
2000.
Atomic force microscopy study of nanoindentation creep on the (100) face of MgO single crystals.
Surface Science,
Vol. 446,
Issue. 3,
p.
314.
Loubet, J. L.
Oliver, W. C.
and
Lucas, B. N.
2000.
Measurement of the loss tangent of low-density polyethylene with a nanoindentation technique.
Journal of Materials Research,
Vol. 15,
Issue. 5,
p.
1195.
Wolf, B.
2000.
Inference of Mechanical Properties from Instrumented Depth Sensing Indentation at Tiny Loads and Indentation Depths.
Crystal Research and Technology,
Vol. 35,
Issue. 4,
p.
377.
2000.
Mechanical Testing and Evaluation.
p.
232.
Pittenger, B.
Fain, S. C.
Cochran, M. J.
Donev, J. M. K.
Robertson, B. E.
Szuchmacher, A.
and
Overney, R. M.
2001.
Premelting at ice-solid interfaces studied via velocity-dependent indentation with force microscope tips.
Physical Review B,
Vol. 63,
Issue. 13,
Guin, Jean-Pierre
Rouxel, Tanguy
Keryvin, Vincent
Sanglebœuf, Jean-Christophe
Serre, Ingrid
and
Lucas, Jacques
2002.
Indentation creep of Ge–Se chalcogenide glasses below Tg: elastic recovery and non-Newtonian flow.
Journal of Non-Crystalline Solids,
Vol. 298,
Issue. 2-3,
p.
260.
Schuh, C. A.
Nieh, T. G.
and
Kawamura, Y.
2002.
Rate Dependence of Serrated Flow During Nanoindentation of a Bulk Metallic Glass.
Journal of Materials Research,
Vol. 17,
Issue. 7,
p.
1651.
Li, James C.M
2002.
Impression creep and other localized tests.
Materials Science and Engineering: A,
Vol. 322,
Issue. 1-2,
p.
23.
Lafontan, X.
Pressecq, F.
Beaudoin, F.
Rigo, S.
Dardalhon, M.
Roux, J.-L.
Schmitt, P.
Kuchenbecker, J.
Baradat, B.
Lellouchi, D.
Le-Touze, C.
and
Nicot, J.-M.
2003.
The advent of MEMS in space.
Microelectronics Reliability,
Vol. 43,
Issue. 7,
p.
1061.
Schuh, C.A.
and
Nieh, T.G.
2003.
A nanoindentation study of serrated flow in bulk metallic glasses.
Acta Materialia,
Vol. 51,
Issue. 1,
p.
87.
Oliver, W.C.
and
Pharr, G.M.
2004.
Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology.
Journal of Materials Research,
Vol. 19,
Issue. 1,
p.
3.
Alkorta, J.
and
Sevillano, J. Gil
2004.
Measuring the strain rate sensitivity by instrumented indentation. Application to an ultrafine grain (equal channel angular–pressed) eutectic Sn–Bi alloy.
Journal of Materials Research,
Vol. 19,
Issue. 1,
p.
282.
Tang, B.
and
Ngan, A. H. W.
2004.
Investigation of Viscoelastic Properties of Amorphous Selenium near Glass Transition Using Depth‐Sensing Indentation.
Soft Materials,
Vol. 2,
Issue. 2-3,
p.
125.
 , and the effective strain rate occurring during the indentation creep process is obtained. Indentation creep measurements on amorphous selenium in the Newtonian viscous flow regime above the glass transition temperature were obtained. The data were then used to determine that the coefficient relating indentation strain rate to the effective strain rate is equal to 0.09, or
, and the effective strain rate occurring during the indentation creep process is obtained. Indentation creep measurements on amorphous selenium in the Newtonian viscous flow regime above the glass transition temperature were obtained. The data were then used to determine that the coefficient relating indentation strain rate to the effective strain rate is equal to 0.09, or 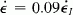 .
.