Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sigmund, O.
1998.
Topology optimization in multiphysics problems.
Calvert, Paul
1998.
Freeforming of polymers.
Current Opinion in Solid State and Materials Science,
Vol. 3,
Issue. 6,
p.
585.
Sigmund, O.
and
Petersson, J.
1998.
Numerical instabilities in topology optimization: A survey on procedures dealing with checkerboards, mesh-dependencies and local minima.
Structural Optimization,
Vol. 16,
Issue. 1,
p.
68.
Gibiansky, L. V.
and
Torquato, S.
1999.
Matrix laminate composites: Realizable approximations for the effective moduli of piezoelectric dispersions.
Journal of Materials Research,
Vol. 14,
Issue. 1,
p.
49.
Pecullan, S.
Gibiansky, L.V.
and
Torquato, S.
1999.
Scale effects on the elastic behavior of periodic andhierarchical two-dimensional composites.
Journal of the Mechanics and Physics of Solids,
Vol. 47,
Issue. 7,
p.
1509.
Telega, J. J.
Gambin, B.
and
Galka, A.
1999.
Smart Structures.
p.
355.
Sigmund, O
and
Torquato, S
1999.
Design of smart composite materials using topology optimization.
Smart Materials and Structures,
Vol. 8,
Issue. 3,
p.
365.
Perry, A
Bowen, C.R
and
Mahon, S.W
1999.
Finite element modelling of 3-3 piezocomposites.
Scripta Materialia,
Vol. 41,
Issue. 9,
p.
1001.
Pedersen, Pauli
1999.
Mechanics of Composite Materials and Structures.
p.
453.
Torquato, S
2000.
Modeling of physical properties of composite materials.
International Journal of Solids and Structures,
Vol. 37,
Issue. 1-2,
p.
411.
Sigmund, O.
2001.
Design of multiphysics actuators using topology optimization – Part I: One-material structures.
Computer Methods in Applied Mechanics and Engineering,
Vol. 190,
Issue. 49-50,
p.
6577.
Glushanin, S V
and
Topolov, V Yu
2001.
Features of electromechanical properties of piezoactive composites with elements of connectivity 1-1.
Journal of Physics D: Applied Physics,
Vol. 34,
Issue. 16,
p.
2518.
Sigmund, Ole
2001.
Mechanics for a New Mellennium.
p.
505.
Adams, B.L.
Henrie, A.
Henrie, B.
Lyon, M.
Kalidindi, S.R.
and
Garmestani, H.
2001.
Microstructure-sensitive design of a compliant beam.
Journal of the Mechanics and Physics of Solids,
Vol. 49,
Issue. 8,
p.
1639.
Calvert, P.
2001.
Encyclopedia of Materials: Science and Technology.
p.
3340.
Sigmund, O.
2001.
Design of multiphysics actuators using topology optimization – Part II: Two-material structures.
Computer Methods in Applied Mechanics and Engineering,
Vol. 190,
Issue. 49-50,
p.
6605.
Pedersen, Claus B. W.
Buhl, Thomas
and
Sigmund, Ole
2001.
Topology synthesis of large‐displacement compliant mechanisms.
International Journal for Numerical Methods in Engineering,
Vol. 50,
Issue. 12,
p.
2683.
Calvert, P.
2001.
Encyclopedia of Materials: Science and Technology.
p.
595.
Topolov, V. Yu.
and
Turik, A. V.
2001.
On increasing the hydrostatic sensitivity of three-component piezocomposites.
Technical Physics Letters,
Vol. 27,
Issue. 1,
p.
81.
Glushanin, S. V.
and
Topolov, V. Yu.
2001.
Anisotropy of the electromechanical properties and a high piezoelectric sensitivity of the 1-1 type ferroelectric piezocomposites.
Technical Physics Letters,
Vol. 27,
Issue. 8,
p.
626.
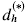 , the hydrophone figure of merit
, the hydrophone figure of merit 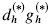 , and the electromechanical coupling factor
, and the electromechanical coupling factor  . The piezocomposite consists of piezoelectric rods embedded in an optimal polymer matrix. We use the topology optimization method to design the optimal (porous) matrix microstructure. When we design for maximum
. The piezocomposite consists of piezoelectric rods embedded in an optimal polymer matrix. We use the topology optimization method to design the optimal (porous) matrix microstructure. When we design for maximum 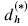 and
and 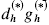 , the optimal transversally isotopic matrix material has negative Poisson's ratio in certain directions. When we design for maximum
, the optimal transversally isotopic matrix material has negative Poisson's ratio in certain directions. When we design for maximum 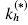 , the optimal matrix microstructure is layered and simple to build.
, the optimal matrix microstructure is layered and simple to build.