Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-25T23:57:40.415Z Has data issue: false hasContentIssue false
  • English
  • Français

Orderings and signatures of higher level on multirings and hyperfields

Part of: Topological fields Forms and linear algebraic groups

Published online by Cambridge University Press:  16 May 2012

Paweł Gładki  and
Murray Marshall
Paweł Gładki
Affiliation:
Institute of Mathematics, University of Silesia, ul. Bankowa 14, Katowice, 40-007, Poland, [email protected]
Murray Marshall
Affiliation:
Department of Mathematics and Statistics, University of Saskatchewan, Saskatoon, SK S7N 5E6, Canada, [email protected]
Get access

Abstract

Multirings are objects like rings but with multi-valued addition. In the present paper we extend results of E. Becker and others concerning orderings of higher level on fields and rings to orderings of higher level on hyperfields and multirings and, in the process of doing this, we establish higher level analogs of the results previously obtained by the second author. In particular, we introduce a class of multirings called ℓ-real reduced multirings, define a natural reflection A ⇝ Qℓ-red(A) from the category of multirings satisfying 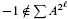to the full subcategory of ℓ-real reduced multirings, and provide an elementary first-order description of these objects. The relationship between ℓ-real reduced hyperfields and the spaces of signatures defined by Mulcahy and Powers is also examined.

Keywords

multiringshypergroupshyperringshyperfieldsorderings of higher levelsignatures of higher level

MSC classification

Secondary: 12J15: Ordered fields 11E10: Forms over real fields
Type
Research Article
Information
Journal of K-Theory , Volume 10 , Issue 3 , December 2012 , pp. 489 - 518
Copyright
Copyright © ISOPP 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Andradas, C., Bröcker, L., Ruiz, J., Constructible sets in real geometry, Springer, 1996.Google Scholar
2.Becker, E., Hereditarily Pythagorean fields and orderings of higher level, IMPA Lecture Notes 29, Rio de Janeiro, 1978.Google Scholar
3.Becker, E., Gondard, D., On rings admitting orderings and 2-primary chains of orderings of higher level, Manuscripta Math. 65 (1989), 63–82.Google Scholar
4.Becker, E., Rosenberg, A., Reduced forms and reduced Witt rings of higher level, J. Algebra 92 (1985), 477–503.Google Scholar
5.Berr, R., The intersection theorem for orderings of higher level in rings, Manuscripta Math. 75 (1992), 273–277.Google Scholar
6.Bochnak, J., Coste, M., Roy, M-F., Géométrie algébrique réelle, Springer, 1987.Google Scholar
7.Connes, A., Consani, C., The hyperring of adèle classes, arXiv:1001.4260.Google Scholar
8.Connes, A., Consani, C., From monoids to hyperstructures: In search of an absolute arithmetic, arXiv:1006.4810v1.Google Scholar
9.Dickmann, M., Miraglia, F., Special groups: Boolean-theoretic methods in the theory of quadratic forms, Memoirs of the AMS 689, Amer. Math. Soc., Providence RI, 2000.Google Scholar
10.Dickmann, M., Miraglia, F., Algebraic K-theory of fields and special groups, Contemp. Math. 235 (1999), 83–98.Google Scholar
11.Dickmann, M., Miraglia, F., Lam's conjecture, Algebra Colloq. 10 (2003), 149–176.Google Scholar
12.Dickmann, M., Miraglia, F., Algebraic K-theory of special groups. J. Pure Appl. Algebra 204 (2006), 195–234.Google Scholar
13.Efrat, I., Valuations, orderings and Milnor K-theory, Math. Surveys and Monographs 124, Amer. Math. Soc., Providence RI, 2006.Google Scholar
14.Gładki, P., Orderings of higher level in multifields and multirings, Ann. Math. Silesianae, 24 (2010), 15–25.Google Scholar
15.Hochster, M., Prime ideal structure in commutative rings, Trans. Amer. Math. Soc. 142 (1969), 43–60.Google Scholar
16.Joly, J-R., Sommes de puissances d-ièmes dans un anneau commutatif, Acta Arith. 17 (1970), 37–114.Google Scholar
17.Krasner, M., Approximation des corps valués complets de caractéristique p ≠ 0 par ceux de caractéristique 0. 1957 Colloque d'algèbre supérieure, tenu à Bruxelles du 19 au 22 décembre 1956 pp. 129–206 Centre Belge de Recherches Mathématiques Établissements Ceuterick, Louvain; Librairie Gauthier-Villars, ParisGoogle Scholar
18.Krasner, M., A class of hyperrings and hyperfields, Internat. J. Math. and Math. Sci. 6 (1983), 307–312.Google Scholar
19.Marshall, M., Classification of finite spaces of orderings, Canad. J. Math. 31 (1979), 320–230.Google Scholar
20.Marshall, M., Spaces of orderings and abstract real spectra, Springer Lecture Notes in Math. 1636 (1996).Google Scholar
21.Marshall, M., The elementary type conjecture in quadratic form theory, Contemp. Math. 144 (2004), 275–293.Google Scholar
22.Marshall, M., Real reduced multirings and multifields, J. Pure and Appl. Alg. 205 (2006), 452–468.Google Scholar
23.Marshall, M., Review of the book Valuations, orderings and Milnor K-theory, Math. Surveys and Monographs 124, Amer. Math. Soc. (2006), by Ido Efrat, Bull. Amer. Math. Soc. 45 (2008), 439–444.Google Scholar
24.Marshall, M., Walter, L., Signatures of higher level on rings with many units. Math. Zeit. 204 (1990), 129–143.Google Scholar
25.Mittas, J., Sur une classe d'hypergroupes commutatifs, C. R. Acad. Sci. Paris Ser. A-B 269 (1969), A485–A488.Google Scholar
26.Mulcahy, C., An abstract approach to higher level forms and rigidity, Comm. Algebra 16 (1988), 477–612.Google Scholar
27.Powers, V., Characterizing reduced Witt rings of higher level, Pacific J. Math. 128 (1987), 333–347.CrossRefGoogle Scholar
28.Powers, V., Finite spaces of signatures, Canad. J. Math. 41 (1989), 808–829.CrossRefGoogle Scholar
29.Viro, O., Hyperfields for Tropical Geometry I. Hyperfields and Dequantization, arXiv:1006.3034v2.Google Scholar
30.Walter, L., Orders and signatures of higher level on a commutative ring, Ph. D. Thesis, University of Saskatchewan, 1994.Google Scholar