Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dhorajia, Alpesh M.
and
Keshari, Manoj K.
2010.
Projective modules over overrings of polynomial rings.
Journal of Algebra,
Vol. 323,
Issue. 2,
p.
551.
Fasel, Jean
2011.
Stably free modules over smooth affine threefolds.
Duke Mathematical Journal,
Vol. 156,
Issue. 1,
Dhorajia, Alpesh M.
and
Keshari, Manoj K.
2012.
A note on cancellation of projective modules.
Journal of Pure and Applied Algebra,
Vol. 216,
Issue. 1,
p.
126.
Gupta, Anjan
Garge, Anuradha
and
Rao, Ravi A.
2014.
A nice group structure on the orbit space of unimodular rows-II.
Journal of Algebra,
Vol. 407,
Issue. ,
p.
201.
Keshari, Manoj K.
and
Lokhande, Swapnil A.
2014.
Projective modules over overrings of polynomial rings and a question of Quillen.
Journal of Pure and Applied Algebra,
Vol. 218,
Issue. 6,
p.
1003.
Gupta, Anjan
2015.
Optimal injective stability for the symplectic K1Sp group.
Journal of Pure and Applied Algebra,
Vol. 219,
Issue. 5,
p.
1336.
Gupta, Anjan
2016.
On the existence of unimodular elements and cancellation of projective modules over noetherian and non-noetherian rings.
Journal of Algebra,
Vol. 446,
Issue. ,
p.
323.
Keshari, Manoj K.
and
Sharma, Sampat
2022.
Nice group structure on the elementary orbit space of unimodular rows.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 4,
p.
106889.
Chakraborty, Kuntal
and
Rao, Ravi A.
2022.
On a theorem of Keune.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 4,
p.
106874.
Chakraborty, Kuntal
2023.
A note on relative Vaserstein symbol.
Journal of Algebra and Its Applications,
Vol. 22,
Issue. 10,
Keshari, Manoj K.
and
Sharma, Sampat
2024.
Applications of Swan's Bertini theorem to unimodular rows.
Journal of Pure and Applied Algebra,
Vol. 228,
Issue. 2,
p.
107487.
Chakraborty, Kuntal
and
Sharma, Sampat
2024.
Relative unimodular rows over polynomial rings.
Journal of Pure and Applied Algebra,
Vol. 228,
Issue. 10,
p.
107687.
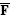 p, then P is cancellative.
p, then P is cancellative.