Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-26T00:12:38.106Z Has data issue: false hasContentIssue false
  • English
  • Français

Hochster's theta pairing and numerical equivalence

Published online by Cambridge University Press:  28 July 2014

Hailong Dao  and
Kazuhiko Kurano
Hailong Dao
Affiliation:
Department of Mathematics, University of Kansas, Lawrence, KS 66045-7523, [email protected]
Kazuhiko Kurano
Affiliation:
Department of Mathematics, School of Science and Technology, Meiji University, Higashimata 1-1-1, Tama-ku, Kawasaki-shi 214-8571, [email protected]
Get access

Abstract

Let (A, 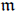) be a local hypersurface with an isolated singularity. We show that Hochster's theta pairing θA vanishes on elements that are numerically equivalent to zero in the Grothendieck group of A under the mild assumption that Spec A admits a resolution of singularities. This extends a result by Celikbas-Walker. We also prove that when dimA = 3, Hochster's theta pairing is positive semi-definite. These results combine to show that the counter-example of Dutta-Hochster-McLaughlin to the general vanishing of Serre's intersection multiplicity exists for any three dimensional isolated hypersurface singularity that is not a UFD and has a desingularization. We also show that, if A is three dimensional isolated hypersurface singularity that has a desingularization, the divisor class group is finitely generated torsion-free. Our method involves showing that θA gives a bivariant class for the morphism Spec (A/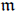) → Spec A.

Keywords

local hypersurfaceisolated singularityHochster's theta invariantintersection multiplicitydivisor class groupGrothendieck groupnumerical equivalences13D0713D1513D2214C1714C35
Type
Research Article
Information
Journal of K-Theory , Volume 14 , Issue 3 , December 2014 , pp. 495 - 525
Copyright
Copyright © ISOPP 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Bourbaki, N., Diviseurs, in “Algèbre Commutative”, Hermann, Paris, 1965.Google Scholar
2.Buchweitz, R-.O, van Straten, D., An Index Theorem for Modules on a Hypersurface Singularity, Mosc. Math. J. 12 (2013), 2823–2844.Google Scholar
3.Celikbas, O., Walker, M., Hochster's theta pairing and Algebraic equivalence, Math. Annalen 353(2) (2012), 359–372.Google Scholar
4.Chan, C.-Y. J., Filtrations of modules, the Chow group, and the Grothendieck group, J. Algebra 219 (1999), 330–344.Google Scholar
5.Dao, H., Some observations on local and projective hypersurfaces, Math. Res. Let. 15(2) (2008), 207–219.Google Scholar
6.Dao, H., Remarks on non-commutative crepant resolutions, Adv. in Math. 224 (2010), 1021–1030.Google Scholar
7.Dao, H., Picard groups of punctured spectra of dimension three local hypersurfaces are torsion-free, Compositio Math. 148 (2012), 145–152.Google Scholar
8.Dao, H., Decency and rigidity for modules over local hypersurfaces, Transactions of the AMS 365(6) (2013), 2803–2821.Google Scholar
9.Deligne, P., Cohomologie des intersections completes, Sem. Geom. Alg. du Bois Marie (SGA 7, II), Springer Lect. Notes Math. 340, (1973).Google Scholar
10.Eisenbud, D., Homological algebra on a complete intersection, with an application to grouprepresentations, Tran. Amer. Math. Soc. 260 (1980), 35–64.Google Scholar
11.Fulton, W., Intersection Theory, 2ndEdition, Springer-Verlag, Berlin, New York, 1997.Google Scholar
12.Gabber, O., On purity for the Brauer group, Arithmetic Algebraic Geometry, Oberwolfach Report No. 34 (2004), 1975–1977.Google Scholar
13.Grothendieck, A., Cohomologie locale des faisceaux cohérents et théorèms de Lefschetz locaux et globaux, Séminaire de Géométrie Algébrique (SGA), North Holland, Amsterdam (1968).Google Scholar
14.Hartshorne, R., Algebraic Geometry, Graduate Texts in Math. 52, Springer-Verlag, Berlin and New York, 1977.Google Scholar
15.Hartshorne, R., Coherent functors, Adv. in Math. 140 (1994), 44–94.Google Scholar
16.Hochster, M., The dimension of an intersection in an ambient hypersurface, Proceedings of the First Midwest Algebraic Geometry Seminar (Chicago Circle, 1980), Lecture Notes in Mathematics 862, Springer-Verlag, 1981, 93–106.Google Scholar
17.Huneke, C., Wiegand, R., Tensor products of modules and the rigidity of Tor, Math. Ann. 299 (1994), 449–476.Google Scholar
18.Huneke, C., Wiegand, R., Tensor products of modules, rigidity and local cohomology, Math. Scan. 81 (1997), 161–183.Google Scholar
19.Huneke, C., Wiegand, R., Jorgensen, D., Vanishing theorems for complete intersections, J. Algebra 238 (2001), 684–702.Google Scholar
20.Jorgensen, D., Finite projective dimension and the vanishing of Ext (M, M), Comm. Alg. 36(12) (2008), 4461–4471.Google Scholar
21.Jothilingam, P., Anote on grade, Nagoya Math. J. 59 (1975), 149–152Google Scholar
22.Kleiman, S., The standard conjectures, Motives (Seattle, WA, 1991) Proc. Sympos. Pure Math. 55, Amer. Math. Soc., Providence, RI, 1994, pp. 3–20.Google Scholar
23.Kurano, K., Numerical equivalence defined on Chow groups of Noetherian local rings, Invent. Math. 157 (2004), 575–619.Google Scholar
24.Lewis, J. D., A survey of the Hodge conjecture, 2ndedition, CRM mono. ser. 10, Amer. Math. Soc., Providence, RI, 1999.Google Scholar
25.Lichtenbaum, S., On the vanishing of Tor in regular local rings, Illinois J. Math. 10 (1966), 220–226.Google Scholar
26.Lütkebohmert, W., On compactification of schemes, Manuscripta Math. 80 (1993), 95–111.Google Scholar
27.Matsumura, H., Commutative Ring Theory, Cambridge Studies in Advanced Mathematics 8, Cambridge university Press, Cambridge (1986).Google Scholar
28.Miller, C., Complexity of Tensor Products of Modules and a Theorem of Huneke-Wiegand, Proc. Amer. Math. Soc. 126 (1998), 53–60.Google Scholar
29.Moore, F., Piepmeyer, G., Spiroff, S., Walker, M., Hochster's theta invariant and the Hodge-Riemann bilinear relations, Adv. in Math. 226(2) (2010), 1692–1714.Google Scholar
30.Nagata, M., A generalization of the imbedding problem of an abstract variety in a complete variety, J. Math. Kyoto Univ. 3 (1963), 89–102.Google Scholar
31.Polishchuk, A., Vaintrob, A., Chern characters and Hirzebruch-Riemann-Roch formula for matrix factorizations, Duke Math J. 161 (2012), 1863–1926.Google Scholar
32.Robbiano, L., Some properties of complete intersections in good projective varieties, Nagoya Math. J. 61 (1976), 103–111.Google Scholar
33.Roberts, P., Multiplicities and Chern classes in Local Algebra, Cambridge Univ. Press, Cambridge (1998).Google Scholar
34.Roberts, P. C. and Srinivas, V., Modules of finite length and finite projective dimension, Invent. Math. 151 (2003), 1–27.Google Scholar
35.Thomason, R. W. and Trobaugh, T., Higher algebraic K-theory of schemes and of derived categories. “The Grothendieck Festschrift”, Vol. III, 247–435, Progr. Math. 88, Birkhauser Boston, Boston, MA, 1990.Google Scholar