Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ding, Zijing
and
Kerswell, Rich R.
2020.
Exhausting the background approach for bounding the heat transport in Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 889,
Issue. ,
Olson, Matthew L.
Goluskin, David
Schultz, William W.
and
Doering, Charles R.
2021.
Heat transport bounds for a truncated model of Rayleigh–Bénard convection via polynomial optimization.
Physica D: Nonlinear Phenomena,
Vol. 415,
Issue. ,
p.
132748.
Motoki, Shingo
Kawahara, Genta
and
Shimizu, Masaki
2021.
Multi-scale steady solution for Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 914,
Issue. ,
Brethouwer, Geert
2021.
Much faster heat/mass than momentum transport in rotating Couette flows.
Journal of Fluid Mechanics,
Vol. 912,
Issue. ,
Mandre, Shreyas
2022.
Brachistochronous motion of a flat plate parallel to its surface immersed in a fluid.
Journal of Fluid Mechanics,
Vol. 939,
Issue. ,
Kumar, Anuj
2022.
Geometrical dependence of optimal bounds in Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Kumar, Anuj
Arslan, Ali
Fantuzzi, Giovanni
Craske, John
and
Wynn, Andrew
2022.
Analytical bounds on the heat transport in internally heated convection.
Journal of Fluid Mechanics,
Vol. 938,
Issue. ,
Tobasco, Ian
2022.
Optimal cooling of an internally heated disc.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 380,
Issue. 2225,
Wen, Baole
Ding, Zijing
Chini, Gregory P.
and
Kerswell, Rich R.
2022.
Heat transport in Rayleigh–Bénard convection with linear marginality.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 380,
Issue. 2225,
Song, Binglin
Fantuzzi, Giovanni
and
Tobasco, Ian
2023.
Bounds on heat transfer by incompressible flows between balanced sources and sinks.
Physica D: Nonlinear Phenomena,
Vol. 444,
Issue. ,
p.
133591.
Alben, Silas
2023.
Transition to branching flows in optimal planar convection.
Physical Review Fluids,
Vol. 8,
Issue. 7,
Alben, Silas
2024.
Optimal wall shapes and flows for steady planar convection.
Journal of Fluid Mechanics,
Vol. 984,
Issue. ,
Ding, Zijing
Huang, Ruiqi
and
Ouyang, Zhen
2024.
Scaling Laws Behind Penetrative Turbulence: History and Perspectives.
Advances in Atmospheric Sciences,
Vol. 41,
Issue. 10,
p.
1881.
Kumar, Anuj
2024.
Three dimensional branching pipe flows for optimal scalar transport between walls.
Nonlinearity,
Vol. 37,
Issue. 11,
p.
115011.
Alben, Silas
Prabala, Shivani
and
Godek, Mitchell
2024.
Enhancing heat transfer in a channel with unsteady flow perturbations.
Physical Review Fluids,
Vol. 9,
Issue. 12,
Lohse, Detlef
and
Shishkina, Olga
2024.
Ultimate Rayleigh-Bénard turbulence.
Reviews of Modern Physics,
Vol. 96,
Issue. 3,
 $Pe$ proportional to their root-mean-square rate of strain, the schemes are applied to compute two-dimensional flows optimizing convective enhancement of diffusive heat transfer, i.e. the Nusselt number
$Pe$ proportional to their root-mean-square rate of strain, the schemes are applied to compute two-dimensional flows optimizing convective enhancement of diffusive heat transfer, i.e. the Nusselt number  $Nu$ up to
$Nu$ up to  $Pe\approx 10^{5}$. The resulting transport exhibits a change of scaling from
$Pe\approx 10^{5}$. The resulting transport exhibits a change of scaling from  $Nu-1\sim Pe^{2}$ for
$Nu-1\sim Pe^{2}$ for  $Pe<10$ in the linear regime to
$Pe<10$ in the linear regime to  $Nu\sim Pe^{0.54}$ for
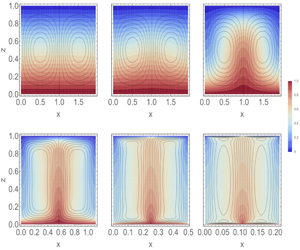
$Nu\sim Pe^{0.54}$ for  $Pe>10^{3}$. Optimal fields are observed to be approximately separable, i.e. products of functions of the wall-parallel and wall-normal coordinates. Analysis employing a separable ansatz yields a conditional upper bound
$Pe>10^{3}$. Optimal fields are observed to be approximately separable, i.e. products of functions of the wall-parallel and wall-normal coordinates. Analysis employing a separable ansatz yields a conditional upper bound  ${\lesssim}Pe^{6/11}=Pe^{0.\overline{54}}$ as
${\lesssim}Pe^{6/11}=Pe^{0.\overline{54}}$ as  $Pe\rightarrow \infty$ similar to the computationally achieved scaling. Implications for heat transfer in buoyancy-driven Rayleigh–Bénard convection are discussed.
$Pe\rightarrow \infty$ similar to the computationally achieved scaling. Implications for heat transfer in buoyancy-driven Rayleigh–Bénard convection are discussed.

