Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-26T18:38:48.011Z Has data issue: false hasContentIssue false
  • English
  • Français

Wall friction and velocity measurements in a double-frequency pulsating turbulent flow

Published online by Cambridge University Press:  08 January 2016

L. R. Joel Sundstrom ,
Berhanu G. Mulu  and
Michel J. Cervantes
L. R. Joel Sundstrom*
Affiliation:
Division of Fluid and Experimental Mechanics, Luleå University of Technology, SE-971 87, Luleå, Sweden
Berhanu G. Mulu
Affiliation:
Vattenfall Research and Development, SE-814 70, Älvkarleby, Sweden
Michel J. Cervantes
Affiliation:
Division of Fluid and Experimental Mechanics, Luleå University of Technology, SE-971 87, Luleå, Sweden Department of Energy and Process Engineering, Norwegian University of Science and Technology, NO-7491, Trondheim, Norway
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Wall shear stress measurements employing a hot-film sensor along with laser Doppler velocimetry measurements of the axial and tangential velocity and turbulence profiles in a pulsating turbulent pipe flow are presented. Time-mean and phase-averaged results are derived from measurements performed at pulsation frequencies 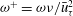${\it\omega}^{+}={\it\omega}{\it\nu}/\bar{u}_{{\it\tau}}^{2}$ over the range of 0.003–0.03, covering the low-frequency, intermediate and quasi-laminar regimes. In addition to the base case of a single pulsation imposed on the mean flow, the study also investigates the flow response when two pulsations are superimposed simultaneously. The measurements from the base case show that, when the pulsation belongs to the quasi-laminar regime, the oscillating flow tends towards a laminar state in which the velocity approaches the purely viscous Stokes solution with a low level of turbulence. For 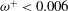${\it\omega}^{+}<0.006$, the oscillating flow is turbulent and exhibits a region with a logarithmic velocity distribution and a collapse of the turbulence intensities, similar to the time-averaged counterparts. In the low-frequency regime, the oscillating wall shear stress is shown to be directly proportional to the Stokes length normalized in wall units 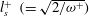$l_{s}^{+}~(=\sqrt{2/{\it\omega}^{+}})$, as predicted by quasi-steady theory. The base case measurements are used as a reference when evaluating the data from the double-frequency case and the oscillating quantities are shown to be close to superpositions from the base case. The previously established view that the time-averaged quantities are unaffected by the imposition of small-amplitude pulsed unsteadiness is shown to hold also when two pulsations are superposed on the mean flow.

JFM classification

Boundary Layers: Pipe flow boundary layer Turbulent Flows: Shear layer turbulence Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 788 , 10 February 2016 , pp. 521 - 548
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abrams, J. & Hanratty, T. J. 1985 Relaxation effects observed for turbulent flow over a wavy surface. J. Fluid Mech. 151, 443–455.CrossRefGoogle Scholar
Alfredsson, P. H., Johansson, A. V., Haritonidis, J. H. & Eckelman, H. 1988 The fluctuating wall shear stress and the velocity field in the viscous sublayer. Phys. Fluids 31, 1026–1033.Google Scholar
Andersson, U.2009 An experimental study of the flow in a sharp-heel Kaplan draft tube. PhD thesis, Luleå University of Technology.Google Scholar
Ariyaratne, C., He, S. & Vardy, A. E. 2010 Wall friction and turbulence dynamics in decelerating pipe flows. J. Hydraul. Res. 48, 810–821.Google Scholar
Binder, G., Tardu, S. & Vezin, P. 1995 Cyclic modulation of Reynolds stresses and length scales in pulsed turbulent channel flow. Proc. R. Soc. Lond. 451, 121–139.Google Scholar
Brereton, G. J., Reynolds, W. C. & Jayaraman, R. 1990 Response of a turbulent boundary layer to sinusoidal free-stream unsteadiness. J. Fluid Mech. 221, 131–159.CrossRefGoogle Scholar
Cervantes, M. J. & Engstrom, T. F. 2008 Pulsating turbulent flow in a straight asymmetric diffuser. J. Hydraul. Res. 46, 112–128.Google Scholar
Cimarelli, A., Frohnapfel, B., Hasegawa, Y., De Angelis, E. & Quadrio, M. 2013 Prediction of turbulence control for arbitrary periodic spanwise wall movement. Phys. Fluids 25, 075102.Google Scholar
Ciocan, G. D. & Avellan, F. 2002 Wall friction measurements: application in a Francis turbine cone. In US ASME–European Fluids Engineering Summer Conference, Montreal, Canada, pp. 317–322.Google Scholar
Coleman, H. W. & Steele, W. G. 2009 Experimentation, Validation and Uncertainty Analysis for Engineers, 3rd edn. John Wiley & Sons.Google Scholar
Durst, F., Jovanovic, J. & Sender, J. 1995 LDA measurements in the near-wall region of a turbulent pipe flow. J. Fluid Mech. 295, 305–335.Google Scholar
Durst, F., Martinuzzi, R., Sender, J. & Thevenin, D. 1992 LDA-measurements of mean velocity, RMS-values and higher order moments of turbulence intensity fluctuations in flow fields with strong velocity gradients. In Proceedings of the 6th International Symposium on LDA, Lisbon, Portugal, pp. 5.1.1–5.1.6.Google Scholar
Eggels, J. G. M., Unger, F., Weiss, M. H., Westerweel, J., Adrian, R. J., Friedrich, R. & Nieuwstadt, F. T. M. 1994 Fully developed turbulent pipe flow: a comparison between direct numerical simulation and experiment. J. Fluid Mech. 268, 175–210.Google Scholar
El Khoury, G. K., Schlatter, P., Noorani, A., Fischer, P. F., Brethouwer, G. & Johansson, A. V. 2013 Direct numerical simulation of turbulent pipe flow at moderately high Reynolds numbers. Flow Turbul. Combust. 91, 475–495.CrossRefGoogle Scholar
Finnicum, D. S. & Hanratty, T. J. 1988 Influence of imposed flow oscillations on turbulence. Physio-Chem. Hydrodyn. 10, 585–598.Google Scholar
Goldstein, R. J. 1996 Fluid Mechanics Measurements, 2nd edn. Taylor & Francis.Google Scholar
He, S., Ariyaratne, C. & Vardy, A. E. 2008 A computational study of wall friction and turbulence dynamics in accelerating pipe flows. Comput. Fluids 37, 674–689.Google Scholar
He, S., Ariyaratne, C. & Vardy, A. E. 2011 Wall shear stress in accelerating turbulent pipe flow. J. Fluid Mech. 685, 440–460.Google Scholar
He, S. & Jackson, J. D. 2000 A study of turbulence under conditions of transient flow in a pipe. J. Fluid Mech. 408, 1–38.Google Scholar
He, S. & Jackson, J. D. 2009 An experimental study of pulsating turbulent flow in a pipe. Eur. J. Mech. (B/Fluids) 28, 309–320.CrossRefGoogle Scholar
He, S. & Seddighi, M. 2013 Turbulence in transient channel flow. J. Fluid Mech. 715, 60–102.CrossRefGoogle Scholar
Ismael, J. O. & Cotton, M. A. 1996 Calculations of wall shear stress in harmonically oscillated turbulent pipe flow using a low-Reynolds-number $k$ – ${\it\varepsilon}$ model. J. Fluids Engng 118, 189–194.Google Scholar
Lien, K., Monty, J. P., Chong, M. S. & Ooi, A. 2004 The entrance length for fully developed turbulent channel flow. In The 15th Australasian Fluid Mechanics Conference, Sydney, Australia.Google Scholar
Lodahl, C. R., Sumer, B. M. & Fredsoe, J. 1998 Turbulent combined oscillatory flow and current in a pipe. J. Fluid Mech. 373, 313–348.Google Scholar
Manna, M., Vacca, A. & Verzicco, R. 2012 Pulsating pipe flow with large-amplitude oscillations in the very high frequency regime. Part 1. Time-averaged analysis. J. Fluid Mech. 700, 246–282.Google Scholar
Manna, M., Vacca, A. & Verzicco, R. 2015 Pulsating pipe flow with large-amplitude oscillations in the very high frequency regime. Part 2. Phase-averaged analysis. J. Fluid Mech. 766, 272–296.Google Scholar
Mao, Z. & Hanratty, T. J. 1986 Studies of the wall shear stress in a turbulent pulsating pipe flow. J. Fluid Mech. 170, 545–564.Google Scholar
Mulu, B. G.2012 An experimental and numerical investigation of a Kaplan turbine model. PhD thesis, Luleå University of Technology.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Ronneberger, D. & Ahrens, C. D. 1977 Wall shear stress caused by small amplitude perturbations of turbulent boundary-layer flow: an experimental investigation. J. Fluid Mech. 83, 433–464.Google Scholar
Scotti, A. & Piomelli, U. 2001 Numerical simulation of pulsating turbulent channel flow. Phys. Fluids 13, 1367–1384.Google Scholar
Seddighi, M., He, S., Orlandi, P. & Vardy, A. 2011 A comparative study of turbulence in ramp-up and ramp-down unsteady flows. Flow Turbul. Combust. 86, 439–454.CrossRefGoogle Scholar
Seddighi, M., He, S., Vardy, A. & Orlandi, P. 2014 Direct numerical simulation of an accelerating channel flow. Flow Turbul. Combust. 92, 473–502.Google Scholar
Tardu, S. F. & Binder, G. 1993 Wall shear stress modulation in unsteady turbulent channel flow with high imposed frequencies. Phys. Fluids 5, 2028–2037.CrossRefGoogle Scholar
Tardu, S. F., Binder, G. & Blackwelder, R. F. 1994 Turbulent channel flow with large-amplitude velocity oscillations. J. Fluid Mech. 267, 109–151.Google Scholar
Telionis, D. P. 1981 Unsteady Viscous Flows. Springer.CrossRefGoogle Scholar
Touber, E. & Leschziner, M. A. 2012 Near-wall streak modification by spanwise oscillatory wall motion and drag-reduction mechanics. J. Fluid Mech. 693, 150–200.CrossRefGoogle Scholar
Tu, S. W. & Ramaprian, B. R. 1983 Fully developed periodic turbulent pipe flow. Part 1. Main experimental results and comparison with predictions. J. Fluid Mech. 137, 31–58.Google Scholar
Uchida, S. 1956 The pulsating viscous flow superposed on the steady laminar motion of incompressible fluid in a circular pipe. Z. Angew. Math. Phys., J. Appl. Math. Phys. 7, 403–422.Google Scholar
Zagarola, M. V. & Smits, A. J. 1998 Mean-flow scaling of turbulent pipe flow. J. Fluid Mech. 373, 33–79.Google Scholar