Article contents
Wakes behind a prolate spheroid in crossflow
Published online by Cambridge University Press: 18 May 2012
Abstract
Viscous laminar flow past a prolate 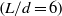 spheroid has been investigated numerically at seven different Reynolds numbers;
spheroid has been investigated numerically at seven different Reynolds numbers;  and
and 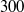 . In contrast to all earlier investigations, the major axis of the spheroid was oriented perpendicular to the free stream flow. As expected, the flow field in the wake showed a strong resemblance of that observed behind a finite-length circular cylinder, yet had features observed in the axisymmetric wake behind a sphere. The following different flow regimes were observed in the present computational study: (i) steady laminar flow with massive flow separation and symmetry about the equatorial and the meridional planes at
. In contrast to all earlier investigations, the major axis of the spheroid was oriented perpendicular to the free stream flow. As expected, the flow field in the wake showed a strong resemblance of that observed behind a finite-length circular cylinder, yet had features observed in the axisymmetric wake behind a sphere. The following different flow regimes were observed in the present computational study: (i) steady laminar flow with massive flow separation and symmetry about the equatorial and the meridional planes at 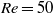 ; (ii) steady laminar flow with massive flow separation and symmetry about the equatorial and the meridional plane at
; (ii) steady laminar flow with massive flow separation and symmetry about the equatorial and the meridional plane at 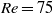 , but the flow in the equatorial plane did no longer resemble the steady wake behind a circular cylinder; (iii) unsteady laminar flow with Strouhal number
, but the flow in the equatorial plane did no longer resemble the steady wake behind a circular cylinder; (iii) unsteady laminar flow with Strouhal number  and symmetry about the equatorial plane at
and symmetry about the equatorial plane at 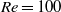 ; (iv) unsteady laminar flow with two distinct frequencies and without any planar symmetries at
; (iv) unsteady laminar flow with two distinct frequencies and without any planar symmetries at  ; (v) transitional flow with a dominant shedding frequency
; (v) transitional flow with a dominant shedding frequency 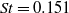 and without any spatial symmetries at
and without any spatial symmetries at 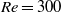 . For all but the two lowest
. For all but the two lowest 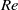 hairpin vortices were alternately shed from the two sides of the spheroid and resulted in a ladder-like pattern of oppositely oriented vortex structures, in contrast with the single-sided shedding in the wake of a sphere. The contour of the very-near-wake mimicked the shape of the prolate spheroid. However,
hairpin vortices were alternately shed from the two sides of the spheroid and resulted in a ladder-like pattern of oppositely oriented vortex structures, in contrast with the single-sided shedding in the wake of a sphere. The contour of the very-near-wake mimicked the shape of the prolate spheroid. However,  downstream the major axis of the wake became aligned with the minor axis of the spheroid. This implies that an axis switching occurred some
downstream the major axis of the wake became aligned with the minor axis of the spheroid. This implies that an axis switching occurred some 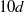 downstream, i.e. the cross-section of the wake evolved such that the major and minor axes interchanged at a certain downstream location. This peculiar phenomenon has frequently been reported to arise for elliptical and rectangular jets, whereas observations of axis switching for asymmetric wakes are scarce.
downstream, i.e. the cross-section of the wake evolved such that the major and minor axes interchanged at a certain downstream location. This peculiar phenomenon has frequently been reported to arise for elliptical and rectangular jets, whereas observations of axis switching for asymmetric wakes are scarce.
JFM classification
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012
References
- 24
- Cited by


