Published online by Cambridge University Press: 03 August 2016
The Rayleigh–Taylor instability induced turbulence is studied under the Boussinesq approximation focusing on vorticity and mixing. A direct numerical simulation has been carried out with an auto-adaptive multidomain Chebyshev–Fourier–Fourier numerical method. The spatial resolution is increased up to 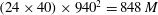 $(24\times 40)\times 940^{2}=848\,M$ collocation points. The Taylor Reynolds number is
$(24\times 40)\times 940^{2}=848\,M$ collocation points. The Taylor Reynolds number is 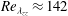 $\mathit{Re}_{\unicode[STIX]{x1D706}_{zz}}\approx 142$ and a short inertial range is observed. The nonlinear growth rate of the turbulent mixing layer is found to be close to
$\mathit{Re}_{\unicode[STIX]{x1D706}_{zz}}\approx 142$ and a short inertial range is observed. The nonlinear growth rate of the turbulent mixing layer is found to be close to 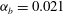 $\unicode[STIX]{x1D6FC}_{b}=0.021$ . Our conclusions may be summarized as follows.
$\unicode[STIX]{x1D6FC}_{b}=0.021$ . Our conclusions may be summarized as follows.
(i) The simulation data are in agreement with the scalings for the pressure ( 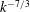 $k^{-7/3}$ ) and the vertical mass flux (
$k^{-7/3}$ ) and the vertical mass flux ( 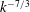 $k^{-7/3}$ ).
$k^{-7/3}$ ).
(ii) Mean quantities have a self-similar behaviour, but some inhomogeneity is still present. For higher-order quantities the self-similar regime is not fully achieved.
(iii) In the self-similar regime, the mean dissipation rate and the enstrophy behave as 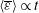 $\langle \overline{\unicode[STIX]{x1D700}}\rangle \propto t$ and
$\langle \overline{\unicode[STIX]{x1D700}}\rangle \propto t$ and 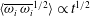 $\langle \overline{\unicode[STIX]{x1D714}_{i}\,\unicode[STIX]{x1D714}_{i}}^{1/2}\rangle \propto t^{1/2}$ , respectively.
$\langle \overline{\unicode[STIX]{x1D714}_{i}\,\unicode[STIX]{x1D714}_{i}}^{1/2}\rangle \propto t^{1/2}$ , respectively.
(iv) The large-scale velocity fluctuation probability density function (PDF) is Gaussian, while vorticity and dissipation PDFs show large departures from Gaussianity.
(v) The pressure PDF exhibits strong departures from Gaussianity and is skewed. This is related to vortex coherent structures.
(vi) The intermediate scales of the mixing are isotropic, while small scales remain anisotropic. This leaves open the possibility of a small-scale buoyancy. Velocity intermediate scales are also isotropic, while small scales remain anisotropic. Mixing and dynamics are therefore consistent.
(vii) Properties and behaviours of vorticity and enstrophy are detailed. In particular, equations for these quantities are written down under the Boussinesq approximation.
(viii) The concentration PDF is quasi-Gaussian. The vertical concentration gradient is both non-Gaussian and strongly skewed.