Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T06:04:38.336Z Has data issue: false hasContentIssue false
  • English
  • Français

Vortex-induced vibrations of a cylinder in planar shear flow

Published online by Cambridge University Press:  20 July 2017

Simon Gsell ,
Rémi Bourguet Open the ORCID record for Rémi Bourguet [Opens in a new window]  and
Marianna Braza
Simon Gsell
Affiliation:
Institut de Mécanique des Fluides de Toulouse, UMR 5502 CNRS-INPT-UPS, Allée du Professeur Camille Soula, 31400 Toulouse, France
Rémi Bourguet*
Affiliation:
Institut de Mécanique des Fluides de Toulouse, UMR 5502 CNRS-INPT-UPS, Allée du Professeur Camille Soula, 31400 Toulouse, France
Marianna Braza
Affiliation:
Institut de Mécanique des Fluides de Toulouse, UMR 5502 CNRS-INPT-UPS, Allée du Professeur Camille Soula, 31400 Toulouse, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The system composed of a circular cylinder, either fixed or elastically mounted, and immersed in a current linearly sheared in the cross-flow direction, is investigated via numerical simulations. 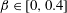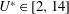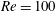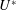The impact of the shear and associated symmetry breaking are explored over wide ranges of values of the shear parameter (non-dimensional inflow velocity gradient, $\unicode[STIX]{x1D6FD}\in [0,0.4]$) and reduced velocity (inverse of the non-dimensional natural frequency of the oscillator, $U^{\ast }\in [2,14]$), at Reynolds number $Re=100$; $\unicode[STIX]{x1D6FD}$, $U^{\ast }$ and 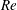$Re$ are based on the inflow velocity at the centre of the body and on its diameter. In the absence of large-amplitude vibrations and in the fixed body case, three successive regimes are identified. Two unsteady flow regimes develop for 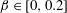$\unicode[STIX]{x1D6FD}\in [0,0.2]$ (regime L) and 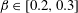$\unicode[STIX]{x1D6FD}\in [0.2,0.3]$ (regime H). They differ by the relative influence of the shear, which is found to be limited in regime L. In contrast, the shear leads to a major reconfiguration of the wake (e.g. asymmetric pattern, lower vortex shedding frequency, synchronized oscillation of the saddle point) and a substantial alteration of the fluid forcing in regime H. A steady flow regime (S), characterized by a triangular wake pattern, is uncovered for $\unicode[STIX]{x1D6FD}>0.3$. Free vibrations of large amplitudes arise in a region of the parameter space that encompasses the entire range of 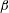$\unicode[STIX]{x1D6FD}$ and a range of 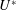$U^{\ast }$ that widens as 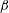$\unicode[STIX]{x1D6FD}$ increases; therefore vibrations appear beyond the limit of steady flow in the fixed body case (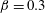$\unicode[STIX]{x1D6FD}=0.3$). Three distinct regimes of the flow–structure system are encountered in this region. In all regimes, body motion and flow unsteadiness are synchronized (lock-in condition). For 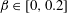$\unicode[STIX]{x1D6FD}\in [0,0.2]$, in regime VL, the system behaviour remains close to that observed in uniform current. The main impact of the shear concerns the amplification of the in-line response and the transition from figure-eight to ellipsoidal orbits. For 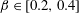$\unicode[STIX]{x1D6FD}\in [0.2,0.4]$, the system exhibits two well-defined regimes: VH1 and VH2 in the lower and higher ranges of 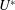$U^{\ast }$, respectively. Even if the wake patterns, close to the asymmetric pattern observed in regime H, are comparable in both regimes, the properties of the vibrations and fluid forces clearly depart. The responses differ by their spectral contents, i.e. sinusoidal versus multi-harmonic, and their amplitudes are much larger in regime VH1, where the in-line responses reach 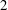$2$ diameters (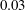$0.03$ diameters in uniform flow) and the cross-flow responses 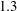$1.3$ diameters. Aperiodic, intermittent oscillations are found to occur in the transition region between regimes VH1 and VH2; it appears that wake–body synchronization persists in this case.

JFM classification

Wakes/Jets: Vortex streets Wakes/Jets: Wakes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 825 , 25 August 2017 , pp. 353 - 384
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. 2000 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Bearman, P. W. 1984 Vortex shedding from oscillating bluff bodies. Annu. Rev. Fluid Mech. 16 (1), 195–222.Google Scholar
Blackburn, H. M., Govardhan, R. N. & Williamson, C. H. K. 2001 A complementary numerical and physical investigation of vortex-induced vibration. J. Fluids Struct. 15 (3), 481–488.Google Scholar
Bourguet, R. & Lo Jacono, D. 2014 Flow-induced vibrations of a rotating cylinder. J. Fluid Mech. 740, 342–380.Google Scholar
Braza, M., Chassaing, P. & Minh, H. H. 1986 Numerical study and physical analysis of the pressure and velocity fields in the near wake of a circular cylinder. J. Fluid Mech. 165, 79–130.Google Scholar
Cagney, N. & Balabani, S. 2014 Streamwise vortex-induced vibrations of cylinders with one and two degrees of freedom. J. Fluid Mech. 758, 702–727.Google Scholar
Cao, S., Ozono, S., Hirano, K. & Tamura, Y. 2007 Vortex shedding and aerodynamic forces on a circular cylinder in linear shear flow at subcritical Reynolds number. J. Fluids Struct. 23 (5), 703–714.Google Scholar
Cao, S., Ozono, S., Tamura, Y., Ge, Y. & Kikugawa, H. 2010 Numerical simulation of Reynolds number effects on velocity shear flow around a circular cylinder. J. Fluids Struct. 26 (5), 685–702.Google Scholar
Cheng, M., Whyte, D. S. & Lou, J. 2007 Numerical simulation of flow around a square cylinder in uniform-shear flow. J. Fluids Struct. 23 (2), 207–226.Google Scholar
Chew, Y. T., Luo, S. C. & Cheng, M. 1997 Numerical study of a linear shear flow past a rotating cylinder. J. Wind Engng Ind. Aerodyn. 66 (2), 107–125.Google Scholar
Dahl, J. M., Hover, F. S., Triantafyllou, M. S. & Oakley, O. H. 2010 Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 643, 395–424.Google Scholar
Feng, C. C.1968 The measurement of vortex-induced effects in flow past stationary and oscillating circular and D-section cylinders. Master’s thesis, University of British Columbia.Google Scholar
Govardhan, R. N. & Williamson, C. H. K. 2000 Modes of vortex formation and frequency response of a freely vibrating cylinder. J. Fluid Mech. 420, 85–130.Google Scholar
Govardhan, R. N. & Williamson, C. H. K. 2006 Defining the ‘modified Griffin plot’ in vortex-induced vibration: revealing the effect of Reynolds number using controlled damping. J. Fluid Mech. 561, 147–180.Google Scholar
Hover, F. S., Techet, A. H. & Triantafyllou, M. S. 1998 Forces on oscillating uniform and tapered cylinders in crossflow. J. Fluid Mech. 363, 97–114.Google Scholar
Jauvtis, N. & Williamson, C. H. K. 2004 The effect of two degrees of freedom on vortex-induced vibration at low mass and damping. J. Fluid Mech. 509, 23–62.Google Scholar
Jordan, S. K. & Fromm, J. E. 1972 Laminar flow past a circle in a shear flow. Phys. Fluids 15 (6), 972–976.Google Scholar
Kang, S. 2006 Uniform-shear flow over a circular cylinder at low Reynolds numbers. J. Fluids Struct. 22 (4), 541–555.Google Scholar
Kang, S., Choi, H. & Lee, S. 1999 Laminar flow past a rotating circular cylinder. Phys. Fluids 11 (11), 3312–3321.Google Scholar
Khalak, A. & Williamson, C. H. K. 1997 Investigation of relative effects of mass and damping in vortex-induced vibration of a circular cylinder. J. Wind Engnng Ind. Aerodyn. 69, 341–350.Google Scholar
Khalak, A. & Williamson, C. H. K. 1999 Motions, forces and mode transitions in vortex-induced vibrations at low mass-damping. J. Fluids Struct. 13 (7), 813–851.Google Scholar
Kim, J., Kim, D. & Choi, H. 2001 An immersed-boundary finite-volume method for simulations of flow in complex geometries. J. Comput. Phys. 171 (1), 132–150.Google Scholar
Kiya, M., Tamura, H. & Arie, M. 1980 Vortex shedding from a circular cylinder in moderate-Reynolds-number shear flow. J. Fluid Mech. 101 (04), 721–735.Google Scholar
Kwon, T. S., Sung, H. J. & Hyun, J. M. 1992 Experimental investigation of uniform-shear flow past a circular cylinder. Trans. ASME J. Fluids Engng 114 (3), 457–460.Google Scholar
Lei, C., Cheng, L. & Kavanagh, K. 2000 A finite difference solution of the shear flow over a circular cylinder. Ocean Engng 27 (3), 271–290.Google Scholar
Leontini, J. S., Thompson, M. C. & Hourigan, K. 2006 The beginning of branching behaviour of vortex-induced vibration during two-dimensional flow. J. Fluids Struct. 22 (6), 857–864.Google Scholar
Liu, C., Zheng, X. & Sung, C. H. 1998 Preconditioned multigrid methods for unsteady incompressible flows. J. Comput. Phys. 139 (1), 35–57.CrossRefGoogle Scholar
Mittal, S. & Kumar, B. 2003 Flow past a rotating cylinder. J. Fluid Mech. 476 (4), 303–334.Google Scholar
Navrose & Mittal, S. 2013 Free vibrations of a cylinder: 3-D computations at Re = 1000. J. Fluids Struct. 41, 109–118.Google Scholar
Nemes, A., Zhao, J., Lo Jacono, D. & Sheridan, J. 2012 The interaction between flow-induced vibration mechanisms of a square cylinder with varying angles of attack. J. Fluid Mech. 710, 102–130.Google Scholar
Norberg, C.1987 Effects of Reynolds number and a low-intensity freestream turbulence on the flow around a circular cylinder. Tech. Rep. 2. Chalmers University, Goteborg, Sweden, Technological Publications.Google Scholar
Païdoussis, M. P., Price, S. J. & de Langre, E. 2010 Fluid-Structure Interactions: Cross-Flow-Induced Instabilities. Cambridge University Press.CrossRefGoogle Scholar
Prasanth, T. K. & Mittal, S. 2008 Vortex-induced vibrations of a circular cylinder at low Reynolds numbers. J. Fluid Mech. 594, 463–491.Google Scholar
Rao, A., Radi, A., Leontini, J. S., Thompson, M. C., Sheridan, J. & Hourigan, K. 2015 A review of rotating cylinder wake transitions. J. Fluids Struct. 53, 2–14.Google Scholar
Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19 (4), 389–447.Google Scholar
Shen, L., Chan, E.-S. & Lin, P. 2009 Calculation of hydrodynamic forces acting on a submerged moving object using immersed boundary method. Comput. Fluids 38 (3), 691–702.Google Scholar
Shiels, D., Leonard, A. & Roshko, A. 2001 Flow-induced vibration of a circular cylinder at limiting structural parameters. J. Fluids Struct. 15 (1), 3–21.Google Scholar
Singh, S. P. & Chatterjee, D. 2014 Impact of transverse shear on vortex induced vibrations of a circular cylinder at low Reynolds numbers. Comput. Fluids 93, 61–73.Google Scholar
Stojković, D., Breuer, M. & Durst, F. 2002 Effect of high rotation rates on the laminar flow around a circular cylinder. Phys. Fluids 14 (9), 3160–3178.Google Scholar
Sumner, D. & Akosile, O. O. 2003 On uniform planar shear flow around a circular cylinder at subcritical Reynolds number. J. Fluids Struct. 18 (3), 441–454.Google Scholar
Tamura, H., Kiya, M. & Arie, M. 1980 Numerical study on viscous shear flow past a circular cylinder. Bull. JSME 23 (186), 1952–1958.Google Scholar
Tritton, D. J. 1959 Experiments on the flow past a circular cylinder at low Reynolds numbers. J. Fluid Mech. 6 (04), 547–567.Google Scholar
Tu, J., Zhou, D., Bao, Y., Fang, C., Zhang, K., Li, C. & Han, Z. 2014 Flow-induced vibration on a circular cylinder in planar shear flow. Comput. Fluids 105, 138–154.Google Scholar
Wieselsberger, C.1922 New data on the laws of fluid resistance. Tech. Rep. National Advisory Comittee for Aeronautics.Google Scholar
Williamson, C. H. K. 1988 Defining a universal and continuous Strouhal–Reynolds number relationship for the laminar vortex shedding of a circular cylinder. Phys. Fluids 31 (10), 2742–2744.Google Scholar
Williamson, C. H. K. & Govardhan, R. N. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.Google Scholar
Yoshino, F. & Hayashi, T. 1984 The numerical solution of flow around a rotating circular cylinder in uniform shear flow. Bull. JSME 27 (231), 1850–1857.Google Scholar
Zhang, H., Fan, B., Chen, Z., Li, H. & Li, B. 2014 An in-depth study on vortex-induced vibration of a circular cylinder with shear flow. Comput. Fluids 100, 30–44.Google Scholar
Zhao, M. & Cheng, L. 2011 Numerical simulation of two-degree-of-freedom vortex-induced vibration of a circular cylinder close to a plane boundary. J. Fluids Struct. 27 (7), 1097–1110.Google Scholar