Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Steinfurth, Ben
and
Weiss, Julien
2021.
Velocity ratio effect on flow structures of non-parallel planar starting jets in cross-flow.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Mohammed-Taifour, Abdelouahab
and
Weiss, Julien
2021.
Periodic forcing of a large turbulent separation bubble.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Limbourg, Raphaël
and
Nedić, Jovan
2021.
An extension to the universal time scale for vortex ring formation.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Steinfurth, Ben
and
Weiss, Julien
2021.
Boundary-Layer Control by Means of Pulsed Jets at Different Inclination Angles.
AIAA Journal,
Vol. 59,
Issue. 10,
p.
3946.
Löffler, Stephan
Ebert, Carola
and
Weiss, Julien
2021.
Fluidic-Oscillator-Based Pulsed Jet Actuators for Flow Separation Control.
Fluids,
Vol. 6,
Issue. 4,
p.
166.
Krieg, Michael
and
Mohseni, Kamran
2021.
A new kinematic criterion for vortex ring pinch-off.
Physics of Fluids,
Vol. 33,
Issue. 3,
Steinfurth, Ben
and
Weiss, Julien
2021.
Development of inclined non-parallel planar starting jets in an adverse-pressure-gradient crossflow.
Steinfurth, Ben
and
Weiss, Julien
2022.
Efficiency Enhancement in Active Separation Control Through Optimizing the Duty Cycle of Pulsed Jets.
AIAA Journal,
Vol. 60,
Issue. 12,
p.
6566.
Steinfurth, Ben
and
Weiss, Julien
2022.
Modelling the decay of finite-span starting and stopping wall jets in an external stream.
Journal of Fluid Mechanics,
Vol. 951,
Issue. ,
2023.
The circulation growth of non-impulsive starting jet.
Physics of Fluids,
Vol. 35,
Issue. 5,
Wang, Lei
Feng, Lihao
and
Xu, Yang
2023.
Lagrangian analysis on structure evolution and mass transport of circular and noncircular turbulent synthetic jets.
Acta Mechanica Sinica,
Vol. 39,
Issue. 3,
Wang, Tianyuan
Shi, Hongda
Zhao, Ming
and
Zhang, Qin
2023.
Effects of a nozzle on the propeller wake in an oblique flow using modal analysis.
Journal of Fluid Mechanics,
Vol. 959,
Issue. ,
Löffler, Stephan
Steinfurth, Ben
and
Weiss, Julien
2023.
Surrogate-Based Exploration of Active Separation Control Parameters: An Experimental Study.
Saliba, Georges C.
Raimbault, Vincent
Batikh, Ahmad
Colin, Stéphane
and
Baldas, Lucien
2023.
Relaxation Fluidic Oscillators: Design Parameters, New Operating Modes, and Characteristics of Their Internal and External Flows.
Journal of Fluids Engineering,
Vol. 145,
Issue. 10,
Steinfurth, B.
2024.
Spatial super-resolution of finite-time Lyapunov exponent fields with generative adversarial networks.
Physics of Fluids,
Vol. 36,
Issue. 2,
Steinfurth, B.
and
Weiss, J.
2024.
Assimilating experimental data of a mean three-dimensional separated flow using physics-informed neural networks.
Physics of Fluids,
Vol. 36,
Issue. 1,
Löffler, Stephan
Thieme, Mathis
Steinfurth, Ben
and
Weiss, Julien
2024.
Wind-Tunnel-in-the-Loop Exploration and Optimization of Active Flow Control Parameters.
AIAA Journal,
Vol. 62,
Issue. 10,
p.
3776.
Steinfurth, B.
Hassanein, A.
Doan, N. A. K.
and
Scarano, F.
2024.
Physics-informed neural networks for dense reconstruction of vortex rings from particle tracking velocimetry.
Physics of Fluids,
Vol. 36,
Issue. 9,
Zheng, Haojun
Gao, Lei
and
Yu, Simon C.M.
2025.
Formation process of a two-dimensional starting jet.
Journal of Fluid Mechanics,
Vol. 1007,
Issue. ,
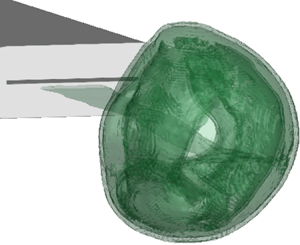
 $\eta >0.9$ are generated. They continue to absorb vorticity far beyond the universal formation time of
$\eta >0.9$ are generated. They continue to absorb vorticity far beyond the universal formation time of  $t^*\approx 4$ applicable to axisymmetric parallel starting jets introduced by Gharib et al. (J. Fluid Mech., vol. 360, 1998, pp. 121–140). Here, the maximum circulation and the corresponding formation time depend on the magnitude of over-pressure in the outlet plane. After momentarily reaching a non-dimensional energy close to or even below a value of
$t^*\approx 4$ applicable to axisymmetric parallel starting jets introduced by Gharib et al. (J. Fluid Mech., vol. 360, 1998, pp. 121–140). Here, the maximum circulation and the corresponding formation time depend on the magnitude of over-pressure in the outlet plane. After momentarily reaching a non-dimensional energy close to or even below a value of  $\alpha =0.16$ associated with Hill's spherical vortex, vorticity is continuously shed into a trailing jet, and the vortex rings evolve into unsteady thinner-core states. No separation between the vortex ring and the trailing jet (pinch-off) is observed. The present study provides new insights into the flow physics of non-parallel planar starting jets that significantly differ from parallel starting flows investigated previously. The potential for active flow control applications is discussed.
$\alpha =0.16$ associated with Hill's spherical vortex, vorticity is continuously shed into a trailing jet, and the vortex rings evolve into unsteady thinner-core states. No separation between the vortex ring and the trailing jet (pinch-off) is observed. The present study provides new insights into the flow physics of non-parallel planar starting jets that significantly differ from parallel starting flows investigated previously. The potential for active flow control applications is discussed.