Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-28T20:55:25.340Z Has data issue: false hasContentIssue false
  • English
  • Français

Vortex moment map for unsteady incompressible viscous flows

Published online by Cambridge University Press:  23 March 2020

Juan Li Open the ORCID record for Juan Li [Opens in a new window] ,
Yinan Wang Open the ORCID record for Yinan Wang [Opens in a new window] ,
Michael Graham Open the ORCID record for Michael Graham [Opens in a new window]  and
Xiaowei Zhao Open the ORCID record for Xiaowei Zhao [Opens in a new window]
Juan Li
Affiliation:
School of Engineering, The University of Warwick, Coventry CV4 7AL, UK
Yinan Wang
Affiliation:
School of Engineering, The University of Warwick, Coventry CV4 7AL, UK
Michael Graham
Affiliation:
Department of Aeronautics, Imperial College, London SW7 2BY, UK
Xiaowei Zhao*
Affiliation:
School of Engineering, The University of Warwick, Coventry CV4 7AL, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

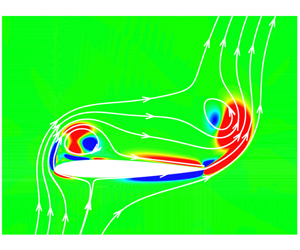
In this paper, a vortex moment map (VMM) method is proposed to predict the pitching moment on a body from the vorticity field. VMM is designed to identify the moment contribution of each given vortex in the flow field. Implementing this VMM approach in starting flows of a NACA0012 airfoil, it is found that, due to the rolling up of leading-edge vortices (LEVs) and trailing-edge vortices (TEVs), the unsteady nose-down moment about the quarter chord is higher than the steady-state value. The time variation of the unsteady moment is closely related to the LEVs and TEVs near the body and the VMM gives an intuitive understanding of how each part of the vorticity field contributes to the pitching moment on the body.

JFM classification

Vortex Flows: Vortex shedding Wakes/Jets: Separated flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 891 , 25 May 2020 , A13
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Chiereghin, N., Cleaver, D. J. & Gursul, I. 2019 Unsteady lift and moment of a periodically plunging airfoil. AIAA J. 57 (1), 208–222.CrossRefGoogle Scholar
DeVoria, A. C., Carr, Z. R. & Ringuette, M. J. 2014 On calculating forces from the flow field with application to experimental volume data. J. Fluid Mech. 749, 297–319.CrossRefGoogle Scholar
Dickinson, M. H. & Gotz, K. G. 1993 Unsteady aerodynamic performance of model wings at low Reynolds numbers. J. Expl. Biol. 174, 45–64.Google Scholar
Eldredge, J. D. & Jones, A. R.Leading-edge vortices: mechanics and modeling. Annu. Rev. Fluid Mech. 51, 75–104.CrossRefGoogle Scholar
Ellington, C. P., Van Den Berg, C., Willmott, A. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384, 626–630.CrossRefGoogle Scholar
Fernandez-Feria, R. & Alaminos-Quesada, J. 2018 Unsteady thrust, lift and moment of a two-dimensional flapping thin airfoil in the presence of leading-edge vortices: a first approximation from linear potential theory. J. Fluid Mech. 851, 344–373.CrossRefGoogle Scholar
Graham, W. R., Pitt Ford, C. W. & Babinsky, H. 2017 An impulse-based approach to estimating forces in unsteady flow. J. Fluid Mech. 815, 60–76.CrossRefGoogle Scholar
Ham, N. D. & Maurice, I. Y. 1966 Torsional oscillation of helicopter blades due to stall. J. Aircraft 3.3, 218–224.CrossRefGoogle Scholar
Hansen, M. H. 2007 Aeroelastic instability problems for wind turbines. Wind Energy: Intl J. Prog. Appl. Wind Power Conversion Technol. 10.6, 551–577.CrossRefGoogle Scholar
Howe, M. S. 1995 On the force and moment on a body in an incompressible fluid, with application to rigid bodies and bubbles at high Reynolds numbers. Q. J. Mech. Appl. Maths 48, 401–425.CrossRefGoogle Scholar
Katz, J. & Plotkin, A. 2001 Low-speed Aerodynamics, 2nd edn. Cambridge University Press.CrossRefGoogle Scholar
Koumoutsakos, P., Leonard, A. & Pepin, F. 1994 Boundary conditions for viscous vortex methods. J. Comput. Phys. 113, 52–61.CrossRefGoogle Scholar
Krashanitsa, R. Y., Silin, D., Shkarayev, S. V. & Abate, G. 2009 Flight dynamics of a flapping-wing air vehicle. Intl J. Micro Air Vehicles 1.1, 35–49.CrossRefGoogle Scholar
Li, J. & Wu, Z. N. 2015 Unsteady lift for the Wagner problem in the presence of additional leading/trailing edge vortices. J. Fluid Mech. 769, 182–217.CrossRefGoogle Scholar
Li, J. & Wu, Z. N. 2016 A vortex force study for a flat plate at high angle of attack. J. Fluid Mech. 801, 222–249.CrossRefGoogle Scholar
Li, J. & Wu, Z. N. 2018 Vortex force map method for viscous flows of general airfoils. J. Fluid Mech. 836, 145–166.CrossRefGoogle Scholar
Lin, J. C. & Rockwell, D. 1996 Force identification by vorticity fields: techniques based on flow imaging. J. Fluids Struct. 10, 663–668.CrossRefGoogle Scholar
Mancini, P., Manar, F., Granlund, K., Ol, M. V. & Jones, A. R. 2015 Unsteady aerodynamic characteristics of a translating rigid wing at low Reynolds number. Phys. Fluids 27 (12), 123102.CrossRefGoogle Scholar
Noca, F.(1996) On the evaluation of instantaneous fluid-dynamic forces on a bluff body. GALCIT Report FM96-5.Google Scholar
Noca, F., Shiels, D. & Jeon, D. 1997 Measuring instantaneous fluid dynamic forces on bodies, using only velocity fields and their derivatives. J. Fluids Struct. 11, 345–350.CrossRefGoogle Scholar
Ohtake, T., Nakae, Y. & Motohashi, T. 2007 Nonlinearity of the aerodynamic characteristics of NACA0012 aerofoil at low Reynolds numbers. J. Japan Soc. Aeronaut. Space Sci. 55, 439–445.Google Scholar
Pitt Ford, C. W. & Babinsky, H. 2013 Lift and the leading-edge vortex. J. Fluid Mech. 720, 280–313.CrossRefGoogle Scholar
Polhamus, E. C.1966 A concept of the vortex lift of sharp-edge delta wings based on a leading-edge-suction analogy. NACA Technical Note, D–3767.Google Scholar
Ramesh, K., Gopalarathnam, A., Granlund, K., Ol, M. V. & Edwards, J. R. 2014 Discrete-vortex method with novel shedding criterion for unsteady aerofoil flows with intermittent leading-edge vortex shedding. J. Fluid Mech. 751, 500–538.CrossRefGoogle Scholar
Rainbird, J. M.2016 Blockage tolerant wind tunnel testing of aerofoils at angles of incidence from $0^{\circ }$ to $360^{\circ }$, with respect to the self-start of vertical-axis wind turbines. PhD thesis, Imperial College London.Google Scholar
Wu, J. C. 1981 Theory for aerodynamic force and moment in viscous flows. AIAA J. 19, 432–441.CrossRefGoogle Scholar
Wu, J. Z., Lu, X. Y. & Zhuang, L. X. 2007 Integral force acting on a body due to local flow structures. J. Fluid Mech. 576, 265–286.CrossRefGoogle Scholar
Xia, X. & Mohseni, K. 2017 Unsteady aerodynamics and vortex-sheet formation of a two-dimensional airfoil. J. Fluid Mech. 830, 439–478.CrossRefGoogle Scholar
Zhao, X., Gouder, K., Graham, J. M. R. & Limebeer, D. J. N. 2016 Buffet response and control of a suspension bridge section in a turbulent wind. J. Fluids Struct. 62, 384–412.CrossRefGoogle Scholar
Zhu, G., Bearman, P. W. & Graham, J. M. R. 2002 Prediction of drag and lift by using velocity and vorticity fields. Aeronaut. J. 106 (1064), 547–554.Google Scholar