Published online by Cambridge University Press: 27 October 2020
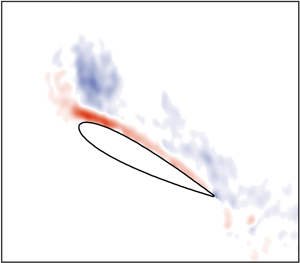
In many applications, conventional aerofoils are subject to a number of simultaneous motions that complicate the prediction of flow separation. The purpose of this work is to evaluate the impact of a large-amplitude free-stream oscillation on the timing of vortex formation for a simultaneously surging and pitching wing. Experimental flow field measurements were obtained on a NACA 0012 aerofoil over a wide range of surge amplitudes ( $1.50 \leq \lambda \leq 2.25$) and reduced frequencies (
$1.50 \leq \lambda \leq 2.25$) and reduced frequencies ( $0.1 \leq k \leq 0.3$). Particular attention was paid to how various mechanisms of flow separation, specifically the velocity induced by the trailing wake and unsteady effects in the boundary layer, were impacted by a change in the properties of the surge motion. In the regime where
$0.1 \leq k \leq 0.3$). Particular attention was paid to how various mechanisms of flow separation, specifically the velocity induced by the trailing wake and unsteady effects in the boundary layer, were impacted by a change in the properties of the surge motion. In the regime where  $k \leq 0.3$, a change in the surge kinematics primarily manifested as a change in the relative strength of the trailing wake. Boundary layer unsteadiness was found to have a negligible influence on the timing of vortex formation in the same flow regime. Thus, the timing of leading-edge vortex formation was well predicted by a combination of an unsteady inviscid flow solver and a quasi-steady treatment of the boundary layer, a promising result for low-order predictions of vortex behaviour in unsteady aerofoil flows.
$k \leq 0.3$, a change in the surge kinematics primarily manifested as a change in the relative strength of the trailing wake. Boundary layer unsteadiness was found to have a negligible influence on the timing of vortex formation in the same flow regime. Thus, the timing of leading-edge vortex formation was well predicted by a combination of an unsteady inviscid flow solver and a quasi-steady treatment of the boundary layer, a promising result for low-order predictions of vortex behaviour in unsteady aerofoil flows.