Published online by Cambridge University Press: 29 February 2024
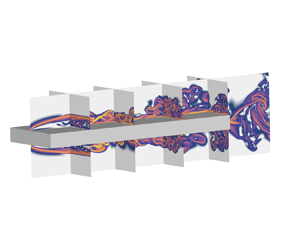
The numerical investigation focuses on the flow patterns around a rectangular cylinder with three aspect ratios ( $L/D=5$,
$L/D=5$,  $10$,
$10$,  $15$) at a Reynolds number of
$15$) at a Reynolds number of  $1000$. The study delves into the dynamics of vortices, their associated frequencies, the evolution of the boundary layer and the decay of the wake. Kelvin–Helmholtz (KH) vortices originate from the leading edge (LE) shear layer and transform into hairpin vortices. Specifically, at
$1000$. The study delves into the dynamics of vortices, their associated frequencies, the evolution of the boundary layer and the decay of the wake. Kelvin–Helmholtz (KH) vortices originate from the leading edge (LE) shear layer and transform into hairpin vortices. Specifically, at  $L/D=5$, three KH vortices merge into a single LE vortex. However, at
$L/D=5$, three KH vortices merge into a single LE vortex. However, at  $L/D=10$ and
$L/D=10$ and  $15$, two KH vortices combine to form a LE vortex, with the rapid formation of hairpin vortex packets. A fractional harmonic arises due to feedback from the split LE shear layer moving upstream, triggering interaction with the reverse flow. Trailing edge (TE) vortices shed, creating a Kármán-like street in the wake. The intensity of wake oscillation at
$15$, two KH vortices combine to form a LE vortex, with the rapid formation of hairpin vortex packets. A fractional harmonic arises due to feedback from the split LE shear layer moving upstream, triggering interaction with the reverse flow. Trailing edge (TE) vortices shed, creating a Kármán-like street in the wake. The intensity of wake oscillation at  $L/D=5$ surpasses that in the other two cases. Boundary layer transition occurs after the saturation of disturbance energy for
$L/D=5$ surpasses that in the other two cases. Boundary layer transition occurs after the saturation of disturbance energy for  $L/D=10$ and
$L/D=10$ and  $15$, but not for
$15$, but not for  $L/D=5$. The low-frequency disturbances are selected to generate streaks inside the boundary layer. The TE vortex shedding induces the formation of a favourable pressure gradient, accelerating the flow and fostering boundary layer relaminarization. The self-similarity of the velocity defect is observed in all three wakes, accompanied by the decay of disturbance energy. Importantly, the decrease in the shedding frequency of LE (TE) vortices significantly contributes to the overall decay of disturbance energy. This comprehensive exploration provides insights into complex flow phenomena and their underlying dynamics.
$L/D=5$. The low-frequency disturbances are selected to generate streaks inside the boundary layer. The TE vortex shedding induces the formation of a favourable pressure gradient, accelerating the flow and fostering boundary layer relaminarization. The self-similarity of the velocity defect is observed in all three wakes, accompanied by the decay of disturbance energy. Importantly, the decrease in the shedding frequency of LE (TE) vortices significantly contributes to the overall decay of disturbance energy. This comprehensive exploration provides insights into complex flow phenomena and their underlying dynamics.