Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Mitroglou, Nicholas
McLorn, Michael
Gavaises, Manolis
Soteriou, Celia
and
Winterbourne, Mark
2014.
Instantaneous and ensemble average cavitation structures in Diesel micro-channel flow orifices.
Fuel,
Vol. 116,
Issue. ,
p.
736.
Jha, Narsing K.
and
Govardhan, R. N.
2015.
Interaction of a vortex ring with a single bubble: bubble and vorticity dynamics.
Journal of Fluid Mechanics,
Vol. 773,
Issue. ,
p.
460.
Cui, Bing
Ni, Baoyu
and
Wu, Qigang
2016.
Bubble–bubble interaction effects on dynamics of multiple bubbles in a vortical flow field.
Advances in Mechanical Engineering,
Vol. 8,
Issue. 2,
Finn, Justin R.
Li, Ming
and
Apte, Sourabh V.
2016.
Particle based modelling and simulation of natural sand dynamics in the wave bottom boundary layer.
Journal of Fluid Mechanics,
Vol. 796,
Issue. ,
p.
340.
Zhang, Lingxin
Chen, Linya
and
Shao, Xueming
2016.
The migration and growth of nuclei in an ideal vortex flow.
Physics of Fluids,
Vol. 28,
Issue. 12,
Rettinger, C.
and
Rüde, U.
2018.
A coupled lattice Boltzmann method and discrete element method for discrete particle simulations of particulate flows.
Computers & Fluids,
Vol. 172,
Issue. ,
p.
706.
Chen, Linya
Zhang, Lingxin
Peng, Xiaoxing
and
Shao, Xueming
2019.
Influence of water quality on the tip vortex cavitation inception.
Physics of Fluids,
Vol. 31,
Issue. 2,
Nguyen, Van Luc
Degawa, Tomohiro
and
Uchiyama, Tomomi
2019.
Numerical simulation of the interaction between a vortex ring and a bubble plume.
International Journal of Numerical Methods for Heat & Fluid Flow,
Vol. 29,
Issue. 9,
p.
3192.
Pakseresht, Pedram
and
Apte, Sourabh V.
2019.
Volumetric displacement effects in Euler-Lagrange LES of particle-laden jet flows.
International Journal of Multiphase Flow,
Vol. 113,
Issue. ,
p.
16.
Nguyen, Van Luc
Degawa, Tomohiro
and
Uchiyama, Tomomi
2019.
Numerical simulation of annular bubble plume by vortex in cell method.
International Journal of Numerical Methods for Heat & Fluid Flow,
Vol. 29,
Issue. 3,
p.
1103.
Cao, Tianyi
He, Zhixia
Zhou, Han
Guan, Wei
Zhang, Liang
and
Wang, Qian
2020.
Experimental study on the effect of vortex cavitation in scaled-up diesel injector nozzles and spray characteristics.
Experimental Thermal and Fluid Science,
Vol. 113,
Issue. ,
p.
110016.
Gomez Santos, Eduardo
Shi, Junmei
Gavaises, Manolis
Soteriou, Celia
Winterbourn, Mark
and
Bauer, Wolfgang
2020.
Investigation of cavitation and air entrainment during pilot injection in real-size multi-hole diesel nozzles.
Fuel,
Vol. 263,
Issue. ,
p.
116746.
Tjahjono, Tri
Wardana, I. N. G.
Sasongko, Mega Nur
and
Widodo, Agung Sugeng
2020.
Experimental and theoretical bubble growth comparison at the initial stages of horizontal injection.
Eastern-European Journal of Enterprise Technologies,
Vol. 3,
Issue. 7 (105),
p.
36.
Pakseresht, Pedram
Esmaily, Mahdi
and
Apte, Sourabh V.
2020.
A correction scheme for wall-bounded two-way coupled point-particle simulations.
Journal of Computational Physics,
Vol. 420,
Issue. ,
p.
109711.
Guan, Wei
He, Zhixia
Duan, Lian
Cao, Tianyi
Sun, Shenxing
and
Zhang, Liang
2021.
Optical investigations of nozzle geometrical and dynamic factors on formation and development characteristics of string cavitation with large-scale diesel tapered-hole nozzles.
International Journal of Engine Research,
Vol. 22,
Issue. 10,
p.
3147.
Sharma, Shubham
Singh, Awanish Pratap
and
Basu, Saptarshi
2021.
On the dynamics of vortex–droplet co-axial interaction: insights into droplet and vortex dynamics.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Xiong, Jinbiao
Xie, Hao
Du, Sijia
and
Cheng, Xu
2022.
Experimental Measurement of Bubbly Two-Phase Flow in a 4x4 Rod Bundle with Wire Mesh Sensor.
SSRN Electronic Journal ,
Biswas, Subhajit
and
Govardhan, Raghuraman N.
2022.
Interaction of a rigid buoyant sphere and a deforming bubble with a vortex ring: The role of deformability.
Physical Review Fluids,
Vol. 7,
Issue. 9,
Abu-Bakr, Ali F.
and
Abu-Nab, Ahmed K.
2022.
Growth of lipid-coated multi-microbubbles in viscoelastic tissues.
The European Physical Journal Plus,
Vol. 137,
Issue. 4,
Abu-Nab, Ahmed K.
and
Abu-Bakr, Ali F.
2022.
Effect of bubble-bubble interaction in Cu−Al2O3/H2O hybrid nanofluids during multibubble growth process.
Case Studies in Thermal Engineering,
Vol. 33,
Issue. ,
p.
101973.
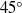 $4{5}^{\circ } $ with the vortex travel direction, resulting in wakes directed towards the vortex centre. Finally, a simple modification to the standard point-particle two-way coupling approach is developed wherein the interphase reaction source terms are consistently altered to account for the fluid displacement effects and reactions due to bubble accelerations.
$4{5}^{\circ } $ with the vortex travel direction, resulting in wakes directed towards the vortex centre. Finally, a simple modification to the standard point-particle two-way coupling approach is developed wherein the interphase reaction source terms are consistently altered to account for the fluid displacement effects and reactions due to bubble accelerations.