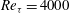Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-26T16:40:01.109Z Has data issue: false hasContentIssue false
  • English
  • Français

Velocity statistics in turbulent channel flow up to $Re_{\tau }=4000$

Published online by Cambridge University Press:  21 February 2014

Matteo Bernardini ,
Sergio Pirozzoli  and
Paolo Orlandi
Matteo Bernardini
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Università di Roma ‘La Sapienza’ Via Eudossiana 18, 00184 Roma, Italy
Sergio Pirozzoli*
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Università di Roma ‘La Sapienza’ Via Eudossiana 18, 00184 Roma, Italy
Paolo Orlandi
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Università di Roma ‘La Sapienza’ Via Eudossiana 18, 00184 Roma, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The high-Reynolds-number behaviour of the canonical incompressible turbulent channel flow is investigated through large-scale direct numerical simulation (DNS). A Reynolds number is achieved (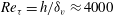$Re_{\tau } = h/\delta _v \approx 4000$, where 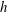$h$ is the channel half-height, and 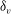$\delta _v$ is the viscous length scale) at which theory predicts the onset of phenomena typical of the asymptotic Reynolds number regime, namely a sensible layer with logarithmic variation of the mean velocity profile, and Kolmogorov scaling of the velocity spectra. Although higher Reynolds numbers can be achieved in experiments, the main advantage of the present DNS study is access to the full three-dimensional flow field. Consistent with refined overlap arguments (Afzal & Yajnik, J. Fluid Mech. vol. 61, 1973, pp. 23–31; Jiménez & Moser, Phil. Trans. R. Soc. Lond. A, vol. 365, 2007, pp. 715–732), our results suggest that the mean velocity profile never achieves a truly logarithmic profile, and the logarithmic diagnostic function instead exhibits a linear variation in the outer layer whose slope decreases with the Reynolds number. The extrapolated value of the von Kármán constant is 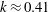$k \approx 0.41$. A near logarithmic layer is observed in the spanwise velocity variance, as predicted by Townsend’s attached eddy hypothesis, whereas the streamwise variance seems to exhibit a shoulder, perhaps being still affected by low-Reynolds-number effects. Comparison with previous DNS data at lower Reynolds number suggests enhancement of the imprinting effect of outer-layer eddies onto the near-wall region. This mechanisms is associated with excess turbulence kinetic energy production in the outer layer, and it reflects in flow visualizations and in the streamwise velocity spectra, which exhibit sharp peaks in the outer layer. Associated with the outer energy production site, we find evidence of a Kolmogorov-like inertial range, limited to the spanwise spectral density of $u$, whereas power laws with different exponents are found for the other spectra. Finally, arguments are given to explain the ‘odd’ scaling of the streamwise velocity variances, based on the analysis of the kinetic energy production term.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 742 , 10 March 2014 , pp. 171 - 191
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Afzal, N. & Yajnik, K. 1973 Analysis of turbulent pipe and channel flows at moderately large Reynolds number. J. Fluid Mech. 61, 23–31.Google Scholar
del Álamo, J. C. & Jiménez, J. 2003 Spectra of the very large anisotropic scales in turbulent channels. Phys. Fluids 15, L41–L44.CrossRefGoogle Scholar
del Álamo, J. C. & Jiménez, J. 2006 Linear energy amplification in turbulent channels. J. Fluid Mech. 559, 205–213.Google Scholar
del Álamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.Google Scholar
Bernardini, M. & Pirozzoli, S. 2009 A general strategy for the optimization of Runge–Kutta schemes for wave propagation phenomena. J. Comput. Phys. 228, 4182–4199.Google Scholar
Bernardini, M., Pirozzoli, S., Quadrio, M. & Orlandi, P. 2013 Turbulent channel flow simulations in convecting reference frames. J. Comput. Phys. 232, 1–6.CrossRefGoogle Scholar
Bradshaw, P. 1967 Inactive motion and pressure fluctuations in turbulent boundary layers. J. Fluid Mech 30, 241–258.Google Scholar
Dean, R. B. 1978 Reynolds number dependence of skin friction and other bulk flow variables in two-dimensional rectangular duct flow. Trans. ASME: J. Fluids Engng 100, 215–223.Google Scholar
DeGraaff, D. B. & Eaton, J. K. 2000 Reynolds-number scaling of the flat-plate turbulent boundary layer. J. Fluid Mech. 422, 319–346.CrossRefGoogle Scholar
Durand, W. F. 1935 Aerodynamic Theory. Springer.Google Scholar
Ferchichi, M. & Tavoularis, S. 2000 Reynolds number effects on the fine structure of uniformly sheared turbulence. Phys. Fluids 12, 2942–2953.CrossRefGoogle Scholar
Flores, O. & Jimenez, J. 2010 Hierarchy of minimal flow units in the logarithmic layer. Phys. Fluids 22, 071704.Google Scholar
Guala, M., Hommema, S. E. & Adrian, R. J. 2006 Large-scale and very-large-scale motions in turbulent pipe flow. J. Fluid Mech. 554, 521–542.Google Scholar
Hamilton, J. M., Kim, J. & Waleffe, F. 1995 Regeneration mechanisms of near-wall turbulent structures. J. Fluid Mech. 287, 317–348.Google Scholar
Hoyas, S. & Jiménez, J. 2006 Scaling of velocity fluctuations in turbulent channels up to $Re_{\tau } = 2003$ . Phys. Fluids 18, 011702.CrossRefGoogle Scholar
Hoyas, S. & Jiménez, J. 2008 Reynolds number effects on the Reynolds-stress budgets in turbulent channels. Phys. Fluids 20, 101511.CrossRefGoogle Scholar
Hultmark, M., Bailey, S. C. C. & Smits, A. J. 2010 Scaling of near-wall turbulence in pipe flow. J. Fluid Mech. 649, 103–113.CrossRefGoogle Scholar
Hunt, J. C. R. & Morrison, J. F. 2001 Eddy structure in turbulent boundary layers. Eur. J. Mech. (B/Fluids) 19, 673–694.Google Scholar
Hutchins, N. & Marusic, I. 2007 Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.Google Scholar
Jiménez, J. & Hoyas, S. 2008 Turbulent fluctuations above the buffer layer of wall-bounded flows. J. Fluid Mech. 611, 215–236.CrossRefGoogle Scholar
Jiménez, J. & Moser, R. D. 2007 What are we learning from simulating wall turbulence?. Phil. Trans. R. Soc. Lond. A 365, 715–732.Google Scholar
Jiménez, J. & Pinelli, A. 1999 The autonomous cycle of near–wall turbulence. J. Fluid Mech. 389, 335–359.CrossRefGoogle Scholar
Jiménez, J., Wray, A. A., Saffman, P. G. & Rogallo, R. S. 1993 The structure of intense vorticity in isotropic turbulence. J. Fluid Mech. 255, 65–90.Google Scholar
Kim, K. C. & Adrian, R. J. 1999 Very large-scale motion in the outer layer. Phys. Fluids 11, 417–422.Google Scholar
Kim, J. & Moin, P. 1985 Application of a fractional-step method to incompressible Navier–Stokes equations. J. Comput. Phys. 59, 308–323.Google Scholar
Klewicki, J. C. 2010 Reynolds number dependence, scaling, and dynamics of turbulent boundary layers. Trans. ASME: J. Fluids Engng 132, 094001.Google Scholar
Klewicki, J. C. & Falco, R. E. 1990 On accurately measuring statistics associated with small scale structure in a turbulent boundary layers using hot wire probes. J. Fluid Mech. 541, 21–54.Google Scholar
Mansour, N. N., Kim, J. & Moin, P. 1988 Reynolds-stress and dissipation-rate budgets in a turbulent channel flow. J. Fluid Mech. 194, 15–44.Google Scholar
Marusic, I. & Kunkel, G. J. 2010 Streamwise turbulence intensity formulation for flat-plate boundary layers. Phys. Fluids 22, 051704.Google Scholar
Marusic, I., McKeon, B. J., Monkewitz, P. A., Nagib, H. M., Smits, A. J. & Sreenivasan, K. R. 2010 Wall-bounded turbulent flows at high Reynolds numbers: Recent advances and key issues. Phys. Fluids 22, 065103.Google Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, R3.Google Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2009 Large-scale amplitude modulation of the small-scale structures in turbulent boundary layers. J. Fluid Mech. 628, 311–337.Google Scholar
McKeon, B. J. & Morrison, J. F. 2007 Asymptotic scaling in turbulent pipe flow. Phil. Trans. R. Soc. Lond. A 365, 771–787.Google Scholar
Metzger, M. M. & Klewicki, J. C. 2001 A comparative study of near-wall turbulence in high and low Reynolds number boundary layers. Phys. Fluids 13, 692–701.CrossRefGoogle Scholar
Mydlarski, L. & Warhaft, Z. 1996 On the onset of high-Reynolds-number grid-generated wind tunnel turbulence. J. Fluid Mech. 320, 331–368.Google Scholar
Nagib, H. M. & Chauhan, K. A. 2008 Variations of von Kármán coefficient in canonical flows. Phys. Fluids 20, 101518.CrossRefGoogle Scholar
Nickels, T. B., Marusic, I., Hafez, S. M. & Chong, M. S. 2005 Evidence of the $k^{-1}$ law in a high-Reynolds-number turbulent boundary layer. Phys. Rev. Lett. 95, 074501.Google Scholar
Orlandi, P. 1997 Helicity fluctuations and turbulent energy production in rotating and nonrotating pipes. Phys. Fluids 9, 2045–2056.Google Scholar
Orlandi, P. 2000 Fluid Flow Phenomena: A Numerical Toolkit. Kluwer.Google Scholar
Perry, A. E. & Abell, C. J. 1977 Asymptotic similarity of turbulence structures in smooth-and rough-walled pipes. J. Fluid Mech. 79, 785–799.Google Scholar
Perry, A. E. & Li, J. D. 1990 Experimental support for the attached-eddy hypothesis in zero-pressure-gradient turbulent boundary layers. J. Fluid Mech. 218, 405–438.CrossRefGoogle Scholar
Pirozzoli, S. & Bernardini, M. 2013 Probing high-Reynolds-number effects in numerical boundary layers. Phys. Fluids 25, 021704.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Rogers, M. M. & Moin, P. 1987 The structure of the vorticity field in homogeneous turbulent flows. J. Fluid Mech. 176, 33–66.CrossRefGoogle Scholar
Saddoughi, S. G. & Veeravalli, S. V. 1994 Local isotropy in turbulent boundary layers at high Reynolds number. J. Fluid Mech. 268, 333–372.Google Scholar
Schultz, M. P. & Flack, K. A. 2013 Reynolds-number scaling of turbulent channel flow. Phys. Fluids 25, 025104.Google Scholar
Sillero, J., Jiménez, J. & Moser, R. D. 2013 One-point statistics for turbulent wall-bounded flows at Reynolds numbers up to $\delta ^+ \approx 2000$ . Phys. Fluids 25 (105102).Google Scholar
Simens, M. P., Jiménez, J., Hoyas, S. & Mizuno, Y. 2009 A high-resolution code for a turbulent boundary layers. J. Comput. Phys. 228, 4218–4231.Google Scholar
Smith, C. R. & Metzler, S. P. 1983 The characteristics of low-speed streaks in the near-wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.CrossRefGoogle Scholar
Townsend, A. A. 1961 Equilibrium layers and wall turbulence. J. Fluid Mech. 11, 97–120.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. 2nd edn. Cambridge University Press.Google Scholar
Wu, X. & Moin, P. 2008 A direct numerical simulation study on the mean velocity characteristics in turbulent pipe flow. J. Fluid Mech. 608, 81–112.Google Scholar