Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-23T18:48:29.712Z Has data issue: false hasContentIssue false
  • English
  • Français

Vapour-bubble nucleation and dynamics in turbulent Rayleigh–Bénard convection

Published online by Cambridge University Press:  13 April 2016

Daniela Narezo Guzman ,
Tomasz Frączek ,
Christopher Reetz ,
Chao Sun ,
Detlef Lohse  and
Guenter Ahlers
Daniela Narezo Guzman*
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J.M. Burgers Center for Fluid Dynamics, and Impact-Institute, University of Twente, 7500 AE Enschede, The Netherlands Department of Physics, University of California, Santa Barbara, CA 93106, USA
Tomasz Frączek
Affiliation:
Department of Physics, University of California, Santa Barbara, CA 93106, USA
Christopher Reetz
Affiliation:
Department of Physics, University of California, Santa Barbara, CA 93106, USA
Chao Sun
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J.M. Burgers Center for Fluid Dynamics, and Impact-Institute, University of Twente, 7500 AE Enschede, The Netherlands Center for Combustion Energy and Department of Thermal Engineering, Tsinghua University, Beijing 100084, China
Detlef Lohse
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J.M. Burgers Center for Fluid Dynamics, and Impact-Institute, University of Twente, 7500 AE Enschede, The Netherlands Max-Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany
Guenter Ahlers
Affiliation:
Department of Physics, University of California, Santa Barbara, CA 93106, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

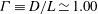Vapour bubbles nucleating at micro-cavities etched into the silicon bottom plate of a cylindrical Rayleigh–Bénard sample (diameter $D=8.8$  cm, aspect ratio ${\it\Gamma}\equiv D/L\simeq 1.00$ where $L$ is the sample height) were visualized from the top and from the side. A triangular array of cylindrical micro-cavities (with a diameter of 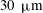$30~{\rm\mu}\text{m}$ and a depth of 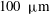$100~{\rm\mu}\text{m}$) covered a circular centred area (diameter of 2.5 cm) of the bottom plate. Heat was applied to the sample only over this central area while cooling was over the entire top-plate area. Bubble sizes and frequencies of departure from the bottom plate are reported for a range of bottom-plate superheats 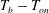$T_{b}-T_{on}$ (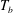$T_{b}$ is the bottom-plate temperature, 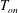$T_{on}$ is the onset temperature of bubble nucleation) from 3 to 12 K for three different cavity separations. The difference $T_{b}-T_{t}\simeq 16$  K between 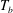$T_{b}$ and the top plate temperature 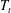$T_{t}$ was kept fixed while the mean temperature $T_{m}=(T_{b}+T_{t})/2$ was varied, leading to a small range of the Rayleigh number 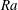$Ra$ from 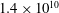$1.4\times 10^{10}$ to $2.0\times 10^{10}$. The time between bubble departures from a given cavity decreased exponentially with increasing superheat and was independent of cavity separation. The contribution of the bubble latent heat to the total enhancement of heat transferred due to bubble nucleation was found to increase with superheat, reaching up to 25 %. The bubbly flow was examined in greater detail for a superheat of 10 K and 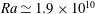$Ra\simeq 1.9\times 10^{10}$. The condensation and/or dissolution rates of departed bubbles revealed two regimes: the initial rate was influenced by steep thermal gradients across the thermal boundary layer near the plate and was two orders of magnitude larger than the final condensation and/or dissolution rate that prevailed once the rising bubbles were in the colder bulk flow of nearly uniform temperature. The dynamics of thermal plumes was studied qualitatively in the presence and absence of nucleating bubbles. It was found that bubbles enhanced the plume velocity by a factor of four or so and drove a large-scale circulation (LSC). Nonetheless, even in the presence of bubbles the plumes and LSC had a characteristic velocity which was smaller by a factor of five or so than the bubble-rise velocity in the bulk. In the absence of bubbles there was strongly turbulent convection but no LSC, and plumes on average rose vertically.

JFM classification

Phase change: Condensation/evaporation Drops and Bubbles: Drops and Bubbles
Type
Papers
Information
Journal of Fluid Mechanics , Volume 795 , 25 May 2016 , pp. 60 - 95
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G. 1995 Experiments on Thermally Driven Convection. Springer.Google Scholar
Ahlers, G. 2009 Turbulent convection. Physics 2, 74, 1–7.Google Scholar
Ahlers, G., Bodenschatz, E., Funfschilling, D., Grossmann, S., He, X., Lohse, D., Stevens, R. J. A. M. & Verzicco, R. 2012 Logarithmic temperature profiles in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 109, 114501.CrossRefGoogle ScholarPubMed
Ahlers, G., Bodenschatz, E. & He, X. 2014 Logarithmic temperature profiles of turbulent Rayleigh–Bénared convection in the classical and ultimate state for a Prandtl number of 0.8. J. Fluid Mech. 758, 436–467.CrossRefGoogle Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.CrossRefGoogle Scholar
Ahlers, G. & Xu, X. 2000 Prandtl-number dependence of heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 86, 3320–3323.Google Scholar
Baltis, C. H. M. & van der Geld, C. W. M. 2015 Heat transfer mechanisms of a vapour bubble growing at a wall in saturated upward flow. J. Fluid Mech. 771, 264–302.Google Scholar
Biferale, L., Perlekar, P., Sbragaglia, M. & Toschi, F. 2012 Convection in multiphase fluid flows using lattice Boltzmann methods. Phys. Rev. Lett. 108, 104502, 1–5.Google Scholar
Brown, E. & Ahlers, G. 2007 Large-scale circulation model of turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 98, 134501, 1–4.Google Scholar
Brown, E. & Ahlers, G. 2008 A model of diffusion in a potential well for the dynamics of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Fluids 20, 075101, 1–16.Google Scholar
de Bruyn, J. R., Bodenschatz, E., Morris, S. W., Trainoff, S., Hu, Y., Cannell, D. S. & Ahlers, G. 1996 Apparatus for the study of Rayleigh–Bénard convection in gases under pressure. Rev. Sci. Instrum. 67, 2043.Google Scholar
Busse, F. H. & Whitehead, J. A. 1971 Instabilities of convection rolls in a high Prandtl number fluid. J. Fluid Mech. 47, 305–320.CrossRefGoogle Scholar
Chen, Y. M. & Mayinger, F. 1992 Measurements of heat transfer at the phase interface of condensing bubbles. Intl J. Multiphase Flow 18, 877–890.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58, 1–25.Google Scholar
Dhir, V. K. 1998 Boiling heat transfer. Annu. Rev. Fluid Mech. 30, 365–401.Google Scholar
Forster, H. K. & Zuber, N. 1954 Growth of vapor bubbles in superheated liquid. J. Appl. Phys. 25 (4), 474–478.Google Scholar
Fritz, W. 1935 Berechnung des Maximalvolumens von Dampfblasen. Phys. Z. 11, 379–385.Google Scholar
Funfschilling, D., Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical cells with aspect ratio one and larger. J. Fluid Mech. 536, 145–154.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16, 4462–4472.Google Scholar
Kadanoff, L. P. 2001 Turbulent heat flow: structures and scaling. Phys. Today 54 (8), 34–39.Google Scholar
Kim, J. 2009 Review of nucleate pool boiling bubble heat transfer mechanism. Intl J. Multiphase Flow 35, 1067–1076.CrossRefGoogle Scholar
Lakkaraju, R., Schmidt, L. E., Oresta, P., Toschi, F., Verzicco, R., Lohse, D. & Prosperetti, A. 2011 Effect of vapor bubbles on velocity fluctuations and dissipation rates in bubble Rayleigh–Bénard convection. Phys. Rev. E 84, 036312, 1–7.Google Scholar
Lakkaraju, R., Stevens, R. J. A. M., Oresta, P., Verzicco, R., Lohse, D. & Prosperetti, A. 2013 Heat transport in bubbling turbulent convection. Proc. Natl Acad. Sci. USA 110, 9237–9242.Google Scholar
Lakkaraju, R., Toschi, F. & Lohse, D. 2014 Bubbling reduces intermittency in turbulent thermal convection. J. Fluid Mech. 745, 1–24.Google Scholar
Legendre, D., Borée, J. & Magnaudet, J. 1998 Thermal and dynamic evolution of a spherical bubble moving steadily in a superheated or subcooled liquid. Phys. Fluids 10 (6), 1256–1272.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Lohse, D. & Zhang, X. 2015 Surface nanobubble and surface nanodroplets. Rev. Mod. Phys. 87, 981–1035.Google Scholar
Magnaudet, J. & Eames, I. 2000 The motion of high-Reynolds-number bubbles in imhomogeneous flows. Annu. Rev. Fluid Mech. 32, 659–708.Google Scholar
Moore, D. W. 1965 The velocity of rise of distorted gas bubbles in a liquid of small viscosity. J. Fluid Mech. 23, 749–766.Google Scholar
Murphy, R. W. & Bergles, A. E. 1972 Subcooled flow boiling of fluorocarbons – hysteresis and dissolved gas effects on heat transfer. In Proceedings of the Heat Transfer and Fluid Mechanics, pp. 400–416. Stanford University Press.Google Scholar
Narezo Guzman, D., Xie, Y., Chen, S., Fernandez Rivas, D., Sun, C., Lohse, D. & Ahlers, G. 2015 Heat flux enhancement by vapour-bubble nucleation in Rayleigh–Bénard turbulence. J. Fluid Mech. 787, 331–366.Google Scholar
Og̃uz, H. N. & Prosperetti, A. 1993 Dynamics of bubble growth and detachment from a needle. J. Fluid Mech. 257, 111–145.Google Scholar
Ohl, C. D., Tijink, A. & Prosperetti, A. 2003 The added mass of an expanding bubble. J. Fluid Mech. 482, 271–290.Google Scholar
Okhotsimskii, A. D. 1988 The thermal regime of vapour bubble collapse at different Jacob numbers. Intl J. Heat Mass Transfer 31, 1569–1576.Google Scholar
Oresta, P., Verzicco, R., Lohse, D. & Properetti, A. 2009 Heat transfer mechanisms in bubbly Rayleigh–Bénard convection. Phy. Rev. E 80, 026304, 1–11.Google Scholar
Plesset, M. S. & Zwick, S. A. 1954 The growth of vapor bubbles in superheated liquids. J. Appl. Phys. 25, 493–500.Google Scholar
Prosperetti, A. & Plesset, M. S. 1978 Vapour-bubble growth in a superheated liquid. J. Fluid Mech. 85, 349–368.Google Scholar
Qiu, X. L. & Tong, P. 2001 Large scale velocity structures in turbulent thermal convection. Phys. Rev. E 64, 036304, 1–13.Google Scholar
Rasenat, S., Hartung, G., Winkler, B. L. & Rehberg, I. 1989 The shadowgraph method in convection experiments. Exp. Fluids 7, 412–420.Google Scholar
Sakurai, S., Tschammer, A., Pesch, W. & Ahlers, G. 1999 Convection in the presence of a first-order phase change. Phys. Rev. E 60, 539–550.Google Scholar
Schmidt, L. E., Oresta, P., Toschi, F., Verzicco, R., Lohse, D. & Prosperetti, A. 2011 Modification of turbulence in Rayleigh–Bénard convection by phase change. New J. Phys. 13, 025002, 1–10.Google Scholar
Shpak, O., Stricker, L., Versluis, M. & Lohse, D. 2013 The role of gas in ultrasonically driven vapor bubble growth. Phys. Med. Biol. 58, 2523–2535.Google Scholar
Sluyter, W. M., Slooten, P. C., Copraji, C. A. & Chesters, A. K. 1991 The departure size of pool-boiling bubbles from artificial cavities at moderate and high pressures. Intl J. Multiphase Flow 17, 153–158.Google Scholar
Steinke, M. E. & Kandlikar, S. G. 2004 Control and dissolved air in water during flow boiling in micro channels. Intl J. Heat Mass Transfer 47, 1925–1935.CrossRefGoogle Scholar
Trainoff, S. P. & Cannel, D. S. 2002 Physical optics treatment of the shadowgraph. Phys. Fluids 14, 1340–1363.Google Scholar
de Vries, J., Luther, S. & Lohse, D. 2002 Induced bubble shape oscillations and their impact on the rise velocity. Eur. Phys. J. B 29, 503–509.CrossRefGoogle Scholar
Wei, P. & Ahlers, G. 2014 Logarithmic temperature profiles in the bulk of turbulent Rayleigh–Bénard convection for a Prandtl number of 12.3. J. Fluid Mech. 758, 809–830.Google Scholar
Xi, H. D., Lam, S. & Xia, K. Q. 2004 From laminar plumes to organized flows: the onset of large-scale circulation in turbulent thermal convection. J. Fluid Mech. 503, 47–56.Google Scholar
Xi, H. D. & Xia, K. Q. 2008 Azimuthal motion, reorientation, cessation, and reversal of the large-scale circulation in turbulent thermal convection: a comparative study in aspect ratio one and one-half geometries. Phys. Rev. E 78, 036326.Google Scholar
Xu, X., Bajaj, K. M. S. & Ahlers, G. 2000 Heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 84 (19), 4357–4360.Google Scholar
Yabuki, T. & Nakabeppu, O.2011 Heat transfer characteristics of isolated bubble nucleate boiling of water. In Proceedings of the ASME/JSME 2011 8th Thermal Engineering Joint Conference. pp. T10196.Google Scholar
Yang, B. & Prosperetti, A. 2008 Vapour-bubble collapse in isothermal and non-isothermal liquids. J. Fluid Mech. 601, 253–279.Google Scholar
Zhang, X., Lu, Z., Tan, H., Bao, L., He, Y., Sun, C. & Lohse, D. 2015 Formation of surface nanodroplets under controlled flow conditions. Proc. Natl Acad. Sci. USA 112, 9253–9257.CrossRefGoogle ScholarPubMed
Zhong, J. Q., Funfschilling, D. & Ahlers, G. 2009 Enhanced heat transport by turbulent two-phase Rayleigh–Bénard convection. Phys. Rev. Lett. 102, 124501, 1–4.Google Scholar

Narezo Guzman et al. supplementary movie

Movie from which figure 5(a) was extracted. The movie shows the bubble detachment process from the bottom plate with superheat of about 10 K and a cavity separation of 0.6 mm. The movie was recorded at 500 frames per second and was slowed down 20 times for display.

Download Narezo Guzman et al. supplementary movie(Video)
Video 9.8 MB

Narezo Guzman et al. supplementary movie

Movie from which figure 5(b) was extracted. The movie shows the detached bubbles rising across 0.4 of the cell height. The bottom plate superheat was about 10 K and the cavity separation was 0.6 mm. The movie was recorded at 1000 frames per second and was slowed down 40 times for display.

Download Narezo Guzman et al. supplementary movie(Video)
Video 9.9 MB

Narezo Guzman et al. supplementary movie

Shadowgraph visualization of two-phase flow capturing the entire cell between bottom to top plates. Movie from which figure 6(a) was extracted. Movie is displayed in real time. The bottom plate superheat was about 10 K and the cavity separation was 0.6 mm.

Download Narezo Guzman et al. supplementary movie(Video)
Video 7.4 MB

Narezo Guzman et al. supplementary movie

Shadowgraph visualization of one-phase flow capturing the entire cell between bottom to top plates. Movie from which figure 6(b) was extracted. Movie is displayed in real time. The bottom plate superheat was about 10 K and the cavity separation was 0.6 mm.

Download Narezo Guzman et al. supplementary movie(Video)
Video 7.4 MB

Narezo Guzman et al. supplementary movie

Movie from which figure 8(d) was extracted. The movie shows bubbles detaching from the bottom plate and rising until eventually they fully condense and dissolve. The movie captured the flow between bottom and top plates and was recorded at 500 frames per second and slowed down 20 times for display. The bottom plate superheat was about 10 K and the cavity separation was 0.6 mm.

Download Narezo Guzman et al. supplementary movie(Video)
Video 10.4 MB