Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
de Silva, C. M.
Marusic, I.
Woodcock, J. D.
and
Meneveau, C.
2015.
Scaling of second- and higher-order structure functions in turbulent boundary layers.
Journal of Fluid Mechanics,
Vol. 769,
Issue. ,
p.
654.
Birnir, Björn
and
Chen, Xi
2016.
Sub-Gaussian behavior of the Townsend-Perry constants in turbulent boundary layers.
Physical Review E,
Vol. 93,
Issue. 1,
Pan, Y.
and
Chamecki, M.
2016.
A scaling law for the shear-production range of second-order structure functions.
Journal of Fluid Mechanics,
Vol. 801,
Issue. ,
p.
459.
Ebner, R. L.
Mehdi, Faraz
and
Klewicki, J. C.
2016.
Shared dynamical features of smooth- and rough-wall boundary-layer turbulence.
Journal of Fluid Mechanics,
Vol. 792,
Issue. ,
p.
435.
Yang, Xiang I. A.
Marusic, Ivan
and
Meneveau, Charles
2016.
Moment generating functions and scaling laws in the inertial layer of turbulent wall-bounded flows.
Journal of Fluid Mechanics,
Vol. 791,
Issue. ,
Mouri, Hideaki
2017.
Two-point correlation in wall turbulence according to the attached-eddy hypothesis.
Journal of Fluid Mechanics,
Vol. 821,
Issue. ,
p.
343.
Chamecki, Marcelo
Dias, Nelson L.
Salesky, Scott T.
and
Pan, Ying
2017.
Scaling Laws for the Longitudinal Structure Function in the Atmospheric Surface Layer.
Journal of the Atmospheric Sciences,
Vol. 74,
Issue. 4,
p.
1127.
Dias, Nelson Luís
Crivellaro, Bianca Luhm
and
Chamecki, Marcelo
2018.
The Hurst Phenomenon in Error Estimates Related to Atmospheric Turbulence.
Boundary-Layer Meteorology,
Vol. 168,
Issue. 3,
p.
387.
Brugger, Peter
Katul, Gabriel G.
De Roo, Frederik
Kröniger, Konstantin
Rotenberg, Eyal
Rohatyn, Shani
and
Mauder, Matthias
2018.
Scalewise invariant analysis of the anisotropic Reynolds stress tensor for atmospheric surface layer and canopy sublayer turbulent flows.
Physical Review Fluids,
Vol. 3,
Issue. 5,
Chamecki, Marcelo
Dias, Nelson L.
and
Freire, Livia S.
2018.
A TKE‐Based Framework for Studying Disturbed Atmospheric Surface Layer Flows and Application to Vertical Velocity Variance Over Canopies.
Geophysical Research Letters,
Vol. 45,
Issue. 13,
p.
6734.
Ghannam, Khaled
Katul, Gabriel G.
Bou-Zeid, Elie
Gerken, Tobias
and
Chamecki, Marcelo
2018.
Scaling and Similarity of the Anisotropic Coherent Eddies in Near-Surface Atmospheric Turbulence.
Journal of the Atmospheric Sciences,
Vol. 75,
Issue. 3,
p.
943.
Polanco, J.I.
Vinkovic, I.
Stelzenmuller, N.
Mordant, N.
and
Bourgoin, M.
2018.
Relative dispersion of particle pairs in turbulent channel flow.
International Journal of Heat and Fluid Flow,
Vol. 71,
Issue. ,
p.
231.
Dias, Nelson Luís
2018.
Smoothed Spectra, Ogives, and Error Estimates for Atmospheric Turbulence Data.
Boundary-Layer Meteorology,
Vol. 166,
Issue. 1,
p.
1.
Wang, C.
and
Anderson, W.
2019.
Turbulence Coherence Within Canonical and Realistic Aeolian Dune-Field Roughness Sublayers.
Boundary-Layer Meteorology,
Vol. 173,
Issue. 3,
p.
409.
Han, Guo Wen
Liu, Li
Bo, Tian Li
and
Zheng, Xiao Jing
2019.
A Predictive Model for the Streamwise Velocity in the Near‐Neutral Atmospheric Surface Layer.
Journal of Geophysical Research: Atmospheres,
Vol. 124,
Issue. 1,
p.
238.
Freire, Livia S.
Dias, Nelson L.
and
Chamecki, Marcelo
2019.
Effects of Path Averaging in a Sonic Anemometer on the Estimation of Turbulence-Kinetic-Energy Dissipation Rates.
Boundary-Layer Meteorology,
Vol. 173,
Issue. 1,
p.
99.
Berg, Jacob
Patton, Edward G.
and
Sullivan, Peter P.
2020.
Large-Eddy Simulation of Conditionally Neutral Boundary Layers: A Mesh Resolution Sensitivity Study.
Journal of the Atmospheric Sciences,
Vol. 77,
Issue. 6,
p.
1969.
Zhang, Wen
Wan, Minping
Xia, Zhenhua
Wang, Jianchun
Lu, Xiyun
and
Chen, Shiyi
2021.
Constrained large-eddy simulation of turbulent flow over rough walls.
Physical Review Fluids,
Vol. 6,
Issue. 4,
Stiperski, Ivana
Chamecki, Marcelo
and
Calaf, Marc
2021.
Anisotropy of Unstably Stratified Near-Surface Turbulence.
Boundary-Layer Meteorology,
Vol. 180,
Issue. 3,
p.
363.
Heisel, Michael
de Silva, Charitha M.
Katul, Gabriel G.
and
Chamecki, Marcelo
2022.
Self-similar geometries within the inertial subrange of scales in boundary layer turbulence.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
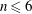 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}n \leq 6$) in the range of scales
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}n \leq 6$) in the range of scales 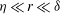 $\eta \ll r \ll \delta $, where
$\eta \ll r \ll \delta $, where 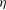 $\eta $ is the Kolmogorov length and
$\eta $ is the Kolmogorov length and  $\delta $ is the boundary layer thickness. This theory rests on the hypothesis that the turbulence in this intermediate range of scales depends only on the scale
$\delta $ is the boundary layer thickness. This theory rests on the hypothesis that the turbulence in this intermediate range of scales depends only on the scale  $r$, the local dissipation rate and the shear velocity. Crucially, the structure of the turbulence is assumed to be independent of the distance from the wall,
$r$, the local dissipation rate and the shear velocity. Crucially, the structure of the turbulence is assumed to be independent of the distance from the wall, 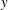 $y$, except to the extent that
$y$, except to the extent that  $y$ sets the value of the local dissipation rate. A detailed comparison is made between the predictions of the theory and data taken from both smooth- and rough-wall boundary layers. The data support the hypothesis that it is the dissipation rate, and not
$y$ sets the value of the local dissipation rate. A detailed comparison is made between the predictions of the theory and data taken from both smooth- and rough-wall boundary layers. The data support the hypothesis that it is the dissipation rate, and not 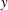 $y$, that controls the structure of the turbulence for this range of eddy sizes. Our findings provide the first unified scaling theory for both smooth- and rough-wall turbulence.
$y$, that controls the structure of the turbulence for this range of eddy sizes. Our findings provide the first unified scaling theory for both smooth- and rough-wall turbulence.