Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hassanzadeh, Pedram
Marcus, Philip S.
and
Le Gal, Patrice
2012.
The universal aspect ratio of vortices in rotating stratified flows: theory and simulation.
Journal of Fluid Mechanics,
Vol. 706,
Issue. ,
p.
46.
Chan, Kwing L.
and
Mayr, Hans G.
2013.
Numerical simulation of convectively generated vortices: Application to the Jovian planets.
Earth and Planetary Science Letters,
Vol. 371-372,
Issue. ,
p.
212.
Aubert, O.
Le Bars, M.
Le Gal, P.
Marcus, P. S.
Barge, P.
and
Jorda, L.
2013.
Vortices in rotating and stratified flows: aspect ratio and sustainability.
EPJ Web of Conferences,
Vol. 46,
Issue. ,
p.
05004.
Marcus, Philip S.
Asay-Davis, Xylar
Wong, Michael H.
and
de Pater, Imke
2013.
Jupiter’s Red Oval BA: Dynamics, Color, and Relationship to Jovian Climate Change.
Journal of Heat Transfer,
Vol. 135,
Issue. 1,
Marcus, Philip S.
Pei, Suyang
Jiang, Chung-Hsiang
and
Hassanzadeh, Pedram
2013.
Three-Dimensional Vortices Generated by Self-Replication in Stably Stratified Rotating Shear Flows.
Physical Review Letters,
Vol. 111,
Issue. 8,
Palotai, Csaba
Dowling, Timothy E.
and
Fletcher, Leigh N.
2014.
3D Modeling of interactions between Jupiter’s ammonia clouds and large anticyclones.
Icarus,
Vol. 232,
Issue. ,
p.
141.
Kurien, Susan
and
Smith, Leslie M.
2014.
Effect of rotation and domain aspect-ratio on layer formation in strongly stratified Boussinesq flows.
Journal of Turbulence,
Vol. 15,
Issue. 4,
p.
241.
Rosenberg, D.
Pouquet, A.
Marino, R.
and
Mininni, P. D.
2015.
Evidence for Bolgiano-Obukhov scaling in rotating stratified turbulence using high-resolution direct numerical simulations.
Physics of Fluids,
Vol. 27,
Issue. 5,
Ungarish, Marius
2015.
On the coupling between spin-up and aspect ratio of vortices in rotating stratified flows: a predictive model.
Journal of Fluid Mechanics,
Vol. 777,
Issue. ,
p.
461.
Facchini, Giulio
and
Le Bars, Michael
2016.
On the lifetime of a pancake anticyclone in a rotating stratified flow.
Journal of Fluid Mechanics,
Vol. 804,
Issue. ,
p.
688.
Yim, Eunok
Billant, Paul
and
Ménesguen, Claire
2016.
Stability of an isolated pancake vortex in continuously stratified-rotating fluids.
Journal of Fluid Mechanics,
Vol. 801,
Issue. ,
p.
508.
Ungarish, M.
Johnson, C.G.
and
Hogg, A.J.
2016.
Sustained axisymmetric intrusions in a rotating system.
European Journal of Mechanics - B/Fluids,
Vol. 56,
Issue. ,
p.
110.
Mahdinia, Mani
Hassanzadeh, Pedram
Marcus, Philip S.
and
Jiang, Chung-Hsiang
2017.
Stability of three-dimensional Gaussian vortices in an unbounded, rotating, vertically stratified, Boussinesq flow: linear analysis.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
97.
Ciani, D.
Carton, X.
Barbosa Aguiar, A.C.
Peliz, A.
Bashmachnikov, I.
Ienna, F.
Chapron, B.
and
Santoleri, R.
2017.
Surface signature of Mediterranean water eddies in a long-term high-resolution simulation.
Deep Sea Research Part I: Oceanographic Research Papers,
Vol. 130,
Issue. ,
p.
12.
De la Rosa Zambrano, H.M.
Cros, A.
Cruz Gómez, R.
Le Bars, M.
and
Le Gal, P.
2017.
A laboratory study of floating lenticular anticyclones.
European Journal of Mechanics - B/Fluids,
Vol. 61,
Issue. ,
p.
1.
Zhai, X. M.
and
Kurien, Susan
2018.
Characteristic length scales of strongly rotating Boussinesq flow in variable-aspect-ratio domains.
Journal of Fluid Mechanics,
Vol. 856,
Issue. ,
p.
397.
Capuano, Tonia Astrid
Speich, Sabrina
Carton, Xavier
and
Laxenaire, Remi
2018.
Indo‐Atlantic Exchange, Mesoscale Dynamics, and Antarctic Intermediate Water.
Journal of Geophysical Research: Oceans,
Vol. 123,
Issue. 5,
p.
3286.
Orozco Estrada, A.
Cruz Gómez, Raúl C.
Cros, A.
and
Le Gal, P.
2020.
Coalescence of lenticular anticyclones in a linearly stratified rotating fluid.
Geophysical & Astrophysical Fluid Dynamics,
Vol. 114,
Issue. 4-5,
p.
504.
Liu, ZhiYing
Liao, GuangHong
Hu, XiaoKai
and
Zhou, BeiFeng
2020.
Aspect Ratio of Eddies Inferred From Argo Floats and Satellite Altimeter Data in the Ocean.
Journal of Geophysical Research: Oceans,
Vol. 125,
Issue. 1,
Galperin, Boris
and
Sukoriansky, Semion
2020.
Quasinormal scale elimination theory of the anisotropic energy spectra of atmospheric and oceanic turbulence.
Physical Review Fluids,
Vol. 5,
Issue. 6,
 of vortices in a rotating, stratified flow, where
of vortices in a rotating, stratified flow, where 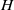 and
and 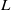 are the vertical half-height and horizontal length scale of the vortices. The aspect ratio depends not only on the Coriolis parameter
are the vertical half-height and horizontal length scale of the vortices. The aspect ratio depends not only on the Coriolis parameter 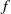 and buoyancy (or Brunt–Väisälä) frequency
and buoyancy (or Brunt–Väisälä) frequency  of the background flow, but also on the buoyancy frequency
of the background flow, but also on the buoyancy frequency  within the vortex and on the Rossby number
within the vortex and on the Rossby number  of the vortex, such that
of the vortex, such that  . This law for
. This law for  is obeyed precisely by the exact equilibrium solution of the inviscid Boussinesq equations that we show to be a useful model of our laboratory vortices. The law is valid for both cyclones and anticyclones. Our anticyclones are generated by injecting fluid into a rotating tank filled with linearly stratified salt water. In one set of experiments, the vortices viscously decay while obeying our law for
is obeyed precisely by the exact equilibrium solution of the inviscid Boussinesq equations that we show to be a useful model of our laboratory vortices. The law is valid for both cyclones and anticyclones. Our anticyclones are generated by injecting fluid into a rotating tank filled with linearly stratified salt water. In one set of experiments, the vortices viscously decay while obeying our law for  , which decreases over time. In a second set of experiments, the vortices are sustained by a slow continuous injection. They evolve more slowly and have larger
, which decreases over time. In a second set of experiments, the vortices are sustained by a slow continuous injection. They evolve more slowly and have larger 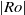 while still obeying our law for
while still obeying our law for  . The law for
. The law for  is not only validated by our experiments, but is also shown to be consistent with observations of the aspect ratios of Atlantic meddies and Jupiter’s Great Red Spot and Oval BA. The relationship for
is not only validated by our experiments, but is also shown to be consistent with observations of the aspect ratios of Atlantic meddies and Jupiter’s Great Red Spot and Oval BA. The relationship for  is derived and examined numerically in a companion paper by Hassanzadeh, Marcus & Le Gal (J. Fluid Mech., vol. 706, 2012, pp. 46–57).
is derived and examined numerically in a companion paper by Hassanzadeh, Marcus & Le Gal (J. Fluid Mech., vol. 706, 2012, pp. 46–57).