Article contents
Understanding liquid-jet atomization cascades via vortex dynamics
Published online by Cambridge University Press: 21 March 2018
Abstract
Temporal instabilities of a planar liquid jet are studied using direct numerical simulation (DNS) of the incompressible Navier–Stokes equations with level-set (LS) and volume-of-fluid (VoF) surface tracking methods.  $\unicode[STIX]{x1D706}_{2}$ contours are used to relate the vortex dynamics to the surface dynamics at different stages of the jet breakup – namely, lobe formation, lobe perforation, ligament formation, stretching and tearing. Three distinct breakup mechanisms are identified in the primary breakup, which are well categorized on the parameter space of gas Weber number (
$\unicode[STIX]{x1D706}_{2}$ contours are used to relate the vortex dynamics to the surface dynamics at different stages of the jet breakup – namely, lobe formation, lobe perforation, ligament formation, stretching and tearing. Three distinct breakup mechanisms are identified in the primary breakup, which are well categorized on the parameter space of gas Weber number (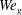 $We_{g}$) versus liquid Reynolds number (
$We_{g}$) versus liquid Reynolds number ( $Re_{l}$). These mechanisms are analysed here from a vortex dynamics perspective. Vortex dynamics explains the hairpin formation, and the interaction between the hairpins and the Kelvin–Helmholtz (KH) roller explains the perforation of the lobes, which is attributed to the streamwise overlapping of two oppositely oriented hairpin vortices on top and bottom of the lobe. The formation of corrugations on the lobe front edge at high
$Re_{l}$). These mechanisms are analysed here from a vortex dynamics perspective. Vortex dynamics explains the hairpin formation, and the interaction between the hairpins and the Kelvin–Helmholtz (KH) roller explains the perforation of the lobes, which is attributed to the streamwise overlapping of two oppositely oriented hairpin vortices on top and bottom of the lobe. The formation of corrugations on the lobe front edge at high 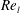 $Re_{l}$ is also related to the location and structure of the hairpins with respect to the KH vortex. The lobe perforation and corrugation formation are inhibited at low
$Re_{l}$ is also related to the location and structure of the hairpins with respect to the KH vortex. The lobe perforation and corrugation formation are inhibited at low 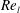 $Re_{l}$ and low
$Re_{l}$ and low 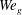 $We_{g}$ due to the high surface tension and viscous forces, which damp the small-scale corrugations and resist hole formation. Streamwise vorticity generation – resulting in three-dimensional instabilities – is mainly caused by vortex stretching and baroclinic torque at high and low density ratios, respectively. Generation of streamwise vortices and their interaction with spanwise vortices produce the liquid structures seen at various flow conditions. Understanding the liquid sheet breakup and the related vortex dynamics are crucial for controlling the droplet-size distribution in primary atomization.
$We_{g}$ due to the high surface tension and viscous forces, which damp the small-scale corrugations and resist hole formation. Streamwise vorticity generation – resulting in three-dimensional instabilities – is mainly caused by vortex stretching and baroclinic torque at high and low density ratios, respectively. Generation of streamwise vortices and their interaction with spanwise vortices produce the liquid structures seen at various flow conditions. Understanding the liquid sheet breakup and the related vortex dynamics are crucial for controlling the droplet-size distribution in primary atomization.
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 44
- Cited by


