Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T02:44:37.622Z Has data issue: false hasContentIssue false
  • English
  • Français

Two-way coupled turbulent particle-laden boundary layer combustion over a flat plate

Published online by Cambridge University Press:  06 September 2022

Guo Chen ,
Haiou Wang Open the ORCID record for Haiou Wang [Opens in a new window] ,
Kun Luo  and
Jianren Fan Open the ORCID record for Jianren Fan [Opens in a new window]
Guo Chen
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Haiou Wang*
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Kun Luo
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
Jianren Fan
Affiliation:
State Key Laboratory of Clean Energy Utilization, Zhejiang University, Hangzhou 310027, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

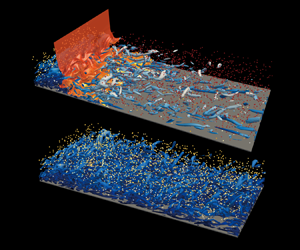
In the present study, turbulent particle-laden boundary layer combustion over a flat plate is investigated using direct numerical simulation (DNS). A two-way coupled Eulerian–Lagrangian point particle method is used for the solid phase. The effects of particle Stokes numbers, mass loadings and chemical reactions on the interactions between particles and boundary layer turbulence in the near-wall region are explored. It was found that particle heat transfer is dominant over wall heat transfer in the reacting case with heavy particles and large mass loadings, resulting in a lower fluid temperature. Particle accumulation due to the turbophoresis effect in the near-wall region is observed, which is more prominent in the cases with a large Stokes number. The turbophoresis effect is examined via the magnitude of streamwise vorticity $\varOmega _x$. It is shown that $\varOmega _x$ is attenuated by heavy particles, and the attenuation increases with increasing mass loadings. Therefore, particle wall–accumulation is less prominent in the cases with large mass loadings. Compared with the non-reacting cases, the distribution of particles is more inhomogeneous for the reacting cases, where the particles move faster due to intense reactions with increasing wall-normal distance. Finally, the flow topologies and the Reynolds stress anisotropy are examined to understand turbulence modulation by combustion and particles. It was suggested that light particles augment the vortex-dominant topologies, whereas heavy particles have an opposite effect. The anisotropy mapping of the Reynolds stress shows that the flows become more one-dimensional in the near-wall region for the cases with combustion and/or large mass loadings.

JFM classification

Reacting Flows: Turbulent reacting flows Turbulent Flows: Turbulent boundary layers Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 948 , 10 October 2022 , A12
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alshaalan, T. & Rutland, C.J. 2002 Wall heat flux in turbulent premixed reacting flow. Combust. Sci. Technol. 174, 135–165.CrossRefGoogle Scholar
Armenio, V. & Fiorotto, V. 2001 The importance of the forces acting on particles in turbulent flows. Phys. Fluids 13, 2437–2440.CrossRefGoogle Scholar
Balachandar, S. & Eaton, J.K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.CrossRefGoogle Scholar
Battista, F., Mollicone, J.-P., Gualtieri, P., Messina, R. & Casciola, C.M. 2019 Exact regularised point particle (erpp) method for particle-laden wall-bounded flows in the two-way coupling regime. J. Fluid Mech. 878, 420–444.CrossRefGoogle Scholar
Battista, F., Picano, F., Troiani, G. & Casciola, C.M. 2011 Intermittent features of inertial particle distributions in turbulent premixed flames. Phys. Fluids 23, 123304.CrossRefGoogle Scholar
Bijlard, M.J., Oliemans, R.V.A., Portela, L.M. & Ooms, G. 2010 Direct numerical simulation analysis of local flow topology in a particle-laden turbulent channel flow. J. Fluid Mech. 653, 35–56.CrossRefGoogle Scholar
Borghesi, G., Mastorakos, E. & Cant, R.S. 2013 Complex chemistry DNS of n-heptane spray autoignition at high pressure and intermediate temperature conditions. Combust. Flame 160, 1254–1275.CrossRefGoogle Scholar
Brock, J.R. 1967 The thermal force in the transition region. J. Colloid Interface Sci. 23 (3), 448–452.CrossRefGoogle Scholar
Brooke, J.W., Hanratty, T.J. & McLaughlin, J.B. 1994 Free-flight mixing and deposition of aerosols. Phys. Fluids 6, 3404–3415.CrossRefGoogle Scholar
Bruneaux, G., Akselvoll, K., Poinsot, T. & Ferziger, J.H. 1996 Flame-wall interaction simulation in a turbulent channel flow. Combust. Flame 107, 27–36.CrossRefGoogle Scholar
Bruneaux, G., Poinsot, T. & Ferziger, J.H. 1997 Premixed flame–wall interaction in a turbulent channel flow : budget for the flame surface density evolution equation and modelling. J. Fluid Mech. 349, 191–219.CrossRefGoogle Scholar
Capecelatro, J. & Desjardins, O. 2013 An Euler–Lagrange strategy for simulating particle-laden flows. J. Comput. Phys. 238, 1–31.CrossRefGoogle Scholar
Capecelatro, J., Desjardins, O. & Fox, R.O. 2016 Strongly coupled fluid-particle flows in vertical channels. I. Reynolds-averaged two-phase turbulence statistics. Phys. Fluids 28, 033306.CrossRefGoogle Scholar
Capecelatro, J., Desjardins, O. & Fox, R.O. 2018 On the transition between turbulence regimes in particle-laden channel flows. J. Fluid Mech. 845, 499–519.CrossRefGoogle Scholar
Chen, J.H., et al. 2009 Terascale direct numerical simulations of turbulent combustion using S3D. Comput. Sci. Disc. 2, 015001.CrossRefGoogle Scholar
Chen, G., Wang, H., Luo, K. & Fan, J. 2021 Flame edge structures and dynamics in planar turbulent non-premixed inclined slot-jet flames impinging at a wall. J. Fluid Mech. 920, A43.CrossRefGoogle Scholar
Chong, M.S., Perry, A.E. & Cantwell, B.J. 1990 A general classification of three-dimensional flow fields. Phys. Fluids A 2, 765–777.CrossRefGoogle Scholar
Costa, P., Brandt, L. & Picano, F. 2020 Interface-resolved simulations of small inertial particles in turbulent channel flow. J. Fluid Mech. 883, A54.CrossRefGoogle Scholar
Costa, P., Brandt, L. & Picano, F. 2021 Near-wall turbulence modulation by small inertial particles. J. Fluid Mech. 922, A9.CrossRefGoogle Scholar
Crowe, C.T. 2000 On models for turbulence modulation in fluid–particle flows. Intl J. Multiphase Flow 26, 719–727.CrossRefGoogle Scholar
Dreizler, A. & Böhm, B. 2015 Advanced laser diagnostics for an improved understanding of premixed flame–wall interactions. Proc. Combust. Inst. 35, 37–64.CrossRefGoogle Scholar
Driscoll, J.F., Chen, J.H., Skiba, A.W., Carter, C.D., Hawkes, E.R. & Wang, H. 2020 Premixed flames subjected to extreme turbulence: some questions and recent answers. Prog. Energy Combust. Sci. 76, 100802.CrossRefGoogle Scholar
Dritselis, C.D. & Vlachos, N.S. 2008 Numerical study of educed coherent structures in the near-wall region of a particle-laden channel flow. Phys. Fluids 20, 55103.CrossRefGoogle Scholar
Dritselis, C.D. & Vlachos, N.S. 2011 Numerical investigation of momentum exchange between particles and coherent structures in low re turbulent channel flow. Phys. Fluids 23, 25103.CrossRefGoogle Scholar
Elghobashi, S. 1994 On predicting particle-laden turbulent flows. Appl. Sci. Res. 52, 309–329.CrossRefGoogle Scholar
Elghobashi, S. 2006 An updated classification map of particle-laden turbulent flows. In IUTAM Symposium on Computational Approaches to Multiphase Flow (ed. S. Balachandar & A. Prosperetti), pp. 3–10. Springer.CrossRefGoogle Scholar
Elghobashi, S. & Truesdell, G.C. 1993 On the two-way interaction between homogeneous turbulence and dispersed solid particles. I: turbulence modification. Phys. Fluids A 5, 1790–1801.CrossRefGoogle Scholar
Fessler, J.R., Kulick, J.D. & Eaton, J.K. 1994 Preferential concentration of heavy particles in a turbulent channel flow. Phys. Fluids 6, 3742–3749.CrossRefGoogle Scholar
Fox, R.O. 2014 On multiphase turbulence models for collisional fluid–particle flows. J. Fluid Mech. 742, 368–424.CrossRefGoogle Scholar
Ganapathisubramani, B., Clemens, N.T. & Dolling, D.S. 2006 Large-scale motions in a supersonic turbulent boundary layer. J. Fluid Mech. 556, 271–282.CrossRefGoogle Scholar
Gore, R.A. & Crowe, C.T. 1989 Effect of particle size on modulating turbulent intensity. Intl J. Multiphase Flow 15, 279–285.CrossRefGoogle Scholar
Gruber, A., Sankaran, R., Hawkes, E.R. & Chen, J.H. 2010 Turbulent flame–wall interaction: a direct numerical simulation study. J. Fluid Mech. 658, 5–32.CrossRefGoogle Scholar
Gualtieri, P., Picano, F., Sardina, G. & Casciola, C.M. 2015 Exact regularized point particle method for multiphase flows in the two-way coupling regime. J. Fluid Mech. 773, 520–561.CrossRefGoogle Scholar
Hawkes, E.R. & Chen, J.H. 2004 Direct numerical simulation of hydrogen-enriched lean premixed methane–air flames. Combust. Flame 138, 242–258.CrossRefGoogle Scholar
Hetsroni, G., Mosyak, A. & Pogrebnyak, E. 2002 Effect of coarse particles on the heat transfer in a particle-laden turbulent boundary layer. Intl J. Multiphase Flow 28, 1873–1894.CrossRefGoogle Scholar
James, R.B. 1962 On the theory of thermal forces acting on aerosol particles. J. Colloid Sci. 17, 768–780.Google Scholar
Kaftori, D., Hetsroni, G. & Banerjee, S. 1995 Particle behavior in the turbulent boundary layer. I. Motion, deposition, and entrainment. Phys. Fluids 7, 1095–1106.CrossRefGoogle Scholar
Kee, R.J., Rupley, F.M. & Miller, J.A. 1989 Chemkin-II: A Fortran chemical kinetics package for the analysis of gas-phase chemical kinetics (No. SAND-89-8009). Sandia National Lab. (SNL-CA).CrossRefGoogle Scholar
Kennedy, C.A. & Carpenter, M.H. 1994 Several new numerical methods for compressible shear-layer simulations. Appl. Numer. Maths 14, 397–433.CrossRefGoogle Scholar
Kennedy, C.A., Carpenter, M.H. & Lewis, R.M. 2000 Low-storage, explicit Runge–Kutta schemes for the compressible Navier–Stokes equations. Appl. Numer. Maths 35, 177–219.CrossRefGoogle Scholar
Kuerten, J.G.M. 2016 Point-particle DNS and LES of particle-laden turbulent flow – a state-of-the-art review. Flow Turbul. Combust. 97, 689–713.CrossRefGoogle Scholar
Kuerten, J.G.M., van der Geld, C.W.M. & Geurts, B.J. 2011 Turbulence modification and heat transfer enhancement by inertial particles in turbulent channel flow. Phys. Fluids 23, 123301.CrossRefGoogle Scholar
Kulick, J.D., Fessler, J.R. & Eaton, J.K. 1994 Particle response and turbulence modification in fully developed channel flow. J. Fluid Mech. 277, 109–134.CrossRefGoogle Scholar
Lee, J. & Lee, C. 2015 Modification of particle-laden near-wall turbulence: effect of Stokes number. Phys. Fluids 27, 23303.CrossRefGoogle Scholar
Li, D., Luo, K. & Fan, J. 2016 a Modulation of turbulence by dispersed solid particles in a spatially developing flat-plate boundary layer. J. Fluid Mech. 802, 359–394.CrossRefGoogle Scholar
Li, D., Luo, K. & Fan, J. 2018 Direct numerical simulation of turbulent flow and heat transfer in a spatially developing turbulent boundary layer laden with particles. J. Fluid Mech. 845, 417–461.CrossRefGoogle Scholar
Li, Y., McLaughlin, J.B., Kontomaris, K. & Portela, L. 2001 Numerical simulation of particle-laden turbulent channel flow. Phys. Fluids 13, 2957–2967.CrossRefGoogle Scholar
Li, D., Wei, A., Luo, K. & Fan, J. 2016 b Direct numerical simulation of a particle-laden flow in a flat plate boundary layer. Intl J. Multiphase Flow 79, 124–143.CrossRefGoogle Scholar
Li, J., Zhao, Z., Kazakov, A. & Dryer, F.L. 2004 An updated comprehensive kinetic model of hydrogen combustion. Intl J. Chem. Kinet. 36, 566–575.CrossRefGoogle Scholar
Lipatnikov, A.N. & Chomiak, J. 2010 Effects of premixed flames on turbulence and turbulent scalar transport. Prog. Energy Combust. Sci. 36, 1–102.CrossRefGoogle Scholar
Lumley, J.L. & Newman, G.R. 1977 The return to isotropy of homogeneous turbulence. J. Fluid Mech. 82, 161–178.CrossRefGoogle Scholar
Marchioli, C. & Soldati, A. 2002 Mechanisms for particle transfer and segregation in a turbulent boundary layer. J. Fluid Mech. 468, 283–315.CrossRefGoogle Scholar
McLaughlin, J.B. 1989 Aerosol particle deposition in numerically simulated channel flow. Phys. Fluids A 1, 1211–1224.CrossRefGoogle Scholar
Mei, R. 1992 An approximate expression for the shear lift force on a spherical particle at finite Reynolds number. Intl J. Multiphase Flow 18, 145–147.CrossRefGoogle Scholar
Miller, R.S. & Bellan, J. 1999 Direct numerical simulation of a confined three-dimensional gas mixing layer with one evaporating hydrocarbon-droplet-laden stream. J. Fluid Mech. 384, 293–338.CrossRefGoogle Scholar
Moser, R.D., Kim, J. & Mansour, N.N. 1999 Direct numerical simulation of turbulent channel flow up to $Re_{\tau }= 590$. Phys. Fluids 11, 943–945.CrossRefGoogle Scholar
Nakhaei, M. & Lessani, B. 2017 Effects of solid inertial particles on the velocity and temperature statistics of wall bounded turbulent flow. Intl J. Heat Mass Transfer 106, 1014–1024.CrossRefGoogle Scholar
Narayanan, C., Lakehal, D., Botto, L. & Soldati, A. 2003 Mechanisms of particle deposition in a fully developed turbulent open channel flow. Phys. Fluids 15, 763–775.CrossRefGoogle Scholar
Nasr, H., Ahmadi, G. & Mclaughlin, J.B. 2009 A DNS study of effects of particle–particle collisions and two-way coupling on particle deposition and phasic fluctuations. J. Fluid Mech. 640, 507–536.CrossRefGoogle Scholar
Niño, Y. & Garcia, M.H. 1996 Experiments on particle—turbulence interactions in the near–wall region of an open channel flow: implications for sediment transport. J. Fluid Mech. 326, 285–319.CrossRefGoogle Scholar
Nilsen, C., Andersson, H.I. & Zhao, L. 2013 A Voronoï analysis of preferential concentration in a vertical channel flow. Phys. Fluids 25, 115108.CrossRefGoogle Scholar
Pan, Y. & Banerjee, S. 1996 Numerical simulation of particle interactions with wall turbulence. Phys. Fluids 8, 2733–2755.CrossRefGoogle Scholar
Pedinotti, S., Mariotti, G. & Banerjee, S. 1992 Direct numerical simulation of particle behaviour in the wall region of turbulent flows in horizontal channels. Intl J. Multiphase Flow 18, 927–941.CrossRefGoogle Scholar
Picano, F., Battista, F., Troiani, G. & Casciola, C.M. 2011 Dynamics of PIV seeding particles in turbulent premixed flames. Exp. Fluids 50, 75–88.CrossRefGoogle Scholar
Picano, F., Sardina, G. & Casciola, C.M. 2009 Spatial development of particle-laden turbulent pipe flow. Phys. Fluids 21, 93305.CrossRefGoogle Scholar
Picciotto, M., Marchioli, C. & Soldati, A. 2005 Characterization of near-wall accumulation regions for inertial particles in turbulent boundary layers. Phys. Fluids 17, 98101.CrossRefGoogle Scholar
Poinsot, T.J., Haworth, D.C. & Bruneaux, G. 1993 Direct simulation and modeling of flame–wall interaction for premixed turbulent combustion. Combust. Flame 95, 118–132.CrossRefGoogle Scholar
Portela, L.M. & Oliemans, R.V.A. 2003 Eulerian–Lagrangian DNS/LES of particle–turbulence interactions in wall-bounded flows. Intl J. Numer. Meth. Fluids 43, 1045–1065.CrossRefGoogle Scholar
Rashidi, M., Hetsroni, G. & Banerjee, S. 1990 Particle-turbulence interaction in a boundary layer. Intl J. Multiphase Flow 16, 935–949.CrossRefGoogle Scholar
Reeks, M.W. 1983 The transport of discrete particles in inhomogeneous turbulence. J. Aerosol Sci. 14, 729–739.CrossRefGoogle Scholar
Richter, D.H. 2015 Turbulence modification by inertial particles and its influence on the spectral energy budget in planar Couette flow. Phys. Fluids 27, 063304.CrossRefGoogle Scholar
Rogers, C.B. & Eaton, J.K. 1991 The effect of small particles on fluid turbulence in a flat-plate, turbulent boundary layer in air. Phys. Fluids A 3, 928–937.CrossRefGoogle Scholar
Rouson, D.W.I. & Eaton, J.K. 2001 On the preferential concentration of solid particles in turbulent channel flow. J. Fluid Mech. 428, 149–169.CrossRefGoogle Scholar
Saffman, P.G. 1965 The lift on a small sphere in a slow shear flow. J. Fluid Mech. 22, 385–400.CrossRefGoogle Scholar
Saffman, P.G. 1968 The lift on a small sphere in a slow shear flow – corrigendum. J. Fluid Mech. 31, 624–624.Google Scholar
Sankaran, R., Hawkes, E.R., Chen, J.H., Lu, T. & Law, C.K. 2007 Structure of a spatially developing turbulent lean methane–air Bunsen flame. Proc. Combust. Inst. 31, 1291–1298.CrossRefGoogle Scholar
Sardina, G., Schlatter, P., Brandt, L., Picano, F. & Casciola, C.M. 2012 a Wall accumulation and spatial localization in particle-laden wall flows. J. Fluid Mech. 699, 50–78.CrossRefGoogle Scholar
Sardina, G., Schlatter, P., Picano, F., Casciola, C.M., Brandt, L. & Henningson, D.S. 2012 b Self-similar transport of inertial particles in a turbulent boundary layer. J. Fluid Mech. 706, 584–596.CrossRefGoogle Scholar
Schiller, L. & Naumann, Z.A. 1935 Drag coefficient correlation. Z. Verein. Deutsch. Ing. 77, 318–320.Google Scholar
Schlatter, P. & Örlü, R. 2010 Assessment of direct numerical simulation data of turbulent boundary layers. J. Fluid Mech. 659, 116–126.CrossRefGoogle Scholar
Squires, K.D. & Eaton, J.K. 1991 Preferential concentration of particles by turbulence. Phys. Fluids 3, 1169–1178.CrossRefGoogle Scholar
Uijttewaal, W.S.J. & Oliemans, R.V.A. 1996 Particle dispersion and deposition in direct numerical and large eddy simulations of vertical pipe flows. Phys. Fluids 8, 2590–2604.CrossRefGoogle Scholar
Wang, H., Chen, G., Luo, K., Hawkes, E.R., Chen, J.H. & Fan, J. 2021 a Turbulence/flame/wall interactions in non-premixed inclined slot-jet flames impinging at a wall using direct numerical simulation. Proc. Combust. Inst. 38, 2711–2720.CrossRefGoogle Scholar
Wang, H., Luo, K., Hawkes, E.R., Chen, J.H. & Fan, J. 2021 b Turbulence, evaporation and combustion interactions in n-heptane droplets under high pressure conditions using DNS. Combust. Flame 225, 417–427.CrossRefGoogle Scholar
Wang, G. & Richter, D.H. 2019 Two mechanisms of modulation of very-large-scale motions by inertial particles in open channel flow. J. Fluid Mech. 868, 538–559.CrossRefGoogle Scholar
Wang, Y. & Trouvé, A. 2006 Direct numerical simulation of nonpremixed flame–wall interactions. Combust. Flame 144, 461–475.CrossRefGoogle Scholar
Wang, Z., Wang, H., Luo, K. & Fan, J. 2020 Direct numerical simulation of particle-laden turbulent boundary layers without and with combustion. Phys. Fluids 32, 105108.CrossRefGoogle Scholar
Wang, H., Wang, Z., Luo, K., Hawkes, E.R., Chen, J.H. & Fan, J. 2021 c Direct numerical simulation of turbulent boundary layer premixed combustion under auto-ignitive conditions. Combust. Flame 228, 292–301.CrossRefGoogle Scholar
Willmarth, W.W. & Lu, S.S. 1972 Structure of the Reynolds stress near the wall. J. Fluid Mech. 55, 65–92.CrossRefGoogle Scholar
Xiao, W., Jin, T., Luo, K., Dai, Q. & Fan, J. 2020 Eulerian–Lagrangian direct numerical simulation of preferential accumulation of inertial particles in a compressible turbulent boundary layer. J. Fluid Mech. 903, A19.CrossRefGoogle Scholar
Young, J. & Leeming, A. 1997 A theory of particle deposition in turbulent pipe flow. J. Fluid Mech. 340, 129–159.CrossRefGoogle Scholar
Zhao, L., Andersson, H.I. & Gillissen, J.J.J. 2010 Turbulence modulation and drag reduction by spherical particles. Phys. Fluids 22, 81702.CrossRefGoogle Scholar
Zhao, L., Andersson, H.I. & Gillissen, J.J.J. 2013 Interphasial energy transfer and particle dissipation in particle-laden wall turbulence. J. Fluid Mech. 715, 32–59.CrossRefGoogle Scholar
Zhao, P., Wang, L. & Chakraborty, N. 2018 Analysis of the flame–wall interaction in premixed turbulent combustion. J. Fluid Mech. 848, 193–218.CrossRefGoogle Scholar
Zhou, T., Zhao, L., Huang, W. & Xu, C. 2020 Non-monotonic effect of mass loading on turbulence modulations in particle-laden channel flow. Phys. Fluids 32, 43304.Google Scholar
Zonta, F., Marchioli, C. & Soldati, A. 2008 Direct numerical simulation of turbulent heat transfer modulation in micro-dispersed channel flow. Acta Mech. 195, 305–326.CrossRefGoogle Scholar