2.1. Numerical methodology

The FSI is simulated using the immersed boundary (IB) method that was first introduced by Peskin (Reference Peskin1972) to simulate the blood flow around the flexible leaflet of a human heart. In the framework of the IB method, the flow governing equations are discretized on a fixed Cartesian grid, which generally does not conform to the geometry of moving solids. As a result, the boundary conditions on the fluid–cylinder interface, manifesting the interaction between the fluid and the structure, cannot be imposed directly. Instead, an extra body force is added to the momentum equation using interpolation and distribution functions to take such interaction into account. Compared with conventional numerical methods, the IB method has significant advantages, particularly in FSI simulations with topological changes. Another merit of the IB method lies in its parameterized and fast implementation for a large number of simulations with different geometric configurations compared with conventional methods using body-conformal grids.

The dynamics of the elastically supported D-section prism is simplified as a mass-damper-spring system. In this study the prism is free to oscillate in both the streamwise and transverse directions and the governing equations of prism motion are

(2.1)\begin{gather} \ddot{X}+4 {\rm \pi}F_n \zeta \dot{X}+(2 {\rm \pi}F_n)^2 X=\frac{4 C_D}{{\rm \pi} m^*}, \end{gather}

(2.2)\begin{gather} \ddot{Y}+4 {\rm \pi}F_n \zeta \dot{Y}+(2 {\rm \pi}F_n)^2 Y=\frac{4 C_L}{{\rm \pi} m^*}, \end{gather}

where $m^*$ ($= 8m/{\rm \pi} \rho D^2$, $m$ is the prism mass per unit length, $\rho$ is the fluid density and $D$ is the prism diameter) is the mass ratio, $\zeta$ is the structural damping ratio, $F_n$ (= $f_nD$/$U_\infty$, $f_n$ is the natural frequency of the prism and $U_\infty$ is the incoming flow velocity) is the normalized natural frequency of the prism, $X$ (= $x/D$, $x$ is the streamwise displacement) and $Y$ (= $y/D$, $y$ is the transverse displacement) are the normalized streamwise and transverse displacements, and $C_D$ ($= 2F_D/\rho U_\infty ^2 D$, $F_D$ is the drag force) and $C_L$ ($= 2F_L/\rho U_\infty ^2 D$, $F_L$ is the lift force) are the dimensionless drag and lift coefficients, respectively. The governing equations of prism motion are based on Newton's second law and solved by applying the standard Newmark-$\beta$ method – a method of numerical integration used to solve differential equations, which is widely used in numerical evaluations of structural responses. More details of the present methodology can be found in our previous works (Ji, Munjiza & Williams Reference Ji, Munjiza and Williams2012; Chen et al. Reference Chen, Ji, Xu, Liu and Campbell2015, Reference Chen, Ji, Xu and Williams2019, Reference Chen, Ji, Alam, Xu, An and Tong2022b).

The streamwise and transverse lengths of the computational domain are 100$D$, as shown in figure 1(a). The prism is placed at the centre of the computational domain. The domain is discretized by a non-uniform Cartesian grid with the largest resolution of $2304\times 1536$. To improve the accuracy of numerical results, a rectangular region of $30D\times 20D$, enclosing the prism, is meshed uniformly, with a non-dimensional grid spacing of 1/64 in both directions. A stretched mesh is adopted out of the region to keep the total grid number within an affordable range. The same mesh configuration was adopted in our previous simulations (Chen et al. Reference Chen, Ji, Xu, Liu and Campbell2015; Chen et al. 2018; Chen et al. Reference Chen, Ji, Alam, Williams and Xu2020b, Reference Chen, Ji, Alam, Xu, An and Tong2022b). A Dirichlet-type boundary and a Neumann-type boundary are adopted at the inflow and outflow, respectively. The top and bottom boundaries are set as free-slip boundaries. The number of IB points for the prism is selected as 506 to ensure at least one IB point in each grid cell.

Figure 1. (a) Computation domain for the 2DOF FIV of a D-section prism and (b) a sketch for the angle of attack ($\alpha$).

The angle of attack ($\alpha$) is varied from $0^{\circ }$ to $180^{\circ }$, where $\alpha = 0^{\circ }$ and $180^{\circ }$ correspond to the configurations with the flat surface pointing downstream and upstream, respectively (figure 1b). The reduced velocity $U^*$ is varied from 2.0 to 20.0, with $m^* = 2.0$ and $\zeta = 0$. To keep the effective Reynolds number constant for $\alpha = 0^{\circ }\unicode{x2013}180^{\circ }$, we define the Reynolds number as ${Re} = U_\infty D_e/\nu = 100$, where effective diameter $D_e=0.5(1+|\cos \alpha |) D$ and $\nu$ is the fluid kinematic viscosity. The intervals of $\alpha$ and $U^*$ are as small as $\Delta \alpha =5^{\circ }$ and $\Delta U^{*}=0.1$, which lead to more than 1000 simulation cases.

The $\bar {C}_{D}$ or $\bar {C}_{L}$ and $C_{D}^{\prime }$ or $C_{L}^{\prime }$ are the time-mean and root-mean-square (r.m.s.) values of the corresponding forces, respectively. The non-dimensional streamwise and transverse amplitudes are defined as $A_x^{*}=\sqrt {2} y_{rms} / D$ and $A_y^{*} =\sqrt {2} y_{rms} / D$, where $x_{rms}$ and $y_{rms}$ are the r.m.s. values of the streamwise and transverse displacements, respectively. The lift, drag and vibration frequencies are obtained through the fast Fourier transform, and the phase lag between the lift and displacement is obtained through the Hilbert transform.

2.2. Convergence analysis and validation cases

Both the convergence analysis and validation cases for the present numerical methodology have been presented in Chen et al. (Reference Chen, Ji, Alam, Xu, An and Tong2022b) for the FIV of a D-section prism. For the sake of conciseness, in this paper we check only the non-dimensional time step ($\Delta t U_{\infty }/ D$) for the 2DOF FIV of a D-section prism. As shown in table 1, the variations in the fluid forces, vibration amplitudes and frequencies are insignificant when the non-dimensional time step $\Delta t U_{\infty }/ D$ is reduced from 0.004 to 0.002. It suggests that $\Delta t U_{\infty }/ D$ = 0.004 is enough for the present simulations.

Table 1. Comparison of the results of 2DOF FIV of a D-section prism at different non-dimensional time steps. Here, $F_{x}$ ($= f_{x} D/U_{\infty }$) and $F_{y}$ ($= f_{y} D/U_{\infty }$) are the normalized vibration frequencies in the streamwise and transverse directions, respectively.

3.1. Group 1: VIV

In the typical VIV regime, both $A_x^{*}$ and $A_y^{*}$ exhibit a similar behaviour to the VIV of a circular cylinder (Singh & Mittal Reference Singh and Mittal2005; Leontini, Thompson & Hourigan Reference Leontini, Thompson and Hourigan2006b; Prasanth & Mittal Reference Prasanth and Mittal2008). They first increase and then decrease mildly with increasing $U^*$ (figure 3a). Similar to that in the 2DOF VIV of a circular cylinder, $A_x^{*}$ is much smaller than $A_y^{*}$ (Jauvtis & Williamson Reference Jauvtis and Williamson2004; Bourguet Reference Bourguet2020). The maximum $A_y^{*}$ is obtained at $U^*$ = 5.0, regardless of $\alpha$. Compared with the 2DOF VIV of a circular cylinder, $A_y^{*}$ of the D-section prism is comparable for $U^* \le 7.5$ but significantly higher for $U^*> 7.5$. On the contrary, $A_x^{*}$ for $\alpha = 15^{\circ }$ and $30^{\circ }$ is relatively higher for $U^*< 8.5$, but comparable to that of a circular cylinder for $U^* \ge 8.5$. This suggests that the symmetry breaking ($\alpha \neq 0^{\circ }$) of the D-section prism exerts distinct impacts, i.e. significantly promoting $A_x^{*}$ in the large $A_y^{*}$ region and enlarging $A_y^{*}$ for $U^*> 7.5$. The hysteretic VIV regime is characterized by the appearance of a hysteresis loop as $U^*$ increases and decreases. As shown in figure 3(c), $A_x^{*}$ and $A_y^{*}$ rapidly increase up to $U^* = 5.0\unicode{x2013}6.0$ and then slowly decrease before plunging to smaller values at $U^* = 8.0\unicode{x2013}10.0$. After the plunge, $A_x^{*}$ becomes very small and $A_y^{*}$ keeps declining slowly, being smaller at a higher $\alpha$. Before the plunge, $A_x^{*}$ and $A_y^{*}$ are higher at larger $\alpha$ but independent of $\alpha$ for $U^*\le 4.5$. In the extended VIV regime the prism vibration starts at the smallest $U^*$ examined, and the amplitudes become small after a critical $U^*$, i.e. $U^* = 13.0$ at $\alpha = 50^{\circ }$ and $U^* = 13.5$ at $\alpha = 55^{\circ }$ (figure 3e). Compared with the typical VIV, the large-amplitude vibration appears in a wider range of $U^*$. In the narrowed VIV regime, $A_x^{*}$ and $A_y^{*}$ first increase and then decrease slowly with increasing $U^*$, with significant growth as $\alpha$ increases (figure 3g). The large-amplitude vibration exists in a narrow range of $U^*$.

Figure 3. Dependence of non-dimensional vibration amplitudes ($A_x^{*}$ and $A_y^{*}$) and frequencies ($f_x^{*}$ and $f_y^{*}$) on reduced velocity $U^*$ and angle of attack $\alpha$. (a,b) Typical VIV, (c,d) hysteretic VIV, (e, f) extended VIV and (g,h) narrowed VIV. The results of the 2DOF VIV of a circular cylinder at ${Re} = 100$ are superimposed in (a,b). The open circles denote the results of the 1DOF case. The inclined dashed lines in (b,d, f,h) with the same colour as that of the streamwise amplitude represent the vortex shedding frequency ($St$) of the corresponding stationary D-section prism. Same for figures 7 and 11.

The spectral results provide further insights into the characteristics of these VIV regimes. In the typical VIV, three different branches are recognized based on the features of $f_x^{*}$ and $f_y^{*}$ (Khalak & Williamson Reference Khalak and Williamson1996), as shown in figure 3(b). At $2.0 \le U^*< 3.5$, $A_x^{*}$ and $A_y^{*}$ are marginal, and $f_y^{*}$ closely follows the vortex shedding frequency ($St$) of the stationary D-section prism, indicating a desynchronization region. At $3.5 \le U^* \le 5.0$, $A_y^{*}$ increases sharply and $f_y^{*}$ remains at 0.8, signifying the initial branch. This constant $f_y^{*}$ below the $St$ line in the initial branch, known as soft lock-in, has been reported in Mittal & Kumar (Reference Mittal and Kumar1999), Singh & Mittal (Reference Singh and Mittal2005) and Prasanth et al. (Reference Prasanth, Behara, Singh, Kumar and Mittal2006). After $U^*> 5.0$, $A_x^{*}$ and $A_y^{*}$ decrease gradually, and the lower branch appears. However, unlike the circular cylinder counterpart, $f_y^{*}$ for the D-section prism linearly increases with increasing $U^*$ due to the fixed shear layer separation at the prism corners (Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). Owing to the asymmetric vortex shedding (especially at larger $\alpha$), $f_x^{*}/f_y^{*}$ changes from 2 to 1 (Chen et al. Reference Chen, Ji, Alam, Xu and Zhang2022a,Reference Chen, Ji, Xu and Zhangc). In the hysteretic VIV regime the response exhibits four branches (figure 3d). At $2.0 \le U^*< 3.0$, $A_x^{*}$ and $A_y^{*}$ are relatively small, and $f_x^{*}$ and $f_y^{*}$ closely follow the $St$ line. It is, therefore, a desynchronization region. However, at $3.0 \le U^* \le 3.5$, $A_x^{*}$ and $A_y^{*}$ rapidly increase, and $f_x^{*}$ and $f_y^{*}$ remain constant around 0.6, deviating from the $St$ line. This corresponds to the initial branch. In the third region, i.e. $3.5 < U^* \le U_{p}^*$, the amplitudes are large, and $f_x^{*}$ and $f_y^{*}$ increase slowly with increasing $U^*$, deviating from the $St$ line. Here, $U_{p}^*$ is defined as the reduced velocity at which the amplitude plunges, which strongly depends on $\alpha$. Similar to that in the VIV of a circular cylinder (Prasanth & Mittal Reference Prasanth and Mittal2008; Zhang et al. Reference Zhang, Li, Ye and Jiang2015; Navrose & Mittal Reference Navrose and Mittal2016), lock-in occurs and the lower branch takes place. After $U^*> U_{p}^*$, $f_x^{*}$ and $f_y^{*}$ increase linearly, approximately following the $St$ line, which leads to the desynchronization region. Similarly, in the extended VIV regime, three different branches are recognized based on the characteristics of $f_x^{*}$ and $f_y^{*}$ (figure 3f). At small $U^*$ ($2.0 \le U^*< 3.5$), $A_x^{*}$ and $A_y^{*}$ rapidly increase, and $f_x^{*}$ and $f_y^{*}$ closely follow the $St$ line, indicating a desynchronization region. At $3.5 \le U^*< 13.5$ for $\alpha = 50^{\circ }$ and $3.5 \le U^*< 13.0$ for $\alpha = 55^{\circ }$, $A_x^{*}$ and $A_y^{*}$ are relatively large, and $f_x^{*}$ and $f_y^{*}$ increase slowly from a value smaller than 1.0 to higher than 1.0, deviating significantly from the $St$ line. It is the lock-in region. At $U^*> 13.0\unicode{x2013}13.5$, $A_x^{*}$ and $A_y^{*}$ are small and invariant, while $f_x^{*}$ and $f_y^{*}$ increase linearly, being slightly smaller than $St$. It is thus a desynchronization region. In the narrowed VIV regime, $f_x^{*}$ and $f_y^{*}$ are identical (figure 3h). With increasing $U^*$, $f_x^{*}$ and $f_y^{*}$ linearly increase, approximately following the $St$ line, which is related to the fixed shear layer separation point on the upper side of the prism.

The power spectral density (PSD) functions of the displacements in these VIV regimes are shown in figure 4. In the typical and hysteretic VIV, only one frequency is observed in the transverse vibration, while both the fundamental and harmonic frequencies emerge in the streamwise vibration, with the former having a comparable amplitude to that of the latter, especially at higher $U^*$ (figure 4a – d). As shown in figure 5(a), at $\alpha = 0^{\circ }$, the symmetric vortex shedding from the prism is maintained and a figure-‘8’ trajectory dominates the entire simulated $U^*$ range. However, at $\alpha = 15^{\circ }\unicode{x2013}30^{\circ }$, the displacement trajectories change from figure-‘8’ to figure-‘o’ as $U^*$ increases, due to the augmentation of the second harmonic component. In the hysteretic VIV, although $f_x^{*}$ and $f_y^{*}$ are identical, the trajectories of the displacements change from figure-‘o’ ($U^* = 2.0\unicode{x2013}5.5$) to figure-‘8’ ($U^* = 6.0\unicode{x2013}7.0$), then to figure-‘o’ ($U^*$ = 7.5–8.0) and figure-‘8’ ($U^*>$ 8.0) as a result of the variation in the amplitude at the second harmonic frequency (figure 5b). In the extended VIV regime the second harmonic frequency is apparent only in the lock-in region with large amplitudes (figure 4e, f). Additionally, the third harmonic frequency in the streamwise vibration becomes visible in the range of $6.5 < U^*< 13.5$. As shown in figure 5(c), the trajectories of the displacements are figure-‘o’ shaped for most $U^*$ cases, which is consistent with the identical $f_x^{*}$ and $f_y^{*}$. However, due to the augmented component of the second harmonic frequency, the figure-‘8’ shaped trajectory is observed in the range of $U^* = 6.0\unicode{x2013}7.5$. In the narrowed VIV regime the dominant frequencies linearly increase with increasing $U^*$ (figure 4g,h). The second harmonic frequency is noticeable only in the streamwise displacement as a result of the shear layer reattachment on the flat part of the prism. As shown in figure 5(d), except at $\alpha = 105^{\circ }$, the trajectories are mostly figure-‘o’ shaped. Because of the intensified amplitude of the second harmonic frequency, the figure-‘8’ trajectory is dominant at $\alpha = 105^{\circ }$ and several small $U^*$ cases at $\alpha = 120^{\circ }\unicode{x2013}135^{\circ }$.

Figure 4. Power spectral density (PSD) of the displacements in the streamwise and transverse directions at $U^* = 2\unicode{x2013}20$ and selected $\alpha$ cases. For each $U^*$, the PSD is normalized by its maxima. (a,b) Typical VIV at $\alpha = 15^{\circ }$, (c,d) hysteretic VIV at $\alpha = 40^{\circ }$, (e, f) extended VIV at $\alpha = 50^{\circ }$ and (g,h) narrowed VIV at $\alpha = 105^{\circ }$. In each plot the inclined dashed line represents the $St$ of the stationary D-section prism. Same for figures 8 and 12.

Figure 5. The trajectories of the streamwise and transverse displacements versus reduced velocity $U^*$ and angle of attack $\alpha$. (a) Typical VIV, (b) hysteretic VIV, (c) extended VIV and (d) narrowed VIV. The displacements are of a figure-‘8’ (blue), irregular (grey), quasi-periodic (olive) or raindrop (red) shaped trajectory. The lilac region represents the prism moving downstream at the extremes of the transverse oscillation (CW) while the light green region represents the prism moving upstream at the extremes of the transverse oscillation (CCW). Same for figures 9 and 13.

As shown in figure 6(b–d), the prism vibrations in the typical VIV are usually periodic. However, an exception is observed at $U^* = 3.5$ where the prism vibration is quasi-periodic, involving a beat-like phenomenon (figure 6a). At this $U^*$, two incommensurate frequencies are identified in the displacements, one corresponding to the vibration frequency while the other corresponding to the natural frequency of the prism, and they have comparable amplitudes (Navrose et al. Reference Navrose, Yogeswaran, Sen and Mittal2014; Kumar, Singh & Sen Reference Kumar, Singh and Sen2018; Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). Cheng et al. (Reference Cheng, Lien, Yee and Zhang2022) observed that with this trait, the two frequencies compete with each other in a balanced way. In other three VIV regimes, the prism vibrations are periodic (figure 6e–l). Within the hysteresis loop, the vibrations in the increasing and decreasing cases are significantly different (figure 6e, f).

Figure 6. Time histories of the streamwise and transverse displacements at different $U^*$ and $\alpha$ values. (a–d) Typical VIV, (e–g) hysteretic VIV, (h–j) extended VIV and (k,l) narrowed VIV.

3.2. Group 2: galloping or combined galloping

At $\alpha = 70^{\circ }$, both $A_x^{*}$ and $A_y^{*}$ increase synchronously with increasing $U^*$, and there is no sign of amplitude convergence (figure 7a). As shown later, both $f_x^{*}$ and $f_y^{*}$ show galloping characters, i.e. constant values. Accordingly, the vibrations in both directions display a galloping behaviour, which is referred to as dual galloping. The ‘dual’ is derived from Dahl et al. (Reference Dahl, Hover, Triantafyllou, Dong and Karniadakis2007, Reference Dahl, Hover, Triantafyllou and Oakley2010) who first introduced the concept of ‘dual resonance’ in the 2DOF VIV of a circular cylinder, where the circular cylinder is resonant simultaneously in both streamwise and transverse directions. However, unlike Dahl et al. (Reference Dahl, Hover, Triantafyllou, Dong and Karniadakis2007) where the ratio ($f_{xn}/f_{yn}$) of the natural frequencies in the streamwise and transverse directions is $2:1$, it is $1:1$ in the present study. This is the first observation of dual galloping in the 2DOF FIV of a non-circular prism. In the response there are several kinks, e.g. at $U^* = 18.0$, resulting from the lock-in of higher harmonic frequencies (Bearman et al. Reference Bearman, Gartshore, Maull and Parkinson1987; Nemes et al. Reference Nemes, Zhao, Lo Jacono and Sheridan2012; Zhao et al. Reference Zhao, Leontini, Lo Jacono and Sheridan2014; Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). At $\alpha = 165^{\circ }\unicode{x2013}180^{\circ }$, $A_y^{*}$ generally increases with increasing $U^*$, indicating the transverse galloping (figure 7c). However, as $U^*$ increases, $A_x^{*}$ initially augments before being constant, no galloping in the streamwise direction. Thus, this response is named transverse-only galloping. The $A_x^{*}$ at $\alpha = 165^{\circ }\unicode{x2013}170^{\circ }$ is slightly larger than that at $\alpha = 175^{\circ }\unicode{x2013}180^{\circ }$, while $A_y^{*}$ is smaller at $\alpha = 165^{\circ }\unicode{x2013}170^{\circ }$ than at $\alpha = 175^{\circ }\unicode{x2013}180^{\circ }$ for $U^*>$ 7.0. Hysteresis prevails and its corresponding $U^*$ range varies significantly with $\alpha$. The hysteresis loop exists at $17.2 < U^*< 19.2$ and $18.7 < U^*< 19.5$ for $\alpha = 165^{\circ }$ and $170^{\circ }$, respectively, while at $13.2 < U^*< 14.5$ and $12.7 < U^*< 15.0$ for $\alpha = 175^{\circ }$ and $180^{\circ }$, respectively. As shown in figure 7(e), at $\alpha = 75^{\circ }\unicode{x2013}80^{\circ }$, the response can be divided into two regions: VIV and galloping (Sen & Mittal Reference Sen and Mittal2011; Cui et al. Reference Cui, Zhao, Teng and Cheng2015; Bhatt & Alam Reference Bhatt and Alam2018). In the VIV region, $A_x^{*}$ and $A_y^{*}$ are very small, while in the galloping region they monotonically increase for $\alpha = 80^{\circ }$ but non-monotonically for $\alpha = 75^{\circ }$ where $A_x^{*}$ and $A_y^{*}$ locally peak at the same $U^*$.

Figure 7. Dependence of non-dimensional vibration amplitudes ($A_x^{*}$ and $A_y^{*}$) and frequencies ($f_x^{*}$ and $f_y^{*}$) on reduced velocity $U^*$ and angle of attack $\alpha$. (a,b) Dual galloping, (c,d) transverse-only galloping and (e, f) combined VIV and galloping.

In the dual galloping regime, $f_x^{*}$ and $f_y^{*}$ are identical (figure 7b). At small $U^*$, i.e. $2.0 \le U^*< 3.0$, $f_x^{*}$ and $f_y^{*}$ closely follow the $St$ line, indicating desynchronized vibration. For $U^* \ge 3.0$, $f_x^{*}$ and $f_y^{*}$ are constant at $\approx$0.75, which is a characteristic feature of galloping (Nemes et al. Reference Nemes, Zhao, Lo Jacono and Sheridan2012; Zhao et al. Reference Zhao, Leontini, Lo Jacono and Sheridan2014; Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). In the transverse-only galloping regime, $f_y^{*}$ initially linearly increases, closely following the $St$ line, before reaching a constant value of approximately 1.0, regardless of $\alpha$ (figure 7d). In the hysteresis region there is a small discrepancy in $f_y^{*}$ between the increasing and decreasing cases due to the modification of vortex dynamics. At $\alpha = 165^{\circ }\unicode{x2013}170^{\circ }$, except at $U^* = 8.0$ and $\alpha = 165^{\circ }$, $f_x^{*}$ is identical to $f_y^{*}$. However, owing to the symmetry recovery of the cross-section to the incoming flow, $f_x^{*}$ is twice $f_y^{*}$ for some $U^*$ cases at $\alpha = 175^{\circ }$ while for all examined $U^*$ at $\alpha = 180^{\circ }$. In the combined VIV and galloping regime, $f_x^{*}$ and $f_y^{*}$ closely follow the $St$ line in the VIV region, thus, no lock-in, while in the galloping they are constant at around 0.65 for $\alpha = 75^{\circ }$ and 0.5 for $\alpha = 80^{\circ }$ (figure 7f).

The PSD results of the displacements shown in figure 8 provide additional information. In the dual galloping regime, in addition to the fundamental frequency, the second and third harmonic frequencies become noticeable as $U^*$ increases (figure 8a,b). The appearance of higher harmonic frequencies is related to the increased nonlinearity of the force at higher amplitude and relative motion of shed vortices with respect to the body motion. The trajectories in the dual galloping regime are mostly figure-‘o’ shaped, indicating relatively lower amplitudes of higher harmonic frequencies compared with the fundamental frequency (figure 9a). At $U^* = 19.0$ and 20.0, multiple frequencies appear in both streamwise and transverse displacements, resulting in chaotic trajectories. Similarly, in the transverse-only galloping regime, higher harmonic frequencies appear, especially in the streamwise displacement, as $U^*$ increases (figure 8c,d). Incommensurate frequencies identified at $U^* = 6.0$ and 13.0 lead to chaotic responses. As the symmetry of the cross-section with respect to the incoming flow recovers, the trajectories are of figure-‘8’ for most $U^*$ cases, while figure-‘o’ shaped trajectory only exists at $U^* = 4.0\unicode{x2013}4.5$ (figure 9b). However, at $\alpha = 165^{\circ }$, although the cross-section remains asymmetric, figure-‘8’ shaped trajectory becomes dominant, particularly at large $U^*$. In the combined VIV and galloping regime, the PSD features differ between the VIV and galloping regions (figure 8e, f). In the VIV region the dominant frequency has a much higher amplitude, while the other frequencies are trivial. In the galloping region the fundamental frequency is accompanied by the second and third harmonic frequencies in both directions. In the combined regime the trajectories of the displacements indicate figure-‘o’ shaped responses (figure 9a), indicating identical frequencies in both directions. The influences of higher harmonic frequencies are noticed only at $U^* = 4.0$.

Figure 8. The PSD of the displacements in the streamwise and transverse directions at $U^* = 2\unicode{x2013}20$ and selected $\alpha$ cases. (a,b) Dual galloping at $\alpha = 70^{\circ }$, (c,d) transverse-only galloping at $\alpha = 180^{\circ }$ and (e, f) combined VIV and galloping at $\alpha = 80^{\circ }$.

Figure 9. The trajectories of the streamwise and transverse displacements versus reduced velocity $U^*$ and angle of attack $\alpha$. (a) Dual galloping and combined response and (b) transverse-only galloping.

The displacements in the dual galloping regime deviate from a sinusoidal shape due to the appearance of harmonic frequencies, although they remain periodic (figure 10a,b). In the transverse-only galloping regime the displacements can be irregular, quasi-periodic or periodic (figure 10c–f). At $U^* = 6.0$, the response shows intermittent behaviour where the vibration alternates between periodic and irregular (figure 10c,d). In the hysteretic region the prism vibrations are relatively regular in both increasing and decreasing cases (figure 10e, f). In the combined regime the prism vibrations are rather regular (figure 10g,h), but the presence of higher harmonic frequencies makes the vibrations in the galloping region deviate from a pure sinusoidal shape.

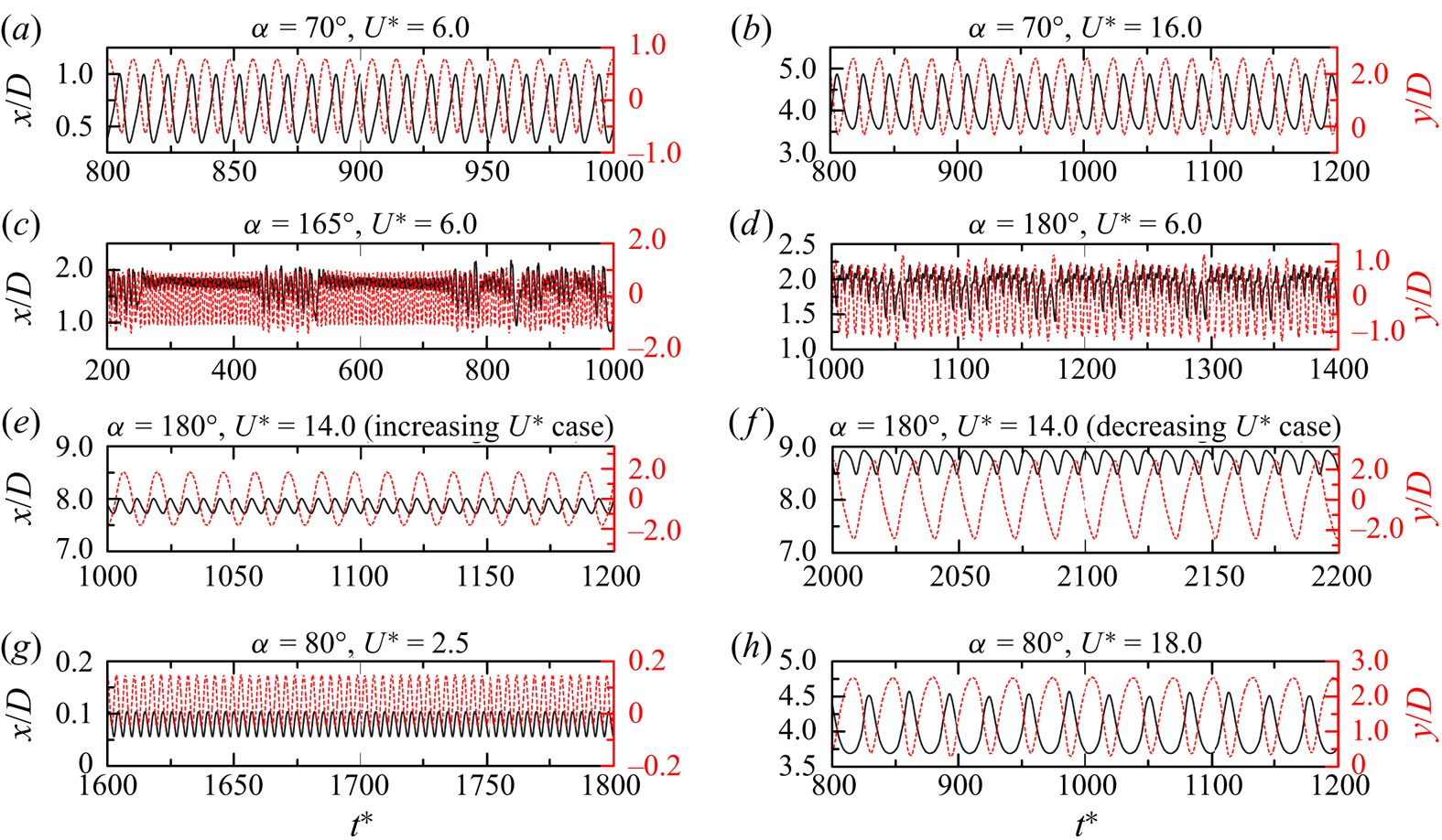
Figure 10. Time histories of the streamwise and transverse displacements at different $U^*$ and $\alpha$ values. (a,b) Dual galloping, (c–f) transverse-only galloping and (g,h) combined VIV and galloping.

3.3. Group 3: galloping-like response

At $\alpha = 60^{\circ }\unicode{x2013}65^{\circ }$, the response exhibits a galloping-like behaviour, but with complicated dependence on $U^*$ (figure 11a). At $\alpha = 60^{\circ }$, the response can be divided into three branches. In the first region ($2.0 \le U^* \le 11.5$ for the increasing case or $2.0 \le U^* \le 11.3$ for the decreasing case), $A_x^{*}$ and $A_y^{*}$ initially increase with increasing $U^*$ and then gradually decrease. However, this behaviour does not persist at higher $U^*$. In the second region ($11.5 < U^*< 16.0$ for the increasing case or $11.3 < U^*< 14.2$ for the decreasing case), $A_x^{*}$ and $A_y^{*}$ jump and decrease gradually with increasing $U^*$. In the third region ($16.0 \le U^* \le 20.0$ for the increasing case or $14.2 \le U^* \le 20.0$ for the decreasing case), $A_x^{*}$ and $A_y^{*}$ are very small. The transitions between adjacent regions are hysteretic, with the hysteresis loop between the second and third regions being wider than the other. At $\alpha = 65^{\circ }$, the variation in amplitudes with increasing $U^*$ is similar to that at $\alpha = 60^{\circ }$, but the first region extends to a higher $U^*$, resulting in only two regions in the examined $U^*$ range. Hysteresis is observed between the two regions. Based on $f_x^{*}$ and $f_y^{*}$, the first region can be divided into desynchronization and lock-in regions (figure 11b). For both $\alpha$ cases, $f_x^{*}$ and $f_y^{*}$ at $U^*<$ 3.5 closely follow the $St$ line, i.e. desynchronization region. In the remaining $U^*$ range of the first region, $f_x^{*}$ and $f_y^{*}$ are approximately constant but lower than 1.0, i.e. lock-in region. The extension of the first region is a result of the widening lock-in as $\alpha$ increases from $60^{\circ }$ to $65^{\circ }$. This response can be considered as a transition from extended VIV to dual galloping. Furthermore, at $\alpha = 60^{\circ }$ and $U^* = 12.5\unicode{x2013}15.5$, the vortex shedding frequency is two or three times the vibration frequency, suggesting the absence of synchronization between the prism vibration and vortex formation. Therefore, this transition response belongs to the galloping-like response (Stansby & Rainey Reference Stansby and Rainey2001; Yogeswaran & Mittal Reference Yogeswaran and Mittal2011). At $\alpha = 150^{\circ }\unicode{x2013}160^{\circ }$, $A_x^{*}$ and $A_y^{*}$ are highly contingent on $U^*$ (figure 11c). Depending on $\alpha$, the response can be divided into three or four regions. At $\alpha = 150^{\circ }$, three regions are identified. In the first region, i.e. $2.0 \le U^* \le 5.4$ (the increasing case) or $2.0 \le U^* \le 4.4$ (the decreasing case), $A_x^{*}$ and $A_y^{*}$ gradually increase. In the second region, i.e. $5.4 < U^* \le 6.6$ (the increasing case) or $4.4< U^* \le 6.6$ (the decreasing case), $A_x^{*}$ and $A_y^{*}$ are almost constant. For $U^*>$ 6.6, the third region is characterized by small amplitudes. The variation of $f_x^{*}$ and $f_y^{*}$ shown in figure 11(d) provides additional information. In the first region, demarcated by $U^* = 3.0$, the vibration can be either desynchronized or lock-in, while that is lock-in in the second region and becomes desynchronized again in the third region. At $\alpha = 160^{\circ }$, the response is also divided into three regions. However, in the third region, i.e. $16.0 < U^* \le 20.0$ (the increasing case) or $15.0 < U^* \le 20.0$ (the decreasing case), $A_x^{*}$ and $A_y^{*}$ are significantly large, and $f_x^{*}$ and $f_y^{*}$ are approximately constant, indicating the lock-in. At $\alpha = 155^{\circ }$, the response is classified into four regions. The features of the former two regions are similar to those at $\alpha = 150^{\circ }$, except for $U^* = 8.3\unicode{x2013}8.6$, where the amplitudes are significantly large. As shown later, the wake mode in this region is the 3S+2S mode while the 2S mode is in the near region. In the third region, i.e. $10.4 < U^*< 14.5$ (the increasing case) or $9.8 < U^*< 14.5$ (the decreasing case), $A_x^{*}$ and $A_y^{*}$ are large again, and $f_x^{*}$ and $f_y^{*}$ are approximately constant at 1.0. Thus, lock-in occurs. Hysteresis loops are observed between some adjacent regions. In the fourth region, i.e. $U^* \ge 14.5$, $A_x^{*}$ and $A_y^{*}$ are much smaller, and $f_x^{*}$ and $f_y^{*}$ follow the $St$ line again. Overall, the response at $\alpha = 150^{\circ }\unicode{x2013}160^{\circ }$ behaves like a transition from narrowed VIV to transverse-only galloping. Similar to the first transition, the vortex shedding frequency is also several times the vibration frequency in the region of $U^* \ge 9.5$ for $\alpha = 160^{\circ }$, and the synchronization between the prism vibration and vortex formation loses. Therefore, this transition also belongs to the galloping-like response.

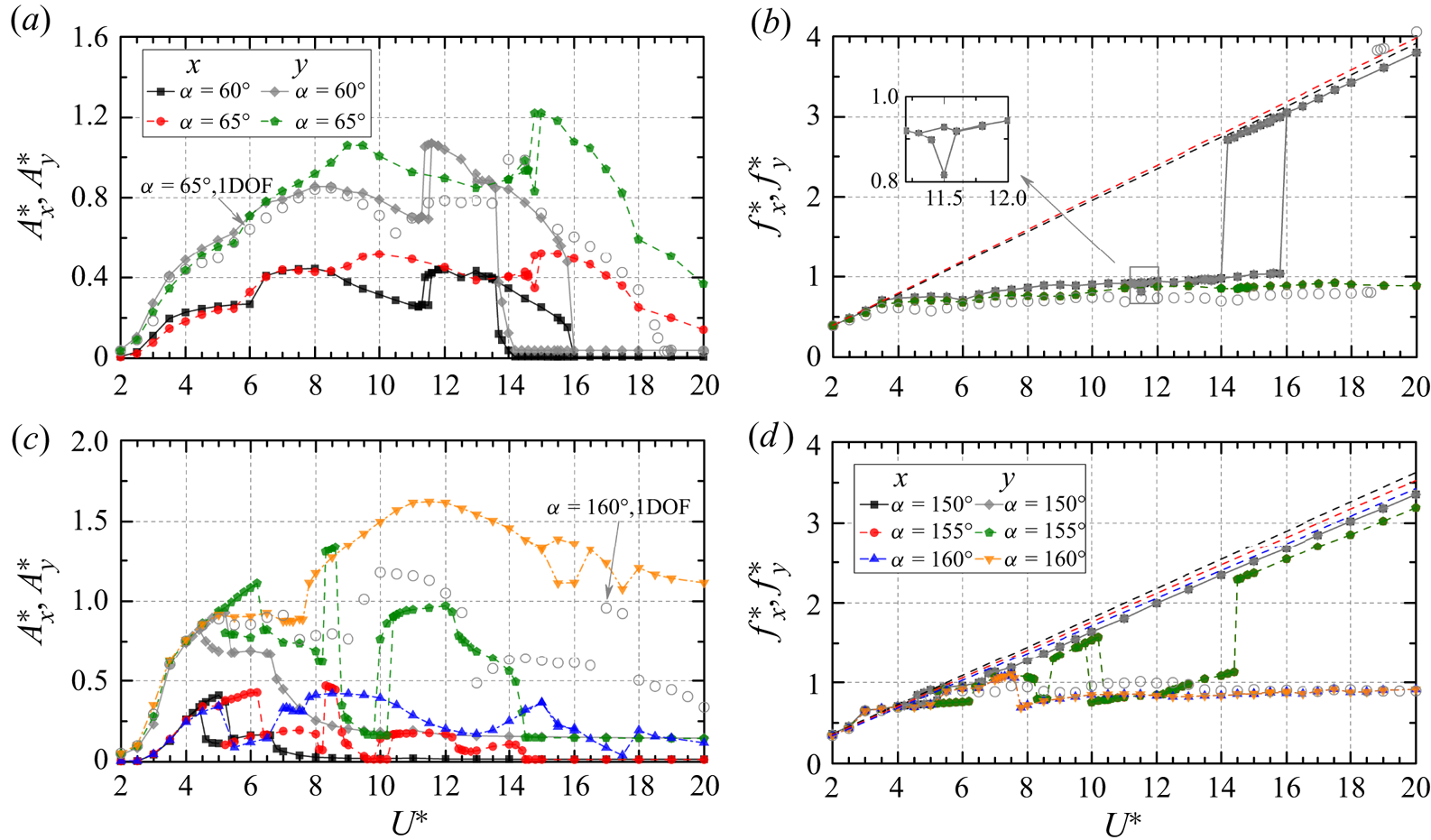
Figure 11. Dependence of non-dimensional vibration amplitudes ($A_x^{*}$ and $A_y^{*}$) and frequencies ($f_x^{*}$ and $f_y^{*}$) on reduced velocity $U^*$ and angle of attack $\alpha$. (a,b) First transition and (c,d) second transition.

The PSD results of the displacements for the galloping-like response are shown in figure 12. In the first transition the fundamental frequency is accompanied by the second harmonic frequency in the lock-in region. At $12.0 < U^*< 15.0$, other frequencies are observed in the displacements in both directions, suggesting complex interactions between the prism vibration and vortex shedding. The trajectories of the displacements are mostly figure-‘o’ shapes (figure 13a), primarily caused by significantly asymmetric vortex shedding. Incommensurate frequencies in the displacements lead to chaotic trajectories at $U^* = 13.0$ and 14.0. At $U^* = 7.5\unicode{x2013}9.0$, the trajectories are figure-‘8’ or figure-‘8’ like, which may be caused by the increasing streamwise amplitude at the second harmonic frequency. In the second transition several frequencies are observed in the displacements, including the dominant frequency and its higher harmonics (figure 12c,d). However, for some $U^*$ cases, such as $U^*$ = 9.0, incommensurate frequencies are observed, resulting in a chaotic response. The trajectories of the displacements at $\alpha = 155^{\circ }$ exhibit all three types of vibrations: periodic, quasi-periodic and chaotic (figure 13b). At $U^*< 6.5$, the trajectories are figure-‘o’ shaped, with some cases showing slight irregularity. At $U^* = 7.0\unicode{x2013}10.0$, except $U^*= 8.5$ where the trajectory is periodic figure-‘8’ shaped, the trajectories become chaotic due to the emergence of incommensurate frequencies, as indicated in figure 12(c,d). When $U^*> 10.0$, the trajectories change into the figure-‘8’ shape.

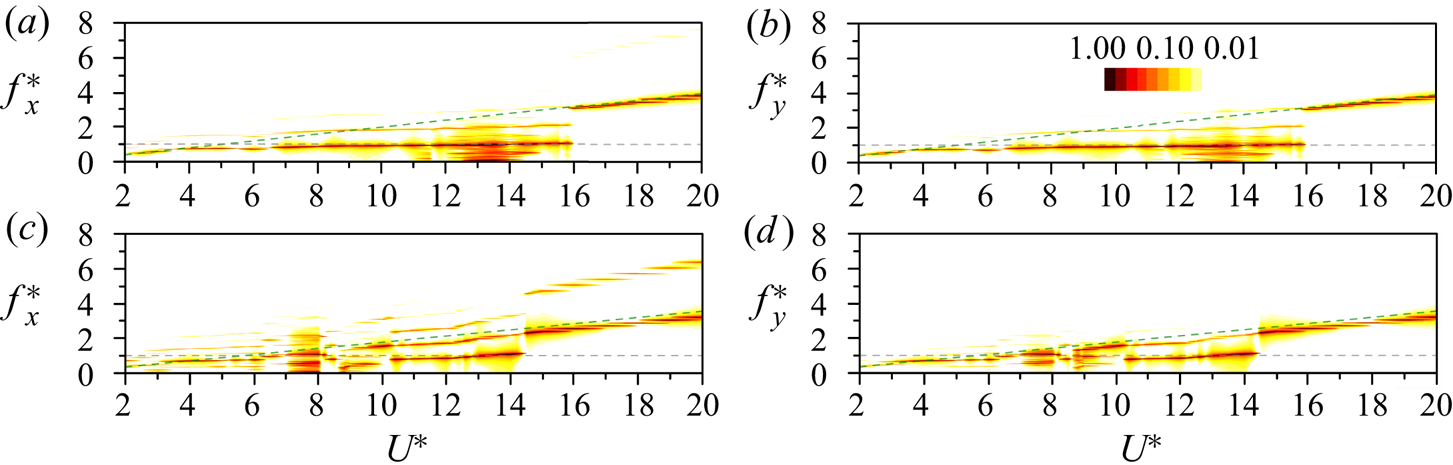
Figure 12. The PSD of the displacements in the streamwise and transverse directions at $U^* = 2\unicode{x2013}20$ and selected $\alpha$ cases. (a,b) First transition at $\alpha = 60^{\circ }$ and (c,d) second transition at $\alpha = 155^{\circ }$.

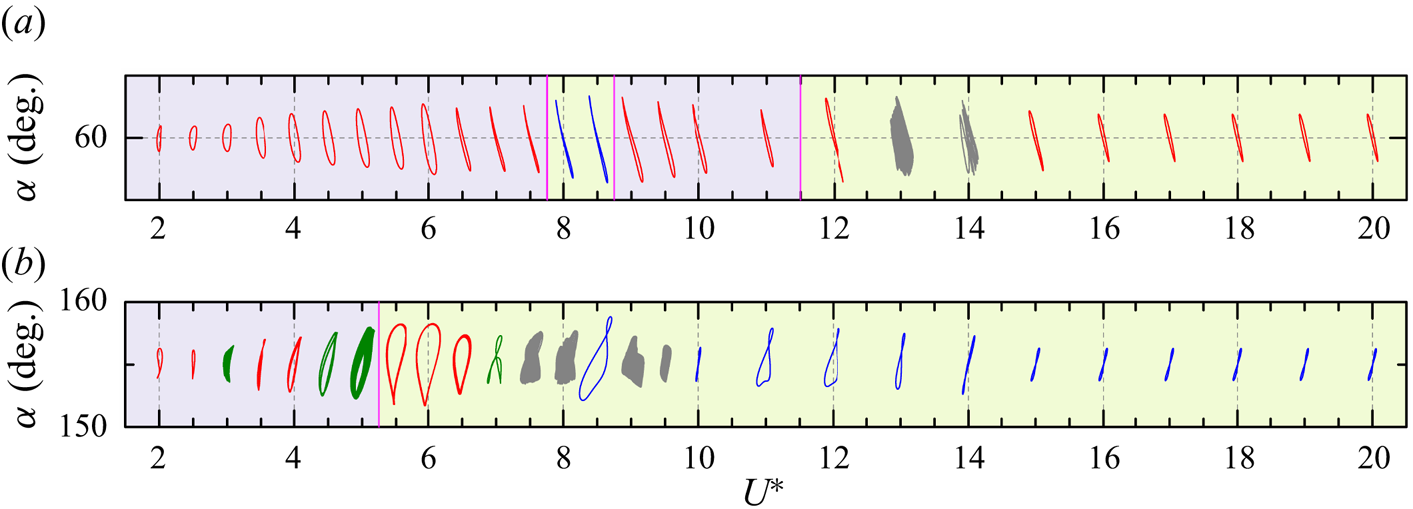
Figure 13. The trajectories of the streamwise and transverse displacements versus reduced velocity $U^*$ and angle of attack $\alpha$. (a) First transition and (b) second transition.

As shown in figure 14(b–e), the prism vibrations within the hysteresis loop are relatively regular. Out of the hysteresis, the vibrations in the first transition are periodic (figure 14a), but not periodic in the second transition, as indicated in figure 14( f–h).

Figure 14. Time histories of the streamwise and transverse displacements at different $U^*$ and $\alpha$ values. (a–c) First transition and (d–h) second transition.

4.1. Overview of wake modes

To some extent, the vortex dynamics behind the prism can be understood from the wake modes. It is worth investigating the connection between wake modes and vibration responses. Over the past decades, several different wake modes were recognized in the VIV of a circular cylinder, such as the 2S mode, P+S mode, 2P mode and 2T mode (Williamson & Roshko Reference Williamson and Roshko1988). These wake modes are linked to vibration and frequency responses (Williamson & Roshko Reference Williamson and Roshko1988; Govardhan & Williamson Reference Govardhan and Williamson2000; Leontini et al. Reference Leontini, Stewart, Thompson and Hourigan2006a). However, because of the changing asymmetry of the cross-section with $\alpha$, distinct responses from the classical VIV are observed and, hence, may be accompanied by distinct wake modes. Figure 15 shows how the wake modes are dependent on $U^*(= 2{-}20)$ and $\alpha$ ($= 0^{\circ }\unicode{x2013}180^{\circ }$). The wakes are categorized into a number of types; some of them are noticed in the VIV of a circular cylinder. Galloping responses involve more vortices shed from the D-section prism, especially when the amplitude is large. A special mode, i.e. $m$S+$n$S mode ($m$ and $n$ are positive integers; $m \ge 3$, $n \ge 2$), is introduced for the large-amplitude response (Seyed-Aghazadeh et al. Reference Seyed-Aghazadeh, Carlson and Modarres-Sadeghi2017; Chen et al. Reference Chen, Ji, Xu, Zhang and Wei2020a, Reference Chen, Ji, Alam, Xu, An and Tong2022b). Also following Williamson & Roshko (Reference Williamson and Roshko1988), $m$ and $n$ are the numbers of vortices shed from each side of the prism in one vibration period. In general, the 2S mode dominates a major part of the examined parametric plane, linked to the typical, hysteretic and narrowed VIV regimes and some other regimes with small amplitude and small $U^*$. However, owing to the asymmetric cross-section ($\alpha \neq 0^{\circ }, 180^{\circ }$), the arrangement of two vortices in the 2S mode is significantly different. As shown in figure 16(a), when the transverse distance between the separation points at two sides is large, vortices are arranged in two parallel rows alternately, generating the parallel vortex street at $x/D \leq 12.0$. The parallel vortex street transmutes into the secondary vortex street (Cimbala, Nagib & Roshko Reference Cimbala, Nagib and Roshko1988; Inoue & Yamazaki Reference Inoue and Yamazaki1999; Kumar & Mittal Reference Kumar and Mittal2012; Thompson et al. Reference Thompson, Radi, Rao, Sheridan and Hourigan2014; Zafar & Alam Reference Zafar and Alam2018; Jiang & Cheng Reference Jiang and Cheng2019; Zheng & Alam Reference Zheng and Alam2019; Shi, Alam & Bai Reference Shi, Alam and Bai2020; Jiang Reference Jiang2021). As shown in figure 16(b), at a higher $\alpha$, the transverse distance between the separation points on the two sides becomes small, as does the lateral distance between the two rows of the vortices in the wake. For a further larger $\alpha$ and large amplitude, although vortex shedding occurs in the 2S mode, streamwise distances between two successive counter-rotating vortices are unequal in the near wake and tend to be equal downstream (figure 16c). The arrangement of vortices looks symmetric in the near wake but asymmetric in the far wake. The mutation from the symmetric to asymmetric arises from the difference in the convective velocities in the upper- and lower-row vortices, given that the lower-row vortices, lying on the wake centreline, have smaller convective velocity than the upper-row vortices.

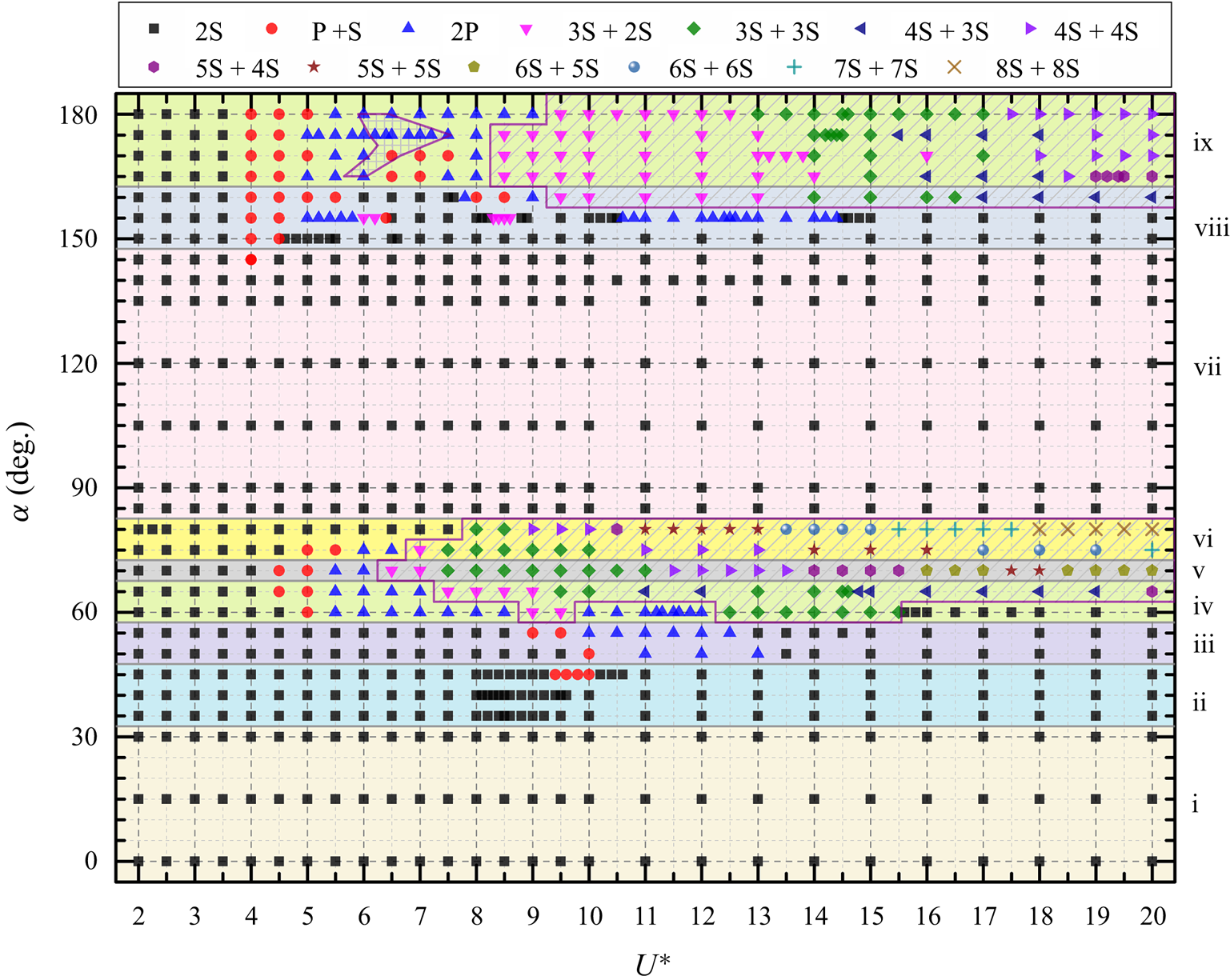
Figure 15. Wake modes in the $(U^*, \alpha )$ parameter plane for the 2DOF FIV of a D-section prism at ${Re} = 100$ and $m^* = 2.0$. Two regions marked by inclined lines represent the zones where the $m$S+$n$S mode appears and the region marked by straight lines denotes the intermittency response.

Figure 16. Selected vorticity contours for the wake modes shown in figure 15. (a) The 2S mode at $\alpha = 15^{\circ }$ and $U^* = 5.0$, (b) the 2S mode at $\alpha = 40^{\circ }$ and $U^* = 17.0$, (c) the 2S mode at $\alpha = 50^{\circ }$ and $U^*$ = 6.0, (d) the P+S mode at $\alpha = 160^{\circ }$ and $U^* = 4.5$, (e) the 2P mode at $\alpha = 155^{\circ }$ and $U^* = 5.0$, ( f) the 4S+4S mode at $\alpha = 180^{\circ }$ and $U^* = 19.0$ and (g,h) the P+S/2P mode at $\alpha = 165^{\circ }$ and $U^* = 5.5$. In ( f), ‘top’ and ‘bottom’ denote that the prism is at the top and bottom, respectively.

Compared with the 2S mode, the P+S mode usually appears in the region with higher amplitudes. The P+S mode in the hysteretic and extended VIV regimes occurs at relatively large $U^* (= 9.0{-}10.0)$ and in the transition, combined and pure galloping regimes, at small $U^* (= 4.0{-}5.5)$. That is, the P+S mode requires a stronger oscillation to make sure a shear layer rolls into two or more times in one oscillation cycle (Leontini et al. Reference Leontini, Stewart, Thompson and Hourigan2006a; Govardhan & Williamson Reference Govardhan and Williamson2000). As shown in figure 16(d), when the prism is at the bottom, one positive vortex shed from the lower side pairs with the negative vortex shed from the upper side of the prism, which generates a vortex pair P. The positive vortex in the pair is small and weak, while pushed toward the free-stream side by the strong rotation of the large negative vortices. The small positive vortex thus decays rapidly and loses its identity downstream. When the prism is at the top position, only one positive vortex is shed from the lower side of the prism. The vortex shedding thus occurs in the P+S mode. During the downstream evolution of the vortices, the two negative vortices coalesce and the single vortex grows in size, which in turn generates symmetric vortices.

The 2P mode prevails only in the transition, combined and galloping regimes. The $U^*$ for the 2P mode is generally higher than that for the P+S mode, which is consistent with the requirement of a higher amplitude to make sure more vortices shed from the prism (Govardhan & Williamson Reference Govardhan and Williamson2000). As shown in figure 16(e), in the 2P mode two vortices are shed from each side of the prism in one vibration period in a similar fashion to the paired vortex described above. The weak vortex of each pair rapidly dissipates while other vortices rearrange themselves downstream.

The multi-vortex mode, namely $m$S+$n$S mode, is observed in the transition and galloping (including galloping region of the combined regime) regimes. In the transition regime the $m$S+$n$S mode occurs around the peak amplitude, while in the galloping region the number of vortices increases with increasing amplitude. As shown in figure 16( f), when the prism moves from the bottom to the top, four vortices, i.e. two positive (vortices 1 and 2) and two negative (vortices 1’ and 2’) vortices, shed from the prism. Similarly, another four vortices shed during the other half-cycle of the oscillation (vortices 3, 3’, 4 and 4’). It leads to the 4S+4S mode. However, it should be pointed out here that the galloping instability is related to specifics of what is happening to the shear layer, not to vortex shedding modes. The dominant frequency has no relationship with the number of vortices being shed in the wake. If one is to define an axis pointing the prism forward motion, the wake would just appear to be a slowly wavering 2S mode, as described in Li et al. (Reference Li, Lyu, Kou and Zhang2019), Yao & Jaiman (Reference Yao and Jaiman2017) and Nemes et al. (Reference Nemes, Zhao, Lo Jacono and Sheridan2012).

Besides the abovementioned wake modes, there is another intermittent mode where different wake modes appear alternately at some combinations of $\alpha$ and $U^*$. The occurrence of this special mode is a result of the significant fluctuations of the displacement. As shown in figure 16(g,h), within different periods, the number of vortices shed from the prism can be three or four, signifying a P+S or 2P mode. This intermittent wake mode has also been noticed in the transverse-only FIV of a D-section prism in Chen et al. (Reference Chen, Ji, Alam, Xu, An and Tong2022b). However, to positively identify the $(U^*,\alpha )$ range for this wake mode in the examined parametric plane, a large number of consecutive snapshots are required, which is outside the scope of this paper. Therefore, the intermittent mode is not marked in figure 15.

4.2. Mechanisms for the identified responses

The physical mechanisms for some responses have been thoroughly explained in Chen et al. (Reference Chen, Ji, Alam, Xu, An and Tong2022b) for a D-section prism allowed to vibrate in the transverse direction only. The freedom to move in the streamwise direction shows no intrinsic modification of the mechanisms. In this section the focus is given to the mechanisms of several crucial phenomena identified exclusively in the 2DOF FIV responses, which will deepen our understanding of this FSI problem.

4.2.1. Mechanisms for the sustenance of hysteresis

Bistability is a well-known phenomenon in dynamic systems, characterized by the coexistence between two stable states. The hysteresis with one loop is a mode of bistable states that are mutually connected (Guidi & Goldbeter Reference Guidi and Goldbeter1997). The solution states are different when the control parameter ($U^*$ in the present study) is continously increased and decreased. As shown in figures 3, 7 and 11, when $U^*$ is increased, the system jumps from one branch of the steady states to another branch after a limit point. However, when $U^*$ is then decreased, the system jumps back at a different point. Depending on the initial conditions, i.e. increasing or decreasing $U^*$, the prism responses may be significantly different. For a vibration system without structural damping, the prism energy extracted from the fluid equals the energy dissipation caused by the viscosity in one vibration period. Therefore, the net energy input is zero. Intrinsically, hysteresis can be thought of as a system that can sustain at two different stable states by extracting different amounts of energy. Take the hysteresis at $\alpha = 40^{\circ }$ for example. In the increasing case, the prism's initial energy equals the dynamic energy of the prism at the lower $U^*$. However, in the decreasing case the initial energy equals the dynamic energy of the prism at the higher $U^*$, which is significantly smaller than that in the increasing case. Table 2 shows the observed hysteresis and factors for its sustenance in different responses. It can be divided into two: hysteresis at $\alpha < 90^{\circ }$ associated with shear layer reattachment and separation point movement while that at $\alpha > 90^{\circ }$ only relates to shear layer reattachment. To elucidate the associated flow physics, we select two cases: (1) $\alpha = 40^{\circ }$ and $U^* = 8.0$ and (2) $\alpha = 180^{\circ }$ and $U^* = 14.0$, representing hysteresis with different factors.

Table 2. A summary of hysteresis observed in the 2DOF vibrations of a D-section prism at ${Re} = 100$ and $m^* = 2.0$.

The work done by the fluid is an indicator of how the prism oscillation is sustained (Qin, Alam & Zhou Reference Qin, Alam and Zhou2017, Reference Qin, Alam and Zhou2019). As the transverse vibration of the prism is sustained by the lift force, our attention is paid to the lift coefficient in phase with the prism velocity. Note that the time-averaged lift ($\bar {C}_{L}$) and transverse position ($\bar {Y}$) are not zero in the asymmetric case ($\alpha \neq 0^{\circ }, 180^{\circ }$). Therefore, the prism oscillation with respect to the mean position, i.e. $Y^{\prime }$ (= $Y-\bar {Y}$), depends on the energy exchange between the prism and fluid. Following the definition in Bourguet & Lo Jacono (Reference Bourguet and Lo Jacono2014), the energy transfer is valued by the lift coefficient in phase with the prism velocity, which is defined as $C_{L,V}={\sqrt {2}\tilde {C}_LV}/{\sqrt {\overline {V^{2}}}}$, with $\tilde {C}_L = C_L-\bar {C}_L$ and $V=\dot {Y}$. Positive and negative $C_{L,V}$ indicate the fluid exciting and damping the oscillation, respectively. Consequently, physical mechanisms for the excitation and inhibition caused by the fluid can be analysed through the variations of vorticity fields and the lift coefficient in phase with the prism velocity.

As shown in figure 17(a,c), in the increasing and decreasing cases, the vibration amplitudes and frequencies at $\alpha = 40^{\circ }$ and $U^* = 8.0$ are different. The same is observed for the streamwise vibration. More evidence regarding the differences is displayed in figures 3(c,d) and 6(e, f). As shown in figure 17(a), when the prism moves from the top to the bottom, $C_{L,V}$ is first positive and then negative. On the other hand, when the prism moves back from the bottom, $C_{L,V}$ changes twice, i.e. positive $\rightarrow$ negative $\rightarrow$ positive $\rightarrow$ negative, which suggests significantly different vortex dynamics. Additionally, the magnitude of $C_{L,V}$ in the second half-period is much smaller than that in the first half-period. Especially, when the prism velocity grows (instant i), the lower shear layer remains attached to the curved surface and rolls over the flat surface while the upper shear layer separating from the upper corner rolls away from the prism. The pressure on the lower side is thus lower than that on the upper side, making positive $C_{L,V}$. After the prism passes through the mean position, the prism velocity decreases gradually. At instant ii, the upper shear layer is attached on both curved and flat surfaces while the lower shear layer rolls behind, which yields negative $C_{L,V}$. At instants iii and iv, the prism moves towards the mean position with growing velocity magnitude. The upper shear layer closely follows the upper curved and flat surfaces and the stagnation point shifts toward the upper corner. The lift force thus changes from positive to negative between instants iii and iv, resulting in positive and negative $C_{L,V}$, respectively.

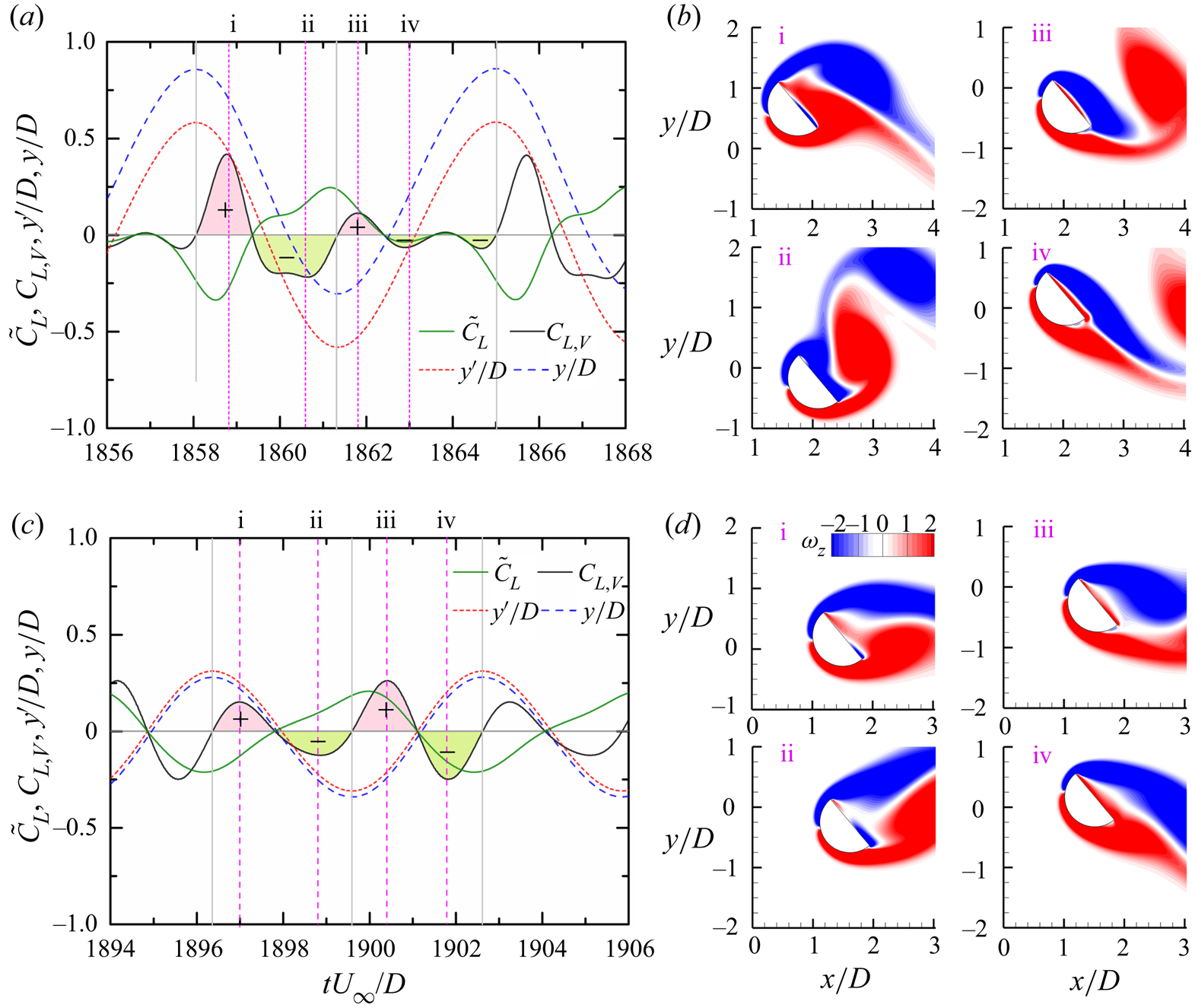
Figure 17. Time histories of the fluctuating lift coefficient ($\tilde {C}_L$, dashed line), lift coefficient in phase with the velocity ($C_{L,V}$, solid line), displacement ($y/D$ and $y^{\prime }/D$) and vorticity contours at marked instants at $\alpha = 40^{\circ }$ and $U^* = 8.0$. (a,b) For the increasing $U^*$ case and (c,d) for the decreasing $U^*$ case. The grey vertical lines in (a,c) denote the borders of the half-period.

In the decreasing case, however, the variation in $C_{L,V}$ becomes more regular (figure 17c). The $C_{L,V}$ is positive when the prism moves towards the mean position while negative when the prism moves away from the mean position. At instant i, as the prism moves toward the mean position, the lower shear layer is attached on the curved surface while the upper shear layer separates from the upper corner owing to the growing transverse velocity in the downward direction. The $C_{L,V}$ is thus positive. When the prism is below the mean position (instant ii), the upper and lower shear layers separate from the upper and lower corners, respectively, almost symmetrically. The lift force is positive, largely due to the negative pressure on the flat surface, resulting in negative $C_{L,V}$. In the following half-period, i.e. bottom $\rightarrow$ top, a similar change is observed. However, due to the influences of the separation (instant iii) and entrainment (to the flat part, instant iv) of the shear layer on the lower side, the magnitude of $C_{L,V}$ is larger than that in the former half-period (top $\rightarrow$ bottom).

From the above discussion, it is understood that in the increasing case, the upper shear layer largely reattaches onto the flat surface and the lower shear layer follows the curved surface (instants ii–iv). In the decreasing case only weak reattachments of the lower shear layer onto the flat part are observed (instants i, iv). In addition, the separation locations of the lower shear layer show discrepancies between the increasing and decreasing cases (figure 17b,d). These factors are essentially responsible for the sustenance of the hysteresis. Furthermore, we note that the prism vibration is synchronized with the vortex formation. The excitation and inhibition caused by the fluid are closely related to dynamic changes in the shear layers, such as reattachment, entrainment and separation. We can infer that the lock-in in the increasing case is largely associated with the shear layer reattachment, upholding our statement in § 3 for the occurrence of lock-in.

The second hysteresis discussed here is in the transverse-only galloping regime where the wake in both the increasing and decreasing cases is the 4S+4S mode and two distinctive states are related to the dynamic changes of shear layer reattachment. As shown in figure 18(a,b), $C_{L,V}$ is repeatable in each half-period, signifying that the vibration period is four times that of the lift responsible for the response. However, for the two states, the variations in $C_{L,V}$ are significantly different. In the increasing case, $C_{L,V}$ is firstly negative when the prism moves upward from the bottom and then positive as the prism is around the mean position (figure 18a). It changes into negative before the prism approaches the top. As shown in figure 18(c), the positive and negative $C_{L,V}$ are reflected by the shear layer reattachment modifications. For example, at instant ii, when the prism is moving toward the mean position, the upper shear layer closely follows most of the curved surface while the lower shear layer separates from the lower corner. Consequently, the pressure at the upper side is lower than that at the lower side, and the resultant lift is in phase with the prism velocity. The vibration is promoted by the energy extracted from the fluid. However, in the decreasing case the variation in $C_{L,V}$ is roughly opposite to that in the increasing case, suggesting distinct vortex dynamics relative to the prism motion from those in the increasing case. As shown in figure 18(d), when the prism moves back from the top, the lower shear layer remains attached on the entire curved surface (instant i), yielding positive $C_{L,V}$. However, when the prism is near the mean position (instant ii), the lower shear layer separates from the curved surface and there is an initiation of the upper shear layer rolling close to the upper corner. It corresponds to positive lift force and negative $C_{L,V}$, given that the prism velocity is negative. From the description, we can see that the two states are caused by the dynamic changes of shear layer reattachment.

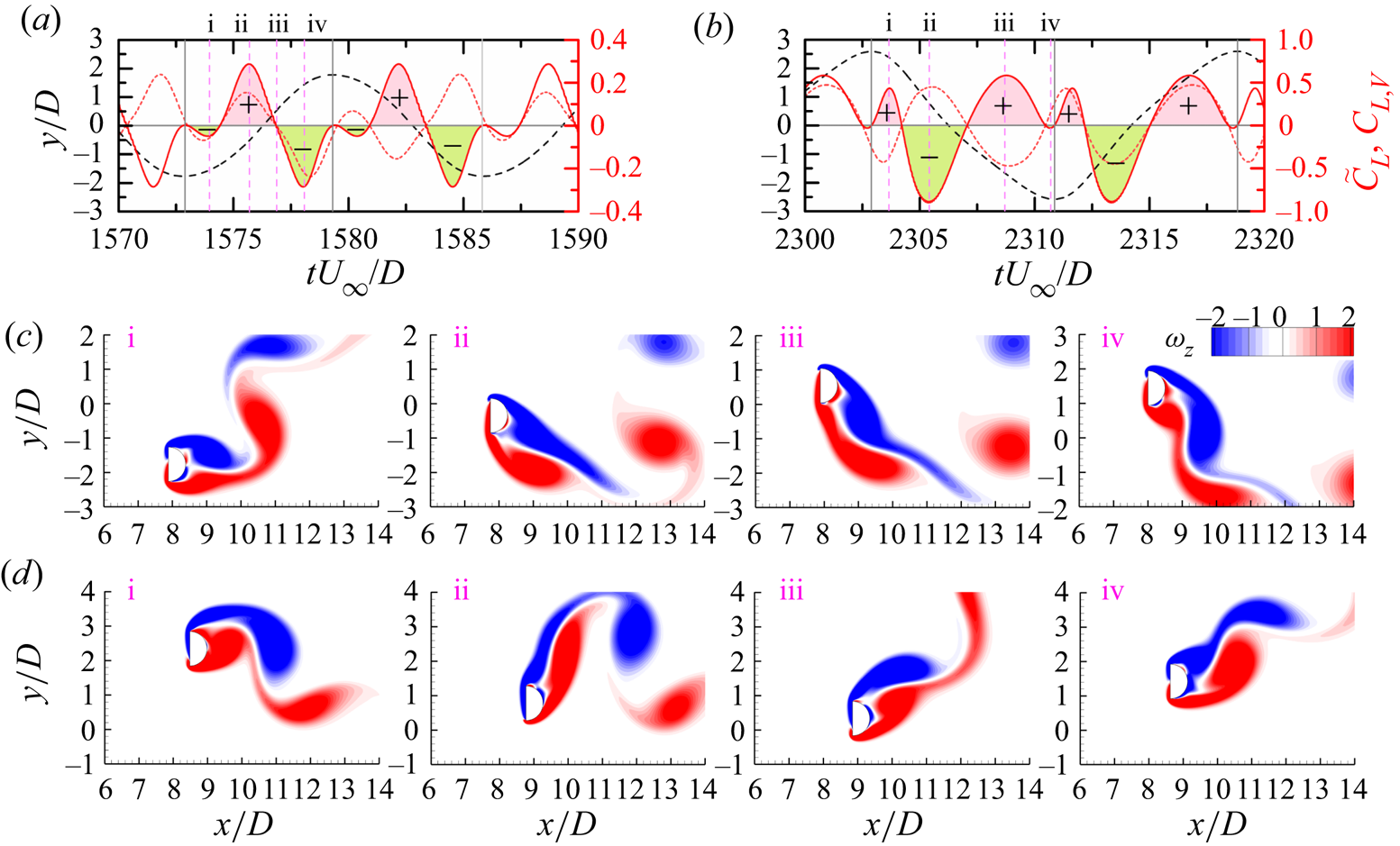
Figure 18. (a,b) Time histories of the fluctuating lift coefficient ($\tilde {C}_L$, dashed line), lift coefficient in phase with the prism velocity ($C_{L,V}$, solid line) and the displacement ($y/D$), and (c,d) vorticity contours at the instants marked in (a,b) at $\alpha = 180^{\circ }$ and $U^* = 14.0$. (a,c) For the increasing case and (b,d) for the decreasing case. In this case, the prism symmetry recovers and the mean displacement position ($\bar {Y}$) is zero. The grey vertical lines in (a,b) denote the borders of the half-period.

4.2.2. Mechanism for the intermittency in the transverse-only galloping

An intermittent response is noted at $\alpha = 165^{\circ }\unicode{x2013}180^{\circ }$ and $U^* = 5.7\unicode{x2013}7.5$, as marked by the straight lines in figure 15. In this section we aim to unearth the physical mechanism for the intermittent behaviour with the help of dynamic mode decomposition (DMD) and wavelet transform (WT). Dynamic mode decomposition is an effective method to capture flow modes of different frequencies (Rowley et al. Reference Rowley, Mezic, Bagheri, Schlatter and Henningson2009; Schmid Reference Schmid2010; Jovanovic, Schmid & Nichols Reference Jovanovic, Schmid and Nichols2014). Therefore, DMD can provide flow modes with pure frequency contents. In this paper we use the codes for the DMD analysis provided by Jovanovic et al. (Reference Jovanovic, Schmid and Nichols2014). In each DMD analysis, 400 snapshots are collected with a non-dimensional time interval of 0.2. Details of WT can be found in Chen et al. (Reference Chen, Ji, Xu, Liu and Campbell2015). Figure 19(a) shows the time histories of the streamwise and transverse displacements. We can see that the vibration changes intermittently between two different states. In state I the vibration is regular but with a smaller amplitude in the streamwise direction. The figure-‘8’ trajectory of the displacements shown in figure 19(b) further supports this fact. However, in state II the vibration is chaotic, as suggested by the chaotic trajectory of the displacements shown in figure 19(c). The wake modes in the two states are the P+S and 2P modes, respectively (figure 19b,c).

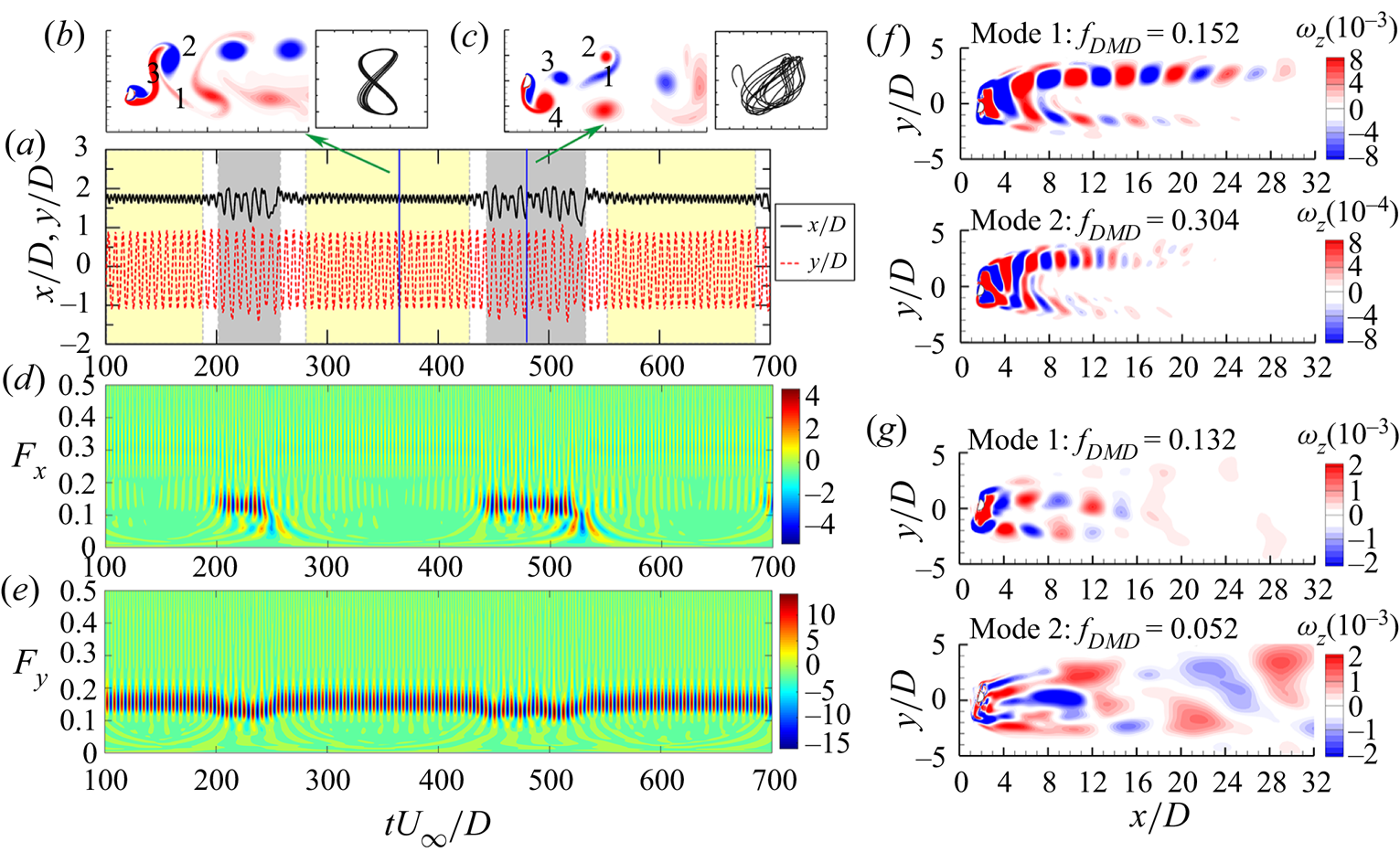
Figure 19. (a) Time histories of the streamwise and transverse displacements, (b,c) instantaneous vorticity contours and trajectories of the displacement for states I and II, (d,e) WT results of the streamwise and transverse displacements and ( f,g) DMD modes of states I and II, respectively, at $\alpha = 165^{\circ }$ and $U^* = 6.0$.

More information is provided by the WT analysis. In state I the streamwise vibration is dominated by the second harmonic frequency ($F_x = 0.304$), with its amplitude much higher than that of the fundamental frequency ($F_x = 0.152$), while the transverse vibration has only the fundamental frequency, which agrees well with the periodic response (figure 19d,e). In state II the dominant frequency ($F_y = 0.132$) of the transverse vibration is slightly lower than that in state I, while other frequencies are still insignificant. The fundamental frequency $F_x = 0.132$ appears for the streamwise vibration, in addition to a lower frequency $F_x = 0.052$ at the transition between states I and II. The DMD modes corresponding to the frequencies observed in WT analysis are shown in figure 19( f,g). In state I the mode at $f_{DMD} = 0.152$ clearly shows the typical Kármán vortex street. However, owing to the asymmetry of the cross-section shape to the incoming flow, vortices shed from the upper side of the prism are stronger than those from the lower side. Correspondingly, the extracted mode is asymmetric. The mode at $f_{DMD} = 0.304$ is of the second harmonic frequency, which is featured by doubled vortices in the wake. In state II, owing to the irregular arrangement of vortices, the mode at $f_{DMD} = 0.132$ dominated in the streamwise and transverse vibrations exhibits a Kármán-like vortex street; the mode at $f_{DMD} = 0.052$ is significantly different. As shown in figure 19(g), in this low-frequency mode the vorticity energy is stronger at $x/D \gtrsim 7.0$, which positively suggests that it comes from unstable vortex interactions in a relatively far wake. This is not surprising when considering the irregular vortex arrangement in state II. Since the vortex interactions are not stable, the developed low-frequency component can impact the vortex shedding through the feedback mechanism (Ho & Huerre Reference Ho and Huerre1984; Huerre & Monkewitz Reference Huerre and Monkewitz1990), which leads to vortex street modification gradually. That is, because of the unstable vortex interactions, the two states are neutrally unstable, and accordingly, they appear intermittently.

4.2.3. Mechanism for the dual galloping

As discussed in § 3.3, the prism at $\alpha = 70^{\circ }$ displays galloping responses in both streamwise and transverse directions. In this section we aim to uncover the physical mechanism for this dual galloping. The case at $\alpha = 70^{\circ }$ and $U^* = 8.0$ is selected. Here, it should be mentioned that due to the strong asymmetry of the cross-section to the incoming flow, the instantaneous pressure $C_p$ on the prism surface is not appropriate for evaluating the work done by the fluid, rather the time-averaged pressure $\bar {C}_{p}$ must be subtracted from $C_p$.

As shown in figure 20(a,b), both the streamwise and transverse displacements are relatively periodic, with large amplitudes, and the vibrations of the two directions show a strong coupling. Firstly, we focus on the streamwise galloping. When the prism moves upstream (instant i), the streamwise velocity increases gradually and the upper shear layer remains reattached on the flat surface before separating from the lower corner. Therefore, the fluctuating pressure $C_p-\bar {C}_{p}$ on the flat surface is much lower than that on the curved surface (figure 20e). The $C_{D,U}$ is thus highly negative. After the prism crosses the mean streamwise position (instant ii), the prism velocity reduces and the lower shear layer separates earlier, which leads to $C_p-\bar {C}_{p}$ on the curved surface decreased at $\theta < 62^{\circ }$. The upper shear layer elongates and rolls behind the lower vortex, leading to a higher $C_p-\bar {C}_{p}$ on the flat surface (figure 20c,e), and hence, a small positive $C_{D,U}$ until the prism reaches the upstream position (figure 20a). However, when the prism moves downstream (instant iii), the intensity of the lower shear layer reaches roughly the peak, and because of the decreased transverse velocity, a small part of the lower shear layer is entrained onto the flat surface (figure 20c). Accordingly, the upper shear layer is forced to separate from the upper corner. The $C_p-\bar {C}_{p}$ on the flat surface is thus further increased continuously while that on the curved surface decreases, especially at $\theta < 40^{\circ }$. Therefore, $C_{D,U}$ changes into a small negative. As the prism crosses the mean position (instant iv), the prism velocities in both directions decrease, and the lower shear layer partly moves further toward the flat surface and partly goes downstream. Therefore, the $C_p-\bar {C}_{p}$ on the flat surface notably increases for $y_i> -0.21$ while that at the curved surface goes significantly lower, especially at $\theta < 45^{\circ }$ and $\theta > 135^{\circ }$, thus resulting in a positive $C_{D,U}$.

Figure 20. (a,b) Time histories of the fluctuating drag coefficient ($\tilde {C}_D$), the drag coefficient in phase with the streamwise velocity ($C_{D,U}$), the streamwise displacement ($x^{\prime }/D$), the fluctuating lift coefficient ($\tilde {C}_L$), the lift coefficient in phase with the transverse velocity ($C_{L,V}$) and the transverse displacement ($y^{\prime }/D$), and (c,d) vorticity contours at instants marked in (a,b) at $\alpha = 70^{\circ }$ and $U^* = 8.0$. The time-averaged streamwise and transverse displacements are $\bar {X} = 1.075$ and $\bar {Y} = 0.271$, respectively, which are not removed in the vorticity fields shown in (c,d). (e, f) The fluctuating pressure coefficient ($C_p-\bar {C}_{p}$, where $C_p = (p-p_\infty )/0.5\rho U_\infty ^{2}$ is the instantaneous pressure on the surface and $\bar {C}_{p}$ is the time-averaged pressure on the surface) along the curved surface ($\theta = 0^{\circ }\sim 180^{\circ }$) and flat surface ($y_i = -0.5\sim 0.5$) at instants marked in (a,b). Here, $\theta = 0^{\circ }$ and $180^{\circ }$, rotating counterclockwise, are at the upper (P2) and lower (P1) intersection points, while $y_i$ = $-$0.5 and 0.5, positive upward, are at the lower (P1) and upper (P2) intersection points, respectively; see the subplot in ( f).

Then, we look at the transverse galloping. As shown in figure 20(b), when the prism moves toward the mean position (instant v), the transverse velocity rapidly increases and the upper shear layer remains attached on the flat surface. The lower shear layer covers a major part of the curved surface; therefore, the $C_p-\bar {C}_{p}$ is relatively weak, especially at $45^{\circ } < \theta < 150^{\circ }$ (figure 20f), thus leading to a negative $C_{L,V}$. When the prism crosses the transverse mean position (instant vi), the upper shear layer goes weaker while the lower shear layer develops (figure 20d). Accordingly, the $C_p-\bar {C}_{p}$ on the flat surface increases for $y_i< 0.15$ while that on the curved surface significantly decreases at $\theta < 45^{\circ }$ and increases at $45^{\circ } < \theta < 180^{\circ }$ (figure 20f). The $C_{L,V}$ becomes positive. At instant vii, the prism moves back from the top position and the lower shear layer is entrained onto the flat surface, which leads to $C_p-\bar {C}_{p}$ being roughly zero. The $C_p-\bar {C}_{p}$ on the curved surface remains positive in a wider region, i.e. $50^{\circ } < \theta < 135^{\circ }$, which is opposite to the prism transverse velocity, thus, $C_{L,V}$ being negative. As the prism moves downstream (instant viii), the $C_p-\bar {C}_{p}$ on the flat surface increases further for $y_i>$ $-$0.25 while the lower shear layer decreases greatly for $\theta > 90^{\circ }$. As a result, $C_{L,V}$ becomes strongly positive.

The above analysis indicates that galloping in both directions is associated with the dynamics of shear layer development, attachment and entrainment, which leads to significant pressure fluctuations and are responsible for the sustenance of large-amplitude vibrations. This is different from the dynamics in the transverse-only galloping where multiple shear layer reattachments appear along with manifold vortices (Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). To illuminate the modifications of the prism oscillation in sustaining the growing amplitude, figure 21 shows the dependence of the vibration amplitude on the phase lag ($\varphi _{xy}$) between the streamwise and transverse displacements. The phase lag was calculated directly using the Hilbert transform of the displacements in the streamwise and transverse directions (Khalak & Williamson Reference Khalak and Williamson1999; Prasanth & Mittal Reference Prasanth and Mittal2008). Generally, the dependence can be divided into four regions. When the vibration amplitude is small (region i), $\varphi _{xy}$ shows significant variations. However, when the amplitude is intermediate (region ii), $\varphi _{xy}$ decreases gradually. At the two regions (regions iii and iv) with large amplitudes, $\varphi _{xy}$ is approximately constant. In region iv the amplitude is significantly large and $\varphi _{xy}$ displays strong fluctuations, signifying a modulation to sustain higher amplitudes.

Figure 21. Dependence of the phase lag ($\varphi _{xy}$) between the streamwise and transverse displacements on (a) $A_x^{*}$ and (b) $A_y^{*}$ at $\alpha = 70^{\circ }$ for the dual galloping.

4.3. Dependence of wake modes on vibration amplitudes and frequencies

In this section we explore the dependence of wake modes on vibration amplitudes and frequencies. As discussed in § 3, in some $U^*$ cases, the transverse amplitude is comparable to the streamwise amplitude, such as in the transition response and dual galloping regimes. There may exist influences of the streamwise vibration on the wake modes. However, it is rather difficult to quantify the coupled impacts. In this study, $A_y^{*}$ and $f_y^{*}$ are applied to explore the dependence. For comparison, the dependence of wake modes in the 1DOF case is superimposed.

According to the compiled map of the circular cylinder wake for low Reynolds numbers given by Williamson & Govardhan (Reference Williamson and Govardhan2004), the 2S mode can persist up to the amplitude of 0.6 and beyond which the P+S mode dominates. Leontini et al. (Reference Leontini, Stewart, Thompson and Hourigan2006a) demonstrated that the critical amplitude for the transition from the 2S to P+S mode depends on ${Re}$. Govardhan & Williamson (Reference Govardhan and Williamson2000) showed that the shear layers of a circular cylinder with a higher amplitude are easier to be stretched and split into small parts, because of the intense strain rate field. In other words, more vortices shed from a vibrating circular cylinder require a higher amplitude. However, in the FIV of a non-circular prism, the wake dependence becomes different, due to the change of the cross-section shape to the incoming flow (Chen et al. Reference Chen, Ji, Xu, Zhang and Wei2020a, Reference Chen, Ji, Alam, Xu, An and Tong2022b). As shown in figure 22(a), the 2S mode occurs in a wide frequency range, depending on maximum $A_y^{*}$, which is similar to that of the 1DOF case. As the amplitude increases, the frequency range rapidly narrows and finally collapses to $f_y^{*} \approx 0.9$, falling inside the lock-in region. However, the largest amplitude for the 2S mode is 0.9, which is 50 % higher than that for a circular cylinder, suggesting the cross-section influences on the critical amplitude for the 2S mode.

Figure 22. Dependence of the wake modes on $A_y^{*}$ and $f_y^{*}$. The modes in (d) belong to the $m{\rm S}+ n{\rm S}$ mode where $m$ and $n$ are positive integers and $m + n \ge 5$. The open circles denote the wake distributions of the 1DOF case.

For the P+S mode, Williamson & Govardhan (Reference Williamson and Govardhan2004) believed it does not appear in the VIV of a circular cylinder for the reason that it does not deliver positive energy to excite a free vibration. However, owing to the symmetry breaking of the cross-section, the P+S mode is frequently encountered in the FIV of a non-circular prism (Nemes et al. Reference Nemes, Zhao, Lo Jacono and Sheridan2012; Wang et al. Reference Wang, Zhao, Yang and Yu2015; Zhao Reference Zhao2015; Seyed-Aghazadeh et al. Reference Seyed-Aghazadeh, Carlson and Modarres-Sadeghi2017). As shown in figure 22(b), similar to that of the 1DOF case, the amplitude for the P+S mode varies in the range of 0.25–1.3, slightly larger than that for the 2S mode. In the 1DOF case, the P+S mode occurs in a slightly wider amplitude range. The corresponding frequency varies around 0.6–1.2, significantly narrower than that for the 2S mode. This indicates that the P+S mode largely occurs in the lock-in region. As shown in figure 22(c), the dependence of the 2P mode is similar to that for the P+S mode. The amplitude for the 2P mode varies in the range of 0.25–1.36 while most of the cases are located at the region in which the amplitude is larger than 0.85. The occurrence of the 2P mode at the small amplitude (<0.6) indicates that the asymmetry could lower the amplitude requirement. The frequency of the 2P mode is always around 1.0, resting in the lock-in region.

The multi-vortex mode, i.e. $m$S+$n$S mode, occurs only in the transition response and three galloping regimes. As shown in figure 22(d), similar to the 1DOF case, the amplitude for the $m$S+$n$S mode covers a wide amplitude range of 0.16–2.85. The frequency for the $m$S+$n$S mode can be mainly separated into two, i.e. one is $f_y^{*} \approx 0.7$ while the other is $f_y^{*} \approx 1.0$, both falling inside the lock-in region. The former is in the dual galloping regime while the latter is in the transverse-only galloping regime. Although the amplitude in the region of $f_y^{*} \approx 0.7$ is smaller than that in the region of $f_y^{*} \approx 1.0$, the number ($m$,$n$) of vortices is higher.

5.1. Statistics of fluid forces

Figure 23 shows the dependence of the fluid forces on $U^*$ and $\alpha$. As shown in figure 23(a), there are two regions where the mean drag coefficient $\bar {C}_{D}$ is relatively large. The first region is in the typical and hysteretic VIV regimes where the curved surface points upstream, while the second region is in the second transition response and transverse-only galloping regimes where the flat surface faces upstream. The $\bar {C}_{D}$ values in the second region are much higher than those in the first region. This is expected as the flow velocity goes to zero when the flow encounters the flat surface and the pressure at the flat surface becomes higher, thus leading to a higher $\bar {C}_{D}$. A significant difference between these two regions is that in the first region the $\bar {C}_{D}$ value is amplitude dependent, the higher the amplitude, the higher the $\bar {C}_{D}$. However, in the second region the dependence of $\bar {C}_{D}$ on the amplitude is not straightforward, decreasing with $U^*$ (>6.0) although the amplitude enhances. With $\alpha$ increasing from $30^{\circ }$ to $90^{\circ }$ where the bluffness of the cross-section reduces, $\bar {C}_{D}$ for regimes ii–vii decreases, not much linked with the amplitude. As shown in figure 23(c), the mean lift coefficient $\bar {C}_{L}$ can be positive or negative, separated by $\alpha \approx 95^{\circ }$. At $\alpha \approx 180^{\circ }$, $\bar {C}_{L}$ is small and negative but still not zero, which stems from the asymmetric vortex shedding, as also reported by Wang et al. (Reference Wang, Zhao, Yang and Yu2015) and Chen et al. (Reference Chen, Ji, Xu, Zhang and Wei2020a). The positive $\bar {C}_{L}$ is more evident in the region where the amplitude is large, indicating the amplitude dependence. The negative $\bar {C}_{L}$ becomes significant at $\alpha = 125^{\circ }\unicode{x2013}145^{\circ }$, resting in the narrowed VIV regime. To some extent, $\bar {C}_{L}$ is largely $\alpha$ dependent as expected.

Figure 23. Dependence of the force coefficients on $U^* (= 2{-}20)$ and $\alpha$ ($= 0^{\circ }\unicode{x2013}180^{\circ }$): (a) $\bar {C}_{D}$, (b) $C_{D}^{\prime }$, (c) $\bar {C}_{L}$ and (d) $C_{L}^{\prime }$.

As shown in figure 23(b,d), the variations in $C_{D}^{\prime }$ and $C_{L}^{\prime }$ are alike. There are two remarkable regions where $C_{D}^{\prime }$ and $C_{L}^{\prime }$ are large. The first region ($U^* = 2.5\unicode{x2013}5.0$; $\alpha \approx 0^{\circ }\unicode{x2013}65^{\circ }$) lies in regimes i–iv, while the second region is at $U^* = 2.5\unicode{x2013}6.5$ and $\alpha = 135^{\circ }\unicode{x2013}180^{\circ }$, lying in regimes viii–ix. The large $C_{D}^{\prime }$ and $C_{L}^{\prime }$ correspond to the initial branch of VIV. Similar dependence was observed in the 1DOF case (Chen et al. Reference Chen, Ji, Alam, Xu, An and Tong2022b). The distributions of $C_{D}^{\prime }$ and $C_{L}^{\prime }$ show significant dependencies on $U^*$ but trivial dependencies on $\alpha$. The larger $C_{D}^{\prime }$ and $C_{L}^{\prime }$ occur at $U^*\approx 4.0\unicode{x2013}7.5$ and 2.5–5.5, respectively, while smaller $C_{D}^{\prime }$ and $C_{L}^{\prime }$ appear roughly in the narrowed VIV regime, as a result of the smaller amplitude and weaker fluctuation of shear layers. The galloping regimes are accompanied by small $C_{D}^{\prime }$ and $C_{L}^{\prime }$. This indicates that the excitation of the galloping is different from VIV, upheld by the results of Nemes et al. (Reference Nemes, Zhao, Lo Jacono and Sheridan2012), Zhao et al. (Reference Zhao, Leontini, Lo Jacono and Sheridan2014, Reference Zhao, Hourigan and Thompson2018) and Chen et al. (Reference Chen, Ji, Alam, Xu, An and Tong2022b).

5.2. Spectral analysis of fluid forces

Figure 24 shows the contours of dominant frequencies $f_D^{*}$ ($= f_D/f_n$) and $f_L^{*}$ ($= f_L/f_n$) obtained from PSD functions of $C_{D}^{\prime }$ and $C_{L}^{\prime }$, respectively. Generally, $f_D^{*}$ and $f_L^{*}$ are identical in most cases except in the typical VIV regime where $f_D^{*}$ is two times $f_L^{*}$ (Bearman Reference Bearman1984; Naudascher Reference Naudascher1987; Jauvtis & Williamson Reference Jauvtis and Williamson2004; Bourguet Reference Bourguet2020). As shown in figure 25(a,b), in the hysteretic VIV regime, both $f_D^{*}$ and $f_L^{*}$ increase linearly with increasing $U^*$, being $f_D^{*} = 2\times f_L^{*}$ except at $U^* \approx 6.0$ where the third harmonic lift frequency is dominant. At a higher $\alpha$, $f_D^{*}$ and $f_L^{*}$ are identical. An exception is discerned around the location where the phase lag shifts from $0^{\circ }$ to $180^{\circ }$. As shown in figure 25(c–h), the second harmonic lift frequency becomes energetic but still weaker than the fundamental one, i.e. the fundamental drag frequency is more energetic than the second harmonic frequency, although several harmonic frequencies appear. The $f_D^{*}$ and $f_L^{*}$ are thus predominantly identical.

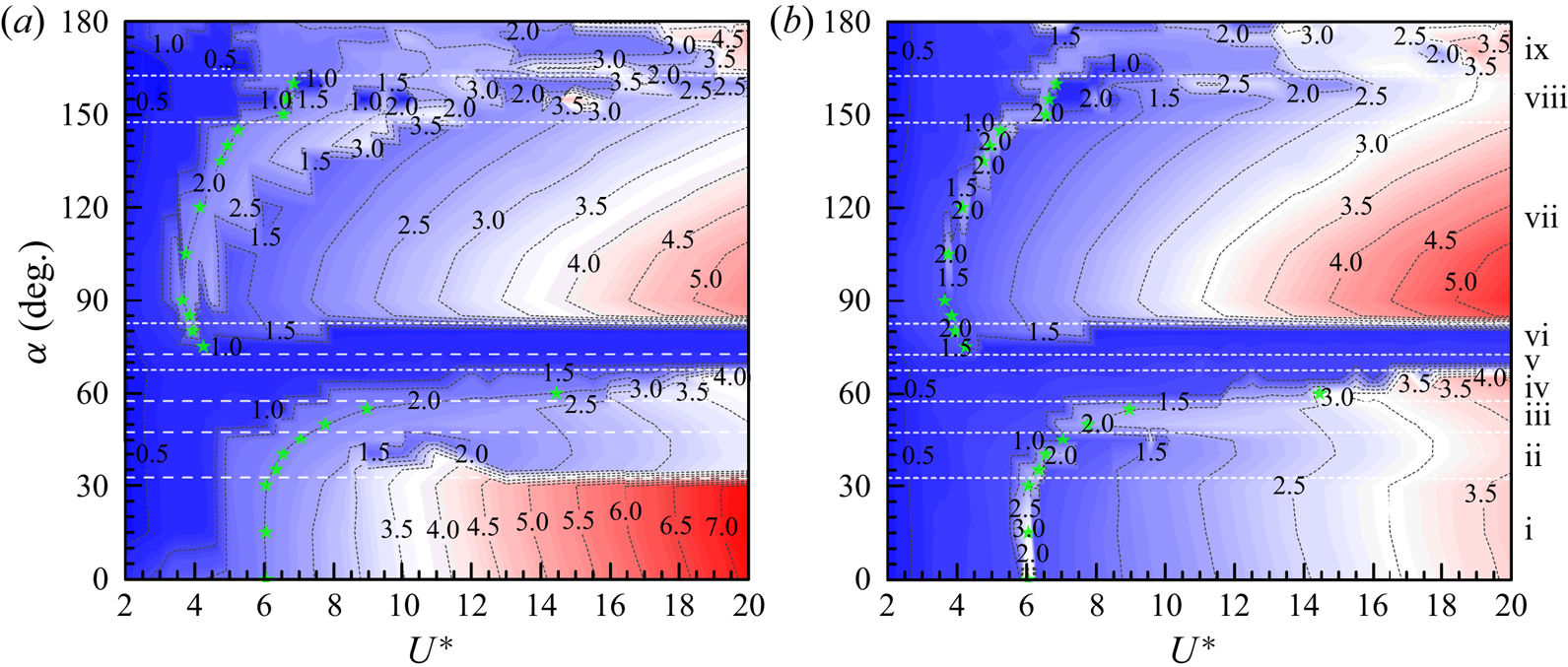
Figure 24. Dependence of (a) drag ($\,f_D^{*}$) and (b) lift ($\,f_L^{*}$) frequencies on $U^* (= 2{-}20)$ and $\alpha$ ($= 0^{\circ }\unicode{x2013}180^{\circ }$). The star symbols represent the location of the phase lag between the lift and displacement jumping from $0^{\circ }$ to $180^{\circ }$.

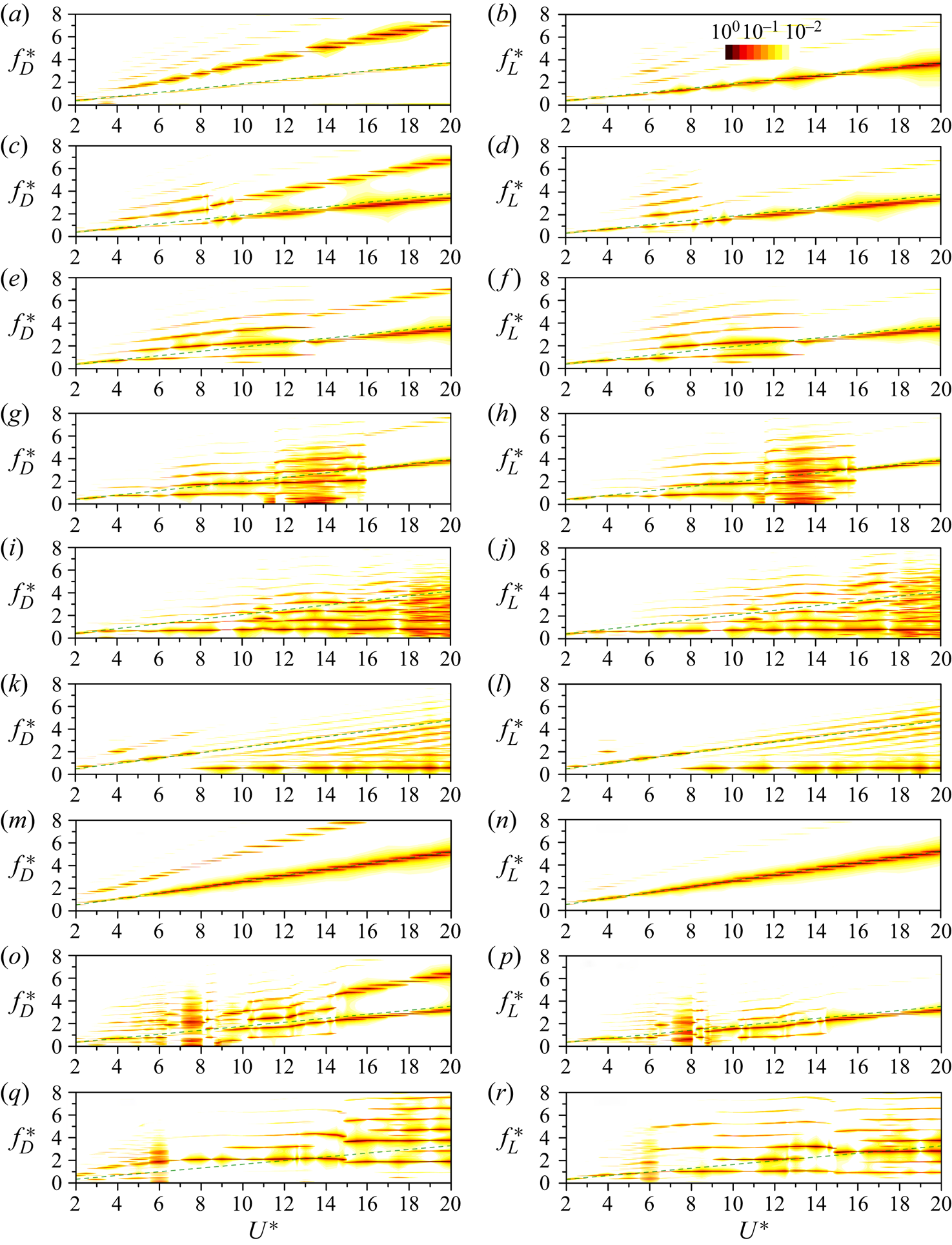
Figure 25. The PSD of the drag and lift coefficients at $U^* = 2\unicode{x2013}20$ and selected $\alpha$ values. (a,b) Typical VIV at $\alpha = 15^{\circ }$, (c,d) hysteretic VIV at $\alpha = 40^{\circ }$, (e, f) extended VIV at $\alpha = 50^{\circ }$, (g,h) first transition at $\alpha = 60^{\circ }$, (i,j) dual galloping at $\alpha = 70^{\circ }$, (k,l) combined VIV and galloping at $\alpha = 80^{\circ }$, (m,n) narrowed VIV at $\alpha = 105^{\circ }$, (o,p) second transition at $\alpha = 155^{\circ }$ and (q,r) transverse-only galloping at $\alpha = 180^{\circ }$.

In the dual galloping and combined response regimes, the identical $f_D^{*}$ and $f_L^{*}$ still stand, but multiple harmonic frequencies are detected (figure 25i–l). In the second transition response and transverse-only galloping regimes, the identical $f_D^{*}$ and $f_L^{*}$ are observed at the small $U^*$ while the relationship between $f_D^{*}$ and $f_L^{*}$ at large $U^*$ is complex, due to complicated vortex interactions in the near wake. As shown in figure 25(o–r), multiple frequencies emerge in the spectra of the drag and lift forces. In the transverse-only galloping regime, higher harmonic frequencies are noted as $U^*$ increases, owing to the growing number of vortices shed from the prism in each vibration cycle (Zhao et al. Reference Zhao, Leontini, Lo Jacono and Sheridan2014, Reference Zhao, Hourigan and Thompson2018; Seyed-Aghazadeh et al. Reference Seyed-Aghazadeh, Carlson and Modarres-Sadeghi2017; Chen et al. Reference Chen, Ji, Xu, Zhang and Wei2020a, Reference Chen, Ji, Alam, Xu, An and Tong2022b).

5.3. Phase lags between the lift and transverse displacement

For a vibration system without structural damping, the phase lag changing from $0^{\circ }$ to $180^{\circ }$ occurs at the position where the vibration frequency ($f_y^{*}$) passes through the unity (Sarpkaya Reference Sarpkaya2004). This way is adopted in the present study to confirm the location of the phase lag jump from $0^{\circ }$ to $180^{\circ }$, the same means adopted by Bourguet & Lo Jacono (Reference Bourguet and Lo Jacono2014). Figure 26 shows the variation of the phase lag between the lift and transverse displacement at different $\alpha$ cases. For comparison, the result of the 1DOF case is provided. It is seen that the variations in phase lag are very similar for the 1DOF and 2DOF cases. In the typical VIV regime the jump in phase lag occurs at a constant $U^*$. In the following three regimes, the phase lag jump appears at a higher $U^*$ as $\alpha$ increases. In the dual galloping regime the phase lag maintains around $0^{\circ }$, which has also been reported by Bourguet & Lo Jacono (Reference Bourguet and Lo Jacono2014), Zhao et al. (Reference Zhao, Leontini, Lo Jacono and Sheridan2014) and Chen et al. (Reference Chen, Ji, Alam, Xu, An and Tong2022b). However, in the combined VIV and galloping regime, the phase lag jump occurs at the VIV region. As $\alpha$ increases, the VIV dominates a wider $U^*$ region and the $U^*$ at which the phase lag jump occurs decreases gradually. The decreasing trend continues until $\alpha \approx 90^{\circ }$, and then the critical $U^*$ increases slowly with increasing $\alpha$. In the second transition response regime, the critical $U^*$ at which the phase lag jump occurs successively increases as $\alpha$ grows. In the transverse-only galloping the phase lag remains around $0^{\circ }$.

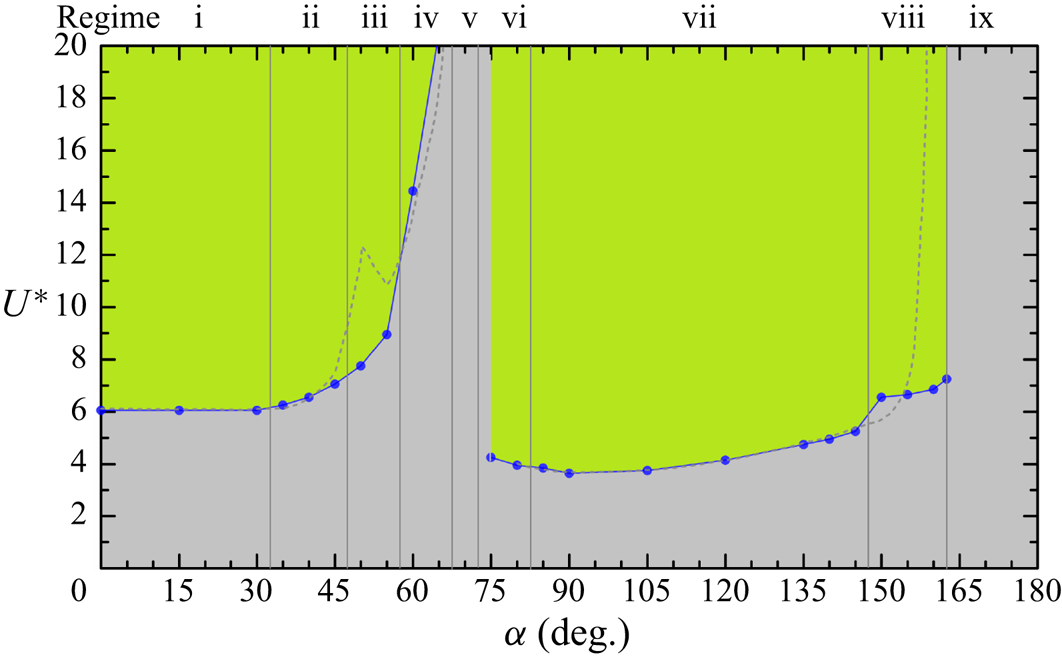
Figure 26. Phase lags ($\varphi$) between the lift and displacement in the 2DOF FIV of a D-section prism at examined ($U^*$, $\alpha$) plane. The grey and lime regions denote that the phase lag is approximately $0^{\circ }$ and $180^{\circ }$, respectively. The grey dashed line represents the phase lag variation in the 1DOF case.

The position of the corresponding $U^*$ at which the phase lag jump occurs is marked in figure 24. As shown in figure 24(b), the phase lag changing from $0^{\circ }$ to $180^{\circ }$ always occurs at the $U^*$ where the higher harmonic frequencies, such as the second or third harmonic frequency, are dominant. This indicates that the phase lag jump is associated with the appearance of higher harmonic frequencies (Prasanth & Mittal Reference Prasanth and Mittal2008; Chen et al. Reference Chen, Ji, Xu, Zhang and Wei2020a, Reference Chen, Ji, Xu and Zhang2022c).