Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-16T15:24:02.608Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent–laminar coexistence in wall flows with Coriolis, buoyancy or Lorentz forces

Published online by Cambridge University Press:  02 July 2012

G. Brethouwer ,
Y. Duguet  and
P. Schlatter
G. Brethouwer*
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
Y. Duguet
Affiliation:
LIMSI-CNRS, UPR 3251, Université Paris-Sud F-91403 Orsay, France
P. Schlatter
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Direct numerical simulations of subcritical rotating, stratified and magneto-hydrodynamic wall-bounded flows are performed in large computational domains, focusing on parameters where laminar and turbulent flow can stably coexist. In most cases, a regime of large-scale oblique laminar-turbulent patterns is identified at the onset of transition, as in the case of pure shear flows. The current study indicates that this oblique regime can be shifted up to large values of the Reynolds number by increasing the damping by the Coriolis, buoyancy or Lorentz force. We show evidence for this phenomenon in three distinct flow cases: plane Couette flow with spanwise cyclonic rotation, plane magnetohydrodynamic channel flow with a spanwise or wall-normal magnetic field, and open channel flow under stable stratification. Near-wall turbulence structures inside the turbulent patterns are invariably found to scale in terms of viscous wall units as in the fully turbulent case, while the patterns themselves remain large-scale with a trend towards shorter wavelength for increasing 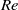. Two distinct regimes are identified: at low Reynolds numbers the patterns extend from one wall to the other, while at large Reynolds number they are confined to the near-wall regions and the patterns on both channel sides are uncorrelated, the core of the flow being highly turbulent without any dominant large-scale structure.

JFM classification

Instability: Transition to turbulence Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 704 , 10 August 2012 , pp. 137 - 172
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. del Álamo, J. C. & Jiménez, J. 2003 Spectra of the very large anisotropic scales in turbulent channels. Phys. Fluids 15, L41.CrossRefGoogle Scholar
2. del Álamo, J. C. & Jiménez, J. 2009 Estimation of turbulent convection velocities and corrections to Taylor’s approximation. J. Fluid Mech. 640, 5–26.CrossRefGoogle Scholar
3. Andereck, C. D., Lui, S. S. & Swinney, H. L. 1986 Flow regimes in a circular Couette system with independently rotating cylinders. J. Fluid Mech. 164, 155–183.CrossRefGoogle Scholar
4. Armenio, V. & Sarkar, S. 2002 An investigation of stably stratified turbulent channel flow using large-eddy simulation. J. Fluid Mech. 459, 1–42.CrossRefGoogle Scholar
5. Barkley, D. & Tuckerman, L. S. 2005 Computational study of turbulent laminar patterns in Couette flow. Phys. Rev. Lett. 94, 014502.CrossRefGoogle ScholarPubMed
6. Barkley, D. & Tuckerman, L. S. 2007 Mean flow of turbulent–laminar patterns in plane Couette flow. J. Fluid Mech. 576, 109–137.CrossRefGoogle Scholar
7. Boeck, T., Krasnov, D., Thess, A. & Zikanov, O. 2008 Large-scale intermittency of liquid-metal channel flow in a magnetic field. Phys. Rev. Lett. 101, 244501.CrossRefGoogle ScholarPubMed
8. Boeck, T., Krasnov, D. & Zienicke, E. 2007 Numerical study of magnetohydrodynamic channel flow. J. Fluid Mech. 572, 179–188.CrossRefGoogle Scholar
9. Chevalier, M., Schlatter, P., Lundbladh, A. & Henningson, D. S. 2007 A pseudo-spectral solver for incompressible boundary layer flows. Technical Report TRITA-MEK 2007:07, KTH Mechanics, Stockholm, Sweden.Google Scholar
10. Coles, D. 1965 Transition in circular Couette flow. J. Fluid Mech. 21, 385–425.CrossRefGoogle Scholar
11. Colovas, P. W. & Andereck, C. D. 1997 Turbulent bursting and spatiotemporal intermittency in the counterrotating Taylor–Couette system. Phys. Rev. E 55, 2736–2741.CrossRefGoogle Scholar
12. Dean, R. B. 1978 Reynolds number dependence of skin friction and other bulk flow variables in two-dimensional rectangular duct flow. Trans. ASME: J. Fluids Engng 100, 215–223.Google Scholar
13. Dong, S. 2009 Evidence for internal structure of spiral turbulence. Phys. Rev. E 80, 067301.CrossRefGoogle ScholarPubMed
14. Dong, S. & Zheng, X. 2011 Direct numerical simulation of spiral turbulence. J. Fluid Mech. 668, 150–173.CrossRefGoogle Scholar
15. Drazin, P. G. & Reid, W. H. 1981 Hydrodynamic and Hydromagnetic Stability. Oxford University Press.Google Scholar
16. Duguet, Y., Schlatter, P. & Henningson, D. S. 2010 Formation of turbulent patterns near the onset of transition in plane Couette flow. J. Fluid Mech. 650, 119–129.CrossRefGoogle Scholar
17. Emmons, H. W. 1951 The laminar–turbulent transition in a boundary layer. J. Aerosp. Sci. 18, 490–498.Google Scholar
18. Flores, O. & Riley, J. J. 2011 Analysis of turbulence collapse in the stably stratified surface layer using direct numerical simulation. Boundary-Layer Meteorol. 139, 241–259.CrossRefGoogle Scholar
19. Fukudome, K., Iida, O. & Nagano, Y. 2009 The mechanism of energy transfer in turbulent Poiseuille flow at very low Reynolds number. In Proceedings of 6th International Symposium on Turbulence and Shear Flow Phenomena, pp. 471–476.CrossRefGoogle Scholar
20. Gage, K. S. & Reid, W. H. 1968 The stability of thermally stratified plane Poiseuille flow. J. Fluid Mech. 33, 21–32.CrossRefGoogle Scholar
21. García-Villalba, M. & del Álamo, J. C. 2011 Turbulence modification by stable stratification in channel flow. Phys. Fluids 23, 045104.CrossRefGoogle Scholar
22. Hashimoto, S., Hasobe, A., Tsukahara, T., Kawaguchi, Y. & Kawamura, H. 2009 An experimental study on turbulent-stripe structure in transitional channel flow. In Proceedings of the Sixth International Symposium on Turbulence, Heat and Mass Transfer, pp. 193–196. Begell House Inc.Google Scholar
23. Komminaho, J., Lundbladh, A. & Johansson, A. V. 1996 Very large structures in plane turbulent Couette flow. J. Fluid Mech. 320, 259–285.CrossRefGoogle Scholar
24. Komori, S., Ueda, H., Ogino, F. & Mizushina, T. 1983 Turbulence structures in stably stratified open-channel flow. J. Fluid Mech. 130, 13.CrossRefGoogle Scholar
25. Krasnov, D., Rossi, M., Zikanov, O. & Boeck, T. 2008a Optimal growth and transition to turbulence in channel flow with spanwise magnetic field. J. Fluid Mech. 596, 73–101.CrossRefGoogle Scholar
26. Krasnov, D., Zikanov, O., Schumacher, J. & Boeck, T. 2008b Magnetohydrodynamic turbulence in a channel with spanwise magnetic field. Phys. Fluids 20, 095105.CrossRefGoogle Scholar
27. Lee, D. & Choi, H. 2001 Magnetohydrodynamic turbulent flow in a channel at low magnetic Reynolds number. J. Fluid Mech. 439, 367–394.CrossRefGoogle Scholar
28. Lesieur, M., Yanase, S. & Métais, O. 1991 Stabilizing and destabilizing effects of a solid-body rotation on quasi-two-dimensional shear layers. Phys. Fluids A 3, 403.CrossRefGoogle Scholar
29. Lezius, D. K. & Johnston, J. P. 1976 Roll-cell instabilities in rotating laminar and turbulent channel flows. J. Fluid Mech. 77, 153–175.CrossRefGoogle Scholar
30. Li, Q., Schlatter, P., Brandt, L. & Henningson, D. S. 2009 DNS of a spatially developing turbulent boundary layer with passive scalar transport. Intl J. Heat Fluid Flow 30, 916–929.CrossRefGoogle Scholar
31. Li, Q., Schlatter, P. & Henningson, D. S. 2008 Spectral simulations of wall-bounded flows on massively parallel computers. Tech. Rep., KTH Mechanics, Stockholm, Sweden. In Licentiate Thesis of Q. Li, 2009.Google Scholar
32. Lingwood, R. J. & Alboussière, T. 1999 On the stability of the Hartmann layer. Phys. Fluids 11, 2058–2068.CrossRefGoogle Scholar
33. Mahrt, L. 1999 Stratified atmospheric boundary layers. Boundary-Layer Meteorol. 90, 375–396.CrossRefGoogle Scholar
34. Meseguer, A., Mellibovsky, F., Avila, M. & Marques, F. 2009 Instability mechanisms and transition scenarios of spiral turbulence in Taylor–Couette flow. Phys. Rev. E 80, 046315.CrossRefGoogle ScholarPubMed
35. Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10, 496–508.CrossRefGoogle Scholar
36. Moser, R. D., Kim, J. & Mansour, N. N. 1999 Direct numerical simulation of turbulent channel flow up to . Phys. Fluids 11, 943–945.CrossRefGoogle Scholar
37. Philip, J. & Manneville, P. 2011 From temporal to spatiotemporal dynamics in transitional plane Couette flow. Phys. Rev. E 83, 036308.CrossRefGoogle ScholarPubMed
38. Prigent, A. 2001 PhD thesis, University Paris-Sud.Google Scholar
39. Prigent, A., Grégoire, G., Chaté, H. & Dauchot, O. 2003 Long-wavelength modulation of turbulent shear flows. Physica D 174, 100–113.CrossRefGoogle Scholar
40. Prigent, A., Grégoire, G., Chaté, H., Dauchot, O. & van Saarloos, W. 2002 Large-scale finite-wavelength modulation within turbulent shear flows. Phys. Rev. Lett. 89, 014501.CrossRefGoogle ScholarPubMed
41. Ptasinski, P. K., Boersma, B. J., Nieuwstadt, F. T. M., Hulsen, M. A., Van den Brule, B. H. A. A. & Hunt, J. C. R. 2003 Turbulent channel flow near maximum drag reduction: simulations, experiments and mechanisms. J. Fluid Mech. 490, 251–291.CrossRefGoogle Scholar
42. Reynolds, O. 1883 An experimental investigation of the circumstances which determine whether the motion of water shall be direct or sinuous and of the law of resistance in parallel channels. Phil. Trans. R. Soc. 174, 935–982.Google Scholar
43. Robertson, J. M. & Johnson, H. F. 1970 Turbulence structure in plane Couette flow. ASCE J. Engng Mech. Div. 96, 1171–1182.CrossRefGoogle Scholar
44. Rolland, J. & Manneville, P. 2011 Pattern fluctuations in transitional plane Couette flow. J. Stat. Phys. 142, 577–591.CrossRefGoogle Scholar
45. Romanov, V. A. 1973 Stability of plane-parallel Couette flow. Funct. Anal. Appl. 7, 137–146.CrossRefGoogle Scholar
46. Schlatter, P., Örlü, R., Brethouwer, G., Fransson, J. H. M., Johansson, A. V., Alfredsson, P. H. & Henningson, D. S. 2009 Turbulent boundary layers up to studied through simulation and experiment. Phys. Fluids 21, 051702.CrossRefGoogle Scholar
47. Takashima, M. 1996 The stability of the modified plane Poiseuille flow in the presence of a transverse magnetic field. Fluid Dyn. Res. 17, 293–310.CrossRefGoogle Scholar
48. Tuckerman, L. & Barkley, D. 2011 Patterns and dynamics in transitional plane Couette flow. Phys. Fluids 23, 041301.CrossRefGoogle Scholar
49. Tsukahara, T., Seki, Y., Kawamura, H. & Tochio, D. 2005 DNS of turbulent channel flow at very low Reynolds numbers. In Proceedings of the 4th Intl Symposium on Turbulence and Shear Flow Phenomena, pp. 935–940.CrossRefGoogle Scholar
50. Tsukahara, T., Tillmark, N. & Alfredsson, P. H. 2010 Flow regimes in a plane Couette flow with system rotation. J. Fluid Mech. 648, 5–33.CrossRefGoogle Scholar
51. Van Atta, C. W. 1966 Exploratory measurements in spiral turbulence. J. Fluid Mech. 25, 495–512.CrossRefGoogle Scholar
52. Wygnanski, I. J. & Champagne, F. H. 1973 On transition in a pipe. Part 1. The origin of puffs and slugs and the flow in a turbulent slug. J. Fluid Mech. 59, 281–335.CrossRefGoogle Scholar