Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-17T11:22:28.908Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent bubble fountains

Published online by Cambridge University Press:  11 December 2017

Martin C. Lippert  and
Andrew W. Woods Open the ORCID record for Andrew W. Woods [Opens in a new window]
Martin C. Lippert*
Affiliation:
BP Institute for Multiphase Flow, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
Andrew W. Woods
Affiliation:
BP Institute for Multiphase Flow, University of Cambridge, Madingley Road, Cambridge CB3 0EZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We explore the dynamics of turbulent bubble fountains produced when a descending stream of fresh water and air bubbles issues from a nozzle submerged in a tank of water. The bubbles have diameters of 2 to 5 mm and the fountains have source Froude numbers ranging from 10 to 240. The Reynolds numbers of the bubbly fountains range from 4000 to 24 000. The bubbles, carried into the tank by the downward jet of water, lead to a buoyancy force which reduces the downward momentum of the jet, thus producing a fountain. We find that $H_{F}$, the downward penetration distance of the bubbles into the water reservoir, may be characterised by two parameters: $\unicode[STIX]{x1D6EC}$, the ratio of the bubble rise speed to the characteristic fountain velocity, 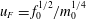$u_{F}=f_{0}^{1/2}/m_{0}^{1/4}$, and 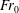$Fr_{0}$, the source Froude number, given by 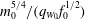$m_{0}^{5/4}/(q_{W_{0}}f_{0}^{1/2})$, where 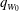$q_{W_{0}}$, 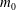$m_{0}$ and 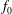$f_{0}$ are the source volume, momentum and buoyancy fluxes. As 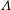$\unicode[STIX]{x1D6EC}$ increases, 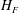$H_{F}$ decreases, a result which is directly analogous to the height of rise of particles in a particle-laden fountain (Mingotti & Woods, J. Fluid Mech., vol. 793, 2016, R1). Also, we find that 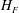$H_{F}$ increases as 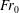$Fr_{0}$ increases, a result directly analogous to single-phase fountains (Turner, J. Fluid Mech., vol. 26, 1966, pp. 779–792). We present a model for the conservation of volume, momentum and buoyancy fluxes and use this to predict the penetration distance of the bubbles corresponding to that point at which the fountain liquid velocity equals the bubble rise speed. Using the best-fit value for the entrainment coefficient, 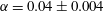$\unicode[STIX]{x1D6FC}=0.04\pm 0.004$, we find that our experimental measurements of the bubble penetration distance are in good accord with the model predictions for 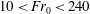$10<Fr_{0}<240$ and 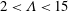$2<\unicode[STIX]{x1D6EC}<15$. In our experiments the bubble rise speed, 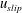$u_{slip}$, is large compared to the entrainment velocity of the descending fountain. Thus, only a small fraction of the rising bubbles are re-entrained, and so the buoyancy flux of the fountain is approximately independent of depth. Flow-visualisation experiments also show that the liquid momentum flux is not exhausted at the point of bubble separation and so the liquid in the fountain continues to travel downward, separated from the bubbles. We use the new theoretical model to estimate the flux of air entrained into plunging water jets.

JFM classification

Wakes/Jets: Jets Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 836 , 10 February 2018 , pp. 277 - 303
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baines, W. D. & Turner, J. S. 1969 Turbulent buoyant convection from a source in a confined region. J. Fluid Mech. 37, 51–80.CrossRefGoogle Scholar
Biń, A. 1993 Gas entrainment by plunging liquid jets. Chem. Engng Sci. 48, 3585–3630.Google Scholar
Bloomfield, L. J. & Kerr, R. C. 2000 A theoretical model of a turbulent fountain. J. Fluid Mech. 424, 197–216.CrossRefGoogle Scholar
Burridge, H. C. & Hunt, G. R. 2013 The rhythm of fountains: the length and time scales of rise height fluctuations at low and high Froude numbers. J. Fluid Mech. 728, 91–119.CrossRefGoogle Scholar
Carazzo, G., Kaminski, E. & Tait, S. 2010 The rise and fall of turbulent fountains: a new model for improved quantitative predictions. J. Fluid Mech. 657, 265–284.Google Scholar
Clanet, C. & Lasheras, J. C. 1997 Depth of penetration of bubbles entrained by a plunging water jet. Phys. Fluids 9, 1864–1866.CrossRefGoogle Scholar
Clift, R., Grace, J. R. & Weber, M. E. 2005 Bubbles, Drops, and Particles. Courier Corporation.Google Scholar
Hunt, G. R. & Burridge, H. C. 2015 Fountains in industry and nature. Annu. Rev. Fluid Mech. 47, 195–220.Google Scholar
McDougall, T. J. 1978 Bubble plumes in stratified environments. J. Fluid Mech. 85, 655–672.Google Scholar
McDougall, T. J. 1981 Negatively buoyant vertical jets. Tellus 33, 313–320.Google Scholar
Mingotti, N. & Woods, A. W. 2016 On turbulent particle fountains. J. Fluid Mech. 793, R1.Google Scholar
Morton, B. R., Taylor, G. & Turner, J. S. 1956 Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. Lond. A 234, 1–23.Google Scholar
Nemoto, S., Kawata, K., Kuribayashii, T., Akiyama, K., Kawai, H. & Murakawai, H. 1997 A study of engine oil aeration. JSAE Review 18, 271–276.CrossRefGoogle Scholar
Neto, I. E. L., Cardoso, S. S. S. & Woods, A. W. 2016 On mixing a density interface by a bubble plume. J. Fluid Mech. 802, R3.Google Scholar
Ohkawa, A., Kusabiraki, D., Kawai, Y., Sakai, N. & Endoh, K. 1986 Some flow characteristics of a vertical liquid jet system having downcomers. Chem. Engng Sci. 41 (9), 2347–2361.Google Scholar
Schlichting, H. 1979 Boundary-Layer Theory. McGraw-Hill.Google Scholar
Turner, J. S. 1966 Jets and plumes with negative or reversing buoyancy. J. Fluid Mech. 26, 779–792.CrossRefGoogle Scholar
Turton, R. & Levenspiel, O. 1986 A short note on the drag correleation for spheres. Powder Technol. 47, 83–86.Google Scholar
Van de Donk, J. A. C. 1981 Water Aeration with Plunging Jets. TU Delft, Delft University of Technology.Google Scholar
Williamson, N., Srinarayana, N., Armfield, S. W., McBain, G. D. & Lin, W. 2008 Low-Reynolds-number fountain behaviour. J. Fluid Mech. 608, 297–317.Google Scholar