Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-01-09T22:08:30.316Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent boundary layers at moderate Reynolds numbers: inflow length and tripping effects

Published online by Cambridge University Press:  21 August 2012

Philipp Schlatter  and
Ramis Örlü
Philipp Schlatter*
Affiliation:
Linné FLOW Centre and Swedish e-Science Research Centre (SeRC), KTH Mechanics, SE-100 44 Stockholm, Sweden
Ramis Örlü
Affiliation:
Linné FLOW Centre and Swedish e-Science Research Centre (SeRC), KTH Mechanics, SE-100 44 Stockholm, Sweden
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A recent assessment of available direct numerical simulation (DNS) data from turbulent boundary layer flows (Schlatter & Örlü, J. Fluid Mech., vol. 659, 2010, pp. 116–126) showed surprisingly large differences not only in the skin friction coefficient or shape factor, but also in their predictions of mean and fluctuation profiles far into the sublayer. While such differences are expected at very low Reynolds numbers and/or the immediate vicinity of the inflow or tripping region, it remains unclear whether inflow and tripping effects explain the differences observed even at moderate Reynolds numbers. This question is systematically addressed by re-simulating the DNS of a zero-pressure-gradient turbulent boundary layer flow by Schlatter et al. (Phys. Fluids, vol. 21, 2009, art. 051702). The previous DNS serves as the baseline simulation, and the new DNS with a range of physically different inflow conditions and tripping effects are carefully compared. The downstream evolution of integral quantities as well as mean and fluctuation profiles is analysed, and the results show that different inflow conditions and tripping effects do indeed explain most of the differences observed when comparing available DNS at low Reynolds number. It is further found that, if transition is initiated inside the boundary layer at a low enough Reynolds number (based on the momentum-loss thickness) 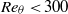, all quantities agree well for both inner and outer layer for 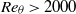. This result gives a lower limit for meaningful comparisons between numerical and/or wind tunnel experiments, assuming that the flow was not severely over- or understimulated. It is further shown that even profiles of the wall-normal velocity fluctuations and Reynolds shear stress collapse for higher 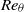irrespective of the upstream conditions. In addition, the overshoot in the total shear stress within the sublayer observed in the DNS of Wu & Moin (Phys. Fluids, vol. 22, 2010, art. 085105) has been identified as a feature of transitional boundary layers.

JFM classification

Instability: Transition to turbulence Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 710 , 10 November 2012 , pp. 5 - 34
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Alfredsson, P. H., Johansson, A. V., Haritonidis, J. H. & Eckelmann, H. 1988 The fluctuating wall-shear stress and the velocity field in the viscous sublayer. Phys. Fluids 31, 1026–1033.CrossRefGoogle Scholar
2. Alfredsson, P. H., Örlü, R. & Schlatter, P. 2011 The viscous sublayer revisited – exploiting self-similarity to determine the wall position and friction velocity. Exp. Fluids 51, 271–280.CrossRefGoogle Scholar
3. Araya, G., Jansen, K. & Castillo, L. 2009 Inlet condition generation for spatially developing turbulent boundary layers via multiscale similarity. J. Turbul. 10 (36), 1–33.CrossRefGoogle Scholar
4. Bertolotti, F. P., Herbert, T. & Spalart, P. R. 1992 Linear and nonlinear stability of the Blasius boundary layer. J. Fluid Mech. 242, 441–474.CrossRefGoogle Scholar
5. Brandt, L., Schlatter, P. & Henningson, D. S. 2004 Transition in boundary layers subject to free stream turbulence. J. Fluid Mech. 517, 167–198.CrossRefGoogle Scholar
6. Buschmann, M. H. & Gad-el-Hak, M. 2010 Normal and cross-flow Reynolds stresses: differences between confined and semi-confined flows. Exp. Fluids 49, 213–223.CrossRefGoogle Scholar
7. Buschmann, M. H., Indinger, T. & Gad-el-Hak, M. 2009 Near-wall behaviour of turbulent wall-bounded flows. Intl J. Heat Fluid Flow 30, 993–1006.CrossRefGoogle Scholar
8. Castillo, L. & Johansson, T. G. 2002 The effects of the upstream conditions on a low Reynolds number turbulent boundary layer with zero pressure-gradient. J. Turbul. 3, 1–19.CrossRefGoogle Scholar
9. Chauhan, K. A., Monkewitz, P. A. & Nagib, H. M. 2009 Criteria for assessing experiments in zero pressure-gradient boundary layers. Fluid Dyn. Res. 41, 021404.CrossRefGoogle Scholar
10. Chevalier, M., Schlatter, P., Lundbladh, A. & Henningson, D. S. 2007 SIMSON – A pseudo-spectral solver for incompressible boundary layer flow. Tech. Rep. TRITA-MEK 2007:07, Royal Institute of Technology, Stockholm, Sweden.Google Scholar
11. Coles, D. E. 1954 The problem of the turbulent boundary layer. Z. Angew. Math. Phys. 5, 181–203.CrossRefGoogle Scholar
12. Durst, F., Kikura, H., Lekakis, I., Jovanovic, J. & Ye, Q. 1996 Wall shear stress determination from near-wall mean velocity data in turbulent pipe and channel flows. Exp. Fluids 20, 417–428.CrossRefGoogle Scholar
13. Emmons, H. W. 1951 The laminar–turbulent transition in a boundary layer. Part 1. J. Aero. Sci. 18, 490–498.CrossRefGoogle Scholar
14. Erm, L. P. & Joubert, P. N. 1991 Low-Reynolds-number turbulent boundary layers. J. Fluid Mech. 230, 1–44.CrossRefGoogle Scholar
15. Fernholz, H. H. & Finley, P. J. 1996 The incompressible zero-pressure-gradient turbulent boundary layer: an assessment of the data. Prog. Aerosp. Sci. 32, 245–311.CrossRefGoogle Scholar
16. Ferrante, A. & Elghobashi, S. E. 2005 Reynolds number effect on drag reduction in a microbubble-laden spatially developing turbulent boundary layer. J. Fluid Mech. 543, 93–106.CrossRefGoogle Scholar
17. Gad-el-Hak, M. 2009 DNS of turbulent boundary layers: the breakthrough that opened a can of worms. CFD Lett. 1 (2), ii–iv.Google Scholar
18. Inoue, M. & Pullin, D. I. 2011 Large-eddy simulation of the zero-pressure-gradient turbulent boundary layer up to . J. Fluid Mech. 686, 507–533.CrossRefGoogle Scholar
19. Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
20. Jiménez, J., Hoyas, S., Simens, M. P. & Mizuno, Y. 2010 Turbulent boundary layers and channels at moderate Reynolds numbers. J. Fluid Mech. 657, 335–360.CrossRefGoogle Scholar
21. Jiménez, J., Simens, M. P., Hoyas, S. & Mizuno, Y. 2008 Entry length requirements for direct simulations of turbulent boundary layers. Center for Turbulence Research, Annual Research Briefs 381–390.Google Scholar
22. Kendall, A. & Koochesfahani, M. M. 2008 A method for estimating wall friction in turbulent wall-bounded flows. Exp. Fluids 44, 773–780.CrossRefGoogle Scholar
23. Khujadze, G. & Oberlack, M. 2004 DNS and scaling laws from new symmetry groups of ZPG turbulent boundary layer flow. Theor. Comput. Fluid Dyn. 18, 391–411.CrossRefGoogle Scholar
24. Khujadze, G. & Oberlack, M. 2007 New scaling laws in ZPG turbulent boundary layer flow. In Proc. 5th Intl Symp. on Turbulence and Shear Flow Phenomena, München, Germany (ed. R. Friedrich, N. A. Adams, J. K. Eaton, J. A. C. Humphrey, N. Kasagi & M. A. Leschziner).CrossRefGoogle Scholar
25. Kim, J., Moin, P. & Moser, R. D. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
26. Klebanoff, P. S. & Diehl, W. S. 1954 Some features of artificially thickened fully developed turbulent boundary layers with zero pressure-gradient. NACA Tech. Rep. 1110.Google Scholar
27. Komminaho, J. & Skote, M. 2002 Reynolds stress budgets in couette and boundary layer flows. Flow Turbul. Combust. 68, 167–192.CrossRefGoogle Scholar
28. Landahl, M. T. 1980 A note on an algebraic instability of inviscid parallel shear flows. J. Fluid Mech. 98, 243–251.CrossRefGoogle Scholar
29. Lee, J. H. & Sung, H. J. 2011 Direct numerical simulation of a turbulent boundary layer up to . Intl J. Heat Fluid Flow 32, 1–10.CrossRefGoogle Scholar
30. Marusic, I. 2009 Unravelling turbulence near walls. J. Fluid Mech. 630, 1–4.CrossRefGoogle Scholar
31. Marusic, I., McKeon, B. J., Monkewitz, P. A., Nagib, H. M., Smits, A. J. & Sreenivasan, K. R. 2010 Wall-bounded turbulent flows at high Reynolds numbers: recent advances and key issues. Phys. Fluids 22, 065103.CrossRefGoogle Scholar
32. McKeon, B. J., Li, J. D., Jiang, W., Morrison, J. F. & Smits, A. J. 2003 Pitot probe corrections in fully developed turbulent pipe flow. Meas. Sci. Technol. 14, 1449–1458.CrossRefGoogle Scholar
33. Monkewitz, P. A., Chauhan, K. A. & Nagib, H. M. 2007 Self-consistent high-Reynolds-number asymptotics for zero-pressure-gradient turbulent boundary layers. Phys. Fluids 19, 115101.CrossRefGoogle Scholar
34. Monty, J. P. & Chong, M. S. 2009 Turbulent channel flow: comparison of streamwise velocity data from experiments and direct numerical simulation. J. Fluid Mech. 633, 461–474.CrossRefGoogle Scholar
35. Murlis, J., Tsai, H. & Bradshaw, P. 1982 The structure of turbulent boundary layers at low Reynolds numbers. J. Fluid Mech. 122, 13–56.CrossRefGoogle Scholar
36. Nordström, J., Nordin, N. & Henningson, D. S. 1999 The fringe region technique and the Fourier method used in the direct numerical simulation of spatially evolving viscous flows. SIAM J. Sci. Comput. 20 (4), 1365–1393.CrossRefGoogle Scholar
37. Örlü, R., Fransson, J. H. M. & Alfredsson, P. H. 2010 On near wall measurements of wall bounded flows – the necessity of an accurate determination of the wall position. Prog. Aerosp. Sci. 46, 353–387.CrossRefGoogle Scholar
38. Örlü, R. & Schlatter, P. 2011 On the fluctuating wall shear stress in zero pressure-gradient turbulent boundary layer flows. Phys. Fluids 23, 021704.CrossRefGoogle Scholar
39. Örlü, R. & Schlatter, P. 2012 Turbulent boundary layer flow: comparing experiments with DNS. In Progress in Turbulence and Wind Energy IV. iTi Conference, September 19–22 2010 (ed. Oberlack, M., Peinke, J., Talamelli, A., Castillo, L. & Hölling, M. ), pp. 213–216. Springer.CrossRefGoogle Scholar
40. Österlund, J. M., Johansson, A. V., Nagib, H. M. & Hites, M. H. 2000 A note on the overlap region in turbulent boundary layers. Phys. Fluids 12, 1–4.CrossRefGoogle Scholar
41. Schlatter, P., Chevalier, M., Ilak, M. & Henningson, D. S. 2010 a The structure of a turbulent boundary layer studied by numerical simulation. Preprint http://arxiv.org/abs/1010.4000.Google Scholar
42. Schlatter, P., Deusebio, E., de Lange, R. & Brandt, L. 2010b Numerical study of the stabilisation of boundary-layer disturbances by finite amplitude streaks. Intl J. Flow Control 2 (4), 259–288.CrossRefGoogle Scholar
43. Schlatter, P., Li, Q., Brethouwer, G., Johansson, A. V. & Henningson, D. S. 2010c Simulations of spatially evolving turbulent boundary layers up to . Intl J. Heat Fluid Flow 31, 251–261.CrossRefGoogle Scholar
44. Schlatter, P. & Örlü, R. 2010 Assessment of direct numerical simulation data of turbulent boundary layers. J. Fluid Mech. 659, 116–126.CrossRefGoogle Scholar
45. Schlatter, P., Örlü, R., Li, Q., Brethouwer, G., Fransson, J. H. M., Johansson, A. V., Alfredsson, P. H. & Henningson, D. S. 2009 Turbulent boundary layers up to studied through simulation and experiment. Phys. Fluids 21, 051702.CrossRefGoogle Scholar
46. Seo, J., Castillo, L., Johansson, T. G. & Hangan, H. 2004 Reynolds stress in turbulent boundary layers at high Reynolds number. J. Turbul. 5 (15), 1–22.CrossRefGoogle Scholar
47. Sillero, J., Jiménez, J., Moser, R. D. & Malaya, N. P. 2011 Direct simulation of a zero-pressure-gradient turbulent boundary layer up to . J. Phys.: Conf. Ser. 318, 022023.Google Scholar
48. Simens, M. P., Jiménez, J., Hoyas, S. & Mizuno, Y. 2009 A high-resolution code for turbulent boundary layers. J. Comput. Phys. 228, 4218–4231.CrossRefGoogle Scholar
49. Skote, M., Haritonidis, J. H. & Henningson, D. S. 2002 Varicose instabilities in turbulent boundary layers. Phys. Fluids 14, 2309–2323.CrossRefGoogle Scholar
50. Smits, A. J., Matheson, N. & Joubert, P. N. 1983 Low-Reynolds-number turbulent boundary layers in zero and favourable pressure-gradients. J. Ship Res. 27, 147–157.CrossRefGoogle Scholar
51. Spalart, P. R. 1988 a Direct numerical study of leading edge contamination. In Fluid Dynamics of Three-Dimensional Turbulent Shear Flows and Transition, AGARD CP-438, pp. 5.1–5.13. AGARD.Google Scholar
52. Spalart, P. R. 1988b Direct simulation of a turbulent boundary layer up to =1410. J. Fluid Mech. 187, 61–98.CrossRefGoogle Scholar
53. Wu, X. 2010 Establishing the generality of three phenomena using a boundary layer with free stream passing wakes. J. Fluid Mech. 664, 193–219.CrossRefGoogle Scholar
54. Wu, X. & Moin, P. 2009a Direct numerical simulation of turbulence in a nominally zero-pressure-gradient flat-plate boundary layer. J. Fluid Mech. 630, 5–41.CrossRefGoogle Scholar
55. Wu, X. & Moin, P. 2009b Forest of hairpins in a low-Reynolds-number zero-pressure-gradient flat-plate boundary layer. Phys. Fluids 21, 091106.CrossRefGoogle Scholar
56. Wu, X. & Moin, P. 2010 Transitional and turbulent boundary layer with heat transfer. Phys. Fluids 22, 085105.CrossRefGoogle Scholar