Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chkhetiani, Otto G.
and
Gledzer, Evgeny B.
2017.
Helical turbulence with small-scale energy and helicity sources and external intermediate scale noises as the origin of large scale generation.
Physica A: Statistical Mechanics and its Applications,
Vol. 486,
Issue. ,
p.
416.
Ali, Sk Zeeshan
and
Dey, Subhasish
2017.
Origin of the scaling laws of sediment transport.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 473,
Issue. 2197,
p.
20160785.
Coscarella, F.
Servidio, S.
Ferraro, D.
Carbone, V.
and
Gaudio, R.
2017.
Turbulent energy dissipation rate in a tilting flume with a highly rough bed.
Physics of Fluids,
Vol. 29,
Issue. 8,
Padhi, Ellora
Penna, Nadia
Dey, Subhasish
and
Gaudio, Roberto
2018.
Spatially averaged dissipation rate in flows over water-worked and screeded gravel beds.
Physics of Fluids,
Vol. 30,
Issue. 12,
p.
125106.
Fang, Hongwei
Han, Xu
He, Guojian
and
Dey, Subhasish
2018.
Influence of permeable beds on hydraulically macro-rough flow.
Journal of Fluid Mechanics,
Vol. 847,
Issue. ,
p.
552.
Padhi, Ellora
Penna, Nadia
Dey, Subhasish
and
Gaudio, Roberto
2018.
Hydrodynamics of water-worked and screeded gravel beds: A comparative study.
Physics of Fluids,
Vol. 30,
Issue. 8,
Ali, Sk Zeeshan
and
Dey, Subhasish
2018.
Impact of phenomenological theory of turbulence on pragmatic approach to fluvial hydraulics.
Physics of Fluids,
Vol. 30,
Issue. 4,
Padhi, Ellora
Penna, Nadia
Gaudio, Roberto
Desai, V. R.
Dey, Subhasish
Paquier, A.
and
Rivière, N.
2018.
Turbulent kinetic energy flux and budget in a water-worked gravel bed.
E3S Web of Conferences,
Vol. 40,
Issue. ,
p.
05006.
Ferraro, D.
Servidio, S.
and
Gaudio, R.
2019.
Velocity scales in steady-nonuniform turbulent flows with low relative submergence.
Environmental Fluid Mechanics,
Vol. 19,
Issue. 4,
p.
1025.
Padhi, Ellora
Penna, Nadia
Dey, Subhasish
and
Gaudio, Roberto
2019.
Near-bed turbulence structures in water-worked and screeded gravel-bed flows.
Physics of Fluids,
Vol. 31,
Issue. 4,
Dey, Subhasish
Ali, Sk Zeeshan
and
Padhi, Ellora
2019.
Bedload transport from analytical and turbulence phenomenological perspectives.
International Journal of Sediment Research,
Vol. 34,
Issue. 6,
p.
509.
Ferraro, D.
Coscarella, F.
and
Gaudio, R.
2019.
Scales of turbulence in open-channel flows with low relative submergence.
Physics of Fluids,
Vol. 31,
Issue. 12,
Alfonsi, G.
Ferraro, D.
Lauria, A.
and
Gaudio, R.
2019.
Large-eddy simulation of turbulent natural-bed flow.
Physics of Fluids,
Vol. 31,
Issue. 8,
p.
085105.
Dey, Subhasish
Ravi Kishore, Galla
Castro-Orgaz, Oscar
and
Ali, Sk Zeeshan
2019.
Turbulent Length Scales and Anisotropy in Submerged Turbulent Plane Offset Jets.
Journal of Hydraulic Engineering,
Vol. 145,
Issue. 2,
Padhi, Ellora
Penna, Nadia
Dey, Subhasish
and
Gaudio, Roberto
2020.
Recent Trends in Environmental Hydraulics.
p.
207.
Coscarella, F.
Penna, N.
Servidio, S.
and
Gaudio, R.
2020.
Turbulence anisotropy and intermittency in open-channel flows on rough beds.
Physics of Fluids,
Vol. 32,
Issue. 11,
Padhi, Ellora
Dey, Subhasish
Penna, Nadia
and
Gaudio, Roberto
2020.
Conditional Turbulence Characteristics in Water-Worked and Screeded Gravel-Bed Flows.
Journal of Hydraulic Engineering,
Vol. 146,
Issue. 2,
Alfonsi, Giancarlo
Ferraro, Domenico
Lauria, Agostino
and
Gaudio, Roberto
2020.
Numerical Computations: Theory and Algorithms.
Vol. 11974,
Issue. ,
p.
280.
Penna, Nadia
Padhi, Ellora
Dey, Subhasish
and
Gaudio, Roberto
2020.
Structure functions and invariants of the anisotropic Reynolds stress tensor in turbulent flows on water-worked gravel beds.
Physics of Fluids,
Vol. 32,
Issue. 5,
Rathore, Vijit
Dey, Subhasish
Penna, Nadia
and
Gaudio, Roberto
2021.
Turbulent flow characteristics over an abrupt step change in bed roughness.
Physics of Fluids,
Vol. 33,
Issue. 9,
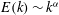 $E(k)\sim k^{{\it\alpha}}$, suggesting the presence of inertial range turbulence. The exponent
$E(k)\sim k^{{\it\alpha}}$, suggesting the presence of inertial range turbulence. The exponent  ${\it\alpha}$ is slightly shallower than the Kolmogorov
${\it\alpha}$ is slightly shallower than the Kolmogorov 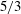 $5/3$ scaling law, with this deviation possibly due to the bed roughness heterogeneity and to fluctuation anisotropy. The Taylor frozen-in approximation is broken at smaller scales towards the roughness crest level; therefore, a new statistical tool for the validation of this approximation is proposed. The Kolmogorov
$5/3$ scaling law, with this deviation possibly due to the bed roughness heterogeneity and to fluctuation anisotropy. The Taylor frozen-in approximation is broken at smaller scales towards the roughness crest level; therefore, a new statistical tool for the validation of this approximation is proposed. The Kolmogorov 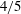 $4/5$-law for the longitudinal increments and simultaneously the Monin–Yaglom
$4/5$-law for the longitudinal increments and simultaneously the Monin–Yaglom 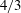 $4/3$-law for the nonlinear normal fluxes (both in physical space) are preserved, providing an accurate estimation of the turbulent kinetic energy dissipation rate. The heterogeneity of the bed acts to induce the transport of finite kinetic helicity to the outer layer through persistently prolonged vortices. An associated
$4/3$-law for the nonlinear normal fluxes (both in physical space) are preserved, providing an accurate estimation of the turbulent kinetic energy dissipation rate. The heterogeneity of the bed acts to induce the transport of finite kinetic helicity to the outer layer through persistently prolonged vortices. An associated 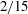 $2/15$-law for the cascade of helicity has been locally found. These findings open a new direction in turbulence research for flows over highly rough beds.
$2/15$-law for the cascade of helicity has been locally found. These findings open a new direction in turbulence research for flows over highly rough beds.