Published online by Cambridge University Press: 14 April 2016
Turbulence in the asymptotic suction boundary layer is investigated numerically at the verge of laminarisation using direct numerical simulation. Following an adiabatic protocol, the Reynolds number 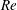 $Re$ is decreased in small steps starting from a fully turbulent state until laminarisation is observed. Computations in a large numerical domain allow in principle for the possible coexistence of laminar and turbulent regions. However, contrary to other subcritical shear flows, no laminar–turbulent coexistence is observed, even near the onset of sustained turbulence. High-resolution computations suggest a critical Reynolds number
$Re$ is decreased in small steps starting from a fully turbulent state until laminarisation is observed. Computations in a large numerical domain allow in principle for the possible coexistence of laminar and turbulent regions. However, contrary to other subcritical shear flows, no laminar–turbulent coexistence is observed, even near the onset of sustained turbulence. High-resolution computations suggest a critical Reynolds number 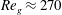 $Re_{g}\approx 270$, below which turbulence collapses, based on observation times of
$Re_{g}\approx 270$, below which turbulence collapses, based on observation times of 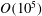 $O(10^{5})$ inertial time units. During the laminarisation process, the turbulent flow fragments into a series of transient streamwise-elongated structures, whose interfaces do not display the characteristic obliqueness of classical laminar–turbulent patterns. The law of the wall, i.e. logarithmic scaling of the velocity profile, is retained down to
$O(10^{5})$ inertial time units. During the laminarisation process, the turbulent flow fragments into a series of transient streamwise-elongated structures, whose interfaces do not display the characteristic obliqueness of classical laminar–turbulent patterns. The law of the wall, i.e. logarithmic scaling of the velocity profile, is retained down to 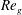 $Re_{g}$, suggesting a large-scale wall-normal transport absent in internal shear flows close to the onset. In order to test the effect of these large-scale structures on the near-wall region, an artificial volume force is added to damp spanwise and wall-normal fluctuations above
$Re_{g}$, suggesting a large-scale wall-normal transport absent in internal shear flows close to the onset. In order to test the effect of these large-scale structures on the near-wall region, an artificial volume force is added to damp spanwise and wall-normal fluctuations above 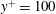 $y^{+}=100$, in viscous units. Once the largest eddies have been suppressed by the forcing, and thus turbulence is confined to the near-wall region, oblique laminar–turbulent interfaces do emerge as in other wall-bounded flows, however only transiently. These results suggest that oblique stripes at the onset are a prevalent feature of internal shear flows, but will not occur in canonical boundary layers, including the spatially growing ones.
$y^{+}=100$, in viscous units. Once the largest eddies have been suppressed by the forcing, and thus turbulence is confined to the near-wall region, oblique laminar–turbulent interfaces do emerge as in other wall-bounded flows, however only transiently. These results suggest that oblique stripes at the onset are a prevalent feature of internal shear flows, but will not occur in canonical boundary layers, including the spatially growing ones.