Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dadfar, R.
Fabbiane, N.
Bagheri, S.
and
Henningson, D. S.
2014.
Centralised Versus Decentralised Active Control of Boundary Layer Instabilities.
Flow, Turbulence and Combustion,
Vol. 93,
Issue. 4,
p.
537.
Brunton, Steven L.
and
Noack, Bernd R.
2015.
Closed-Loop Turbulence Control: Progress and Challenges.
Applied Mechanics Reviews,
Vol. 67,
Issue. 5,
Kriegseis, J
Maden, I
Schwarz, C
Tropea, C
and
Grundmann, S
2015.
Addendum to ‘velocity-information based force-term estimation of dielectric barrier discharge plasma actuators’.
Journal of Physics D: Applied Physics,
Vol. 48,
Issue. 32,
p.
329401.
Fabbiane, Nicolò
Simon, Bernhard
Fischer, Felix
Grundmann, Sven
Bagheri, Shervin
and
Henningson, Dan S.
2015.
On the role of adaptivity for robust laminar flow control.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
Dadfar, Reza
Hanifi, Ardeshir
and
Henningson, Dan S.
2015.
Feedback Control for Laminarization of flow over Wings.
Flow, Turbulence and Combustion,
Vol. 94,
Issue. 1,
p.
43.
Tol, Henry
de Visser, Coen C.
and
Kotsonis, Marios
2016.
Control of fluid flows using multivariate spline reduced order models.
Simon, Bernhard
Fabbiane, Nicolò
Nemitz, Timotheus
Bagheri, Shervin
Henningson, Dan S.
and
Grundmann, Sven
2016.
In-flight active wave cancelation with delayed-x-LMS control algorithm in a laminar boundary layer.
Experiments in Fluids,
Vol. 57,
Issue. 10,
Taira, Kunihiko
Brunton, Steven L.
Dawson, Scott T. M.
Rowley, Clarence W.
Colonius, Tim
McKeon, Beverley J.
Schmidt, Oliver T.
Gordeyev, Stanislav
Theofilis, Vassilios
and
Ukeiley, Lawrence S.
2017.
Modal Analysis of Fluid Flows: An Overview.
AIAA Journal,
Vol. 55,
Issue. 12,
p.
4013.
Semeraro, Onofrio
Lusseyran, François
Pastur, Luc
and
Jordan, Peter
2017.
Qualitative dynamics of wave packets in turbulent jets.
Physical Review Fluids,
Vol. 2,
Issue. 9,
Sasaki, Kenzo
Piantanida, Selene
Cavalieri, André V. G.
and
Jordan, Peter
2017.
Real-time modelling of wavepackets in turbulent jets.
Journal of Fluid Mechanics,
Vol. 821,
Issue. ,
p.
458.
Fabbiane, Nicolò
Bagheri, Shervin
and
Henningson, Dan S.
2017.
Energy efficiency and performance limitations of linear adaptive control for transition delay.
Journal of Fluid Mechanics,
Vol. 810,
Issue. ,
p.
60.
Tol, H. J.
Kotsonis, M.
de Visser, C. C.
and
Bamieh, B.
2017.
Localised estimation and control of linear instabilities in two-dimensional wall-bounded shear flows.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
818.
Rowley, Clarence W.
and
Dawson, Scott T.M.
2017.
Model Reduction for Flow Analysis and Control.
Annual Review of Fluid Mechanics,
Vol. 49,
Issue. 1,
p.
387.
Sasaki, Kenzo
Morra, Pierluigi
Fabbiane, Nicoló
Cavalieri, André V. G.
Hanifi, Ardeshir
and
Henningson, Dan S.
2018.
On the wave-cancelling nature of boundary layer flow control.
Theoretical and Computational Fluid Dynamics,
Vol. 32,
Issue. 5,
p.
593.
Dadfar, R.
Hanifi, A.
and
Henningson, D. S.
2018.
Control of Instabilities in an Unswept Wing Boundary Layer.
AIAA Journal,
Vol. 56,
Issue. 5,
p.
1750.
Priddin, Matthew
Baker, David I.
Ayton, Lorna J.
and
Peake, Nigel
2018.
Vortex Sound Models: Passive and Active Noise Control.
Semeraro, Onofrio
and
Pralits, Jan O.
2018.
Full-order optimal compensators for flow control: the multiple inputs case.
Theoretical and Computational Fluid Dynamics,
Vol. 32,
Issue. 3,
p.
285.
Tol, H. J.
Kotsonis, M.
and
De Visser, C. C.
2019.
Pressure Output Feedback Control of Tollmien–Schlichting Waves in Falkner–Skan Boundary Layers.
AIAA Journal,
Vol. 57,
Issue. 4,
p.
1538.
Tol, H. J.
de Visser, C. C.
and
Kotsonis, M.
2019.
Experimental Model-Based Estimation and Control of Natural Tollmien–Schlichting Waves.
AIAA Journal,
Vol. 57,
Issue. 6,
p.
2344.
Sashittal, Palash
and
Bodony, Daniel J.
2019.
Reduced-order control using low-rank dynamic mode decomposition.
Theoretical and Computational Fluid Dynamics,
Vol. 33,
Issue. 6,
p.
603.
 $a\approx 1\hspace{0.167em} \% $ of the free stream velocity at the actuator location. Up to these amplitudes, it is found that a proper choice of the actuators positively affects the performance of the controller. For a transitional case,
$a\approx 1\hspace{0.167em} \% $ of the free stream velocity at the actuator location. Up to these amplitudes, it is found that a proper choice of the actuators positively affects the performance of the controller. For a transitional case, 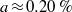 $a\approx 0. 20\hspace{0.167em} \% $, we show a transition delay of
$a\approx 0. 20\hspace{0.167em} \% $, we show a transition delay of 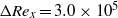 $\Delta {\mathit{Re}}_{x} = 3. 0\times 1{0}^{5} $.
$\Delta {\mathit{Re}}_{x} = 3. 0\times 1{0}^{5} $.