Published online by Cambridge University Press: 21 February 2023
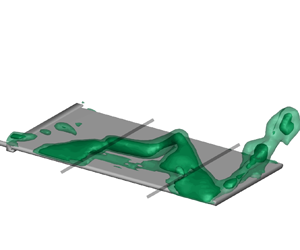
Plenoptic particle image velocimetry and surface pressure measurements were used to analyse the early development of leading-edge vortices (LEVs) created by a flat-plate wing of aspect ratio 2 rolling in a uniform flow parallel to the roll axis. Four cases were constructed by considering two advance coefficients,  $J=0.54$ and 1.36, and two wing radii of gyration,
$J=0.54$ and 1.36, and two wing radii of gyration,  $R_g/c=2.5$ and 3.25. In each case, the wing pitch angle was articulated such as to achieve an angle of attack of
$R_g/c=2.5$ and 3.25. In each case, the wing pitch angle was articulated such as to achieve an angle of attack of  $33^{\circ }$ at the radius of gyration of the wing. The sources and sinks of vorticity were quantified for a chordwise rectangular control region, using a vorticity transport framework in a non-inertial coordinate system attached to the wing. Within this framework, terms associated with Coriolis acceleration provide a correction to tilting and spanwise convective fluxes measured in the rotating frame and, for the present case, have insignificant values. For the baseline case (
$33^{\circ }$ at the radius of gyration of the wing. The sources and sinks of vorticity were quantified for a chordwise rectangular control region, using a vorticity transport framework in a non-inertial coordinate system attached to the wing. Within this framework, terms associated with Coriolis acceleration provide a correction to tilting and spanwise convective fluxes measured in the rotating frame and, for the present case, have insignificant values. For the baseline case ( $J=0.54, R_g/c=3.25$), three distinct spanwise regions were observed within the LEV, with distinct patterns of vortex evolution and vorticity transport mechanisms in each region. Reducing the radius of gyration to
$J=0.54, R_g/c=3.25$), three distinct spanwise regions were observed within the LEV, with distinct patterns of vortex evolution and vorticity transport mechanisms in each region. Reducing the radius of gyration to  $R_g/c=2.5$ resulted in a more stable vortex with the inboard region extending over a broader spanwise range. Increasing advance ratio eliminated the conical vortex, resulting in transport processes resembling the mid-span region of the baseline case. Although the circulation of the LEV system was generally stronger at the larger advance coefficient, the shear-layer contribution was diminished.
$R_g/c=2.5$ resulted in a more stable vortex with the inboard region extending over a broader spanwise range. Increasing advance ratio eliminated the conical vortex, resulting in transport processes resembling the mid-span region of the baseline case. Although the circulation of the LEV system was generally stronger at the larger advance coefficient, the shear-layer contribution was diminished.