Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-29T07:28:43.617Z Has data issue: false hasContentIssue false
  • English
  • Français

Transient flows and migration in granular suspensions: key role of Reynolds-like dilatancy

Published online by Cambridge University Press:  23 September 2022

S. Athani ,
B. Metzger Open the ORCID record for B. Metzger [Opens in a new window] ,
Y. Forterre  and
R. Mari Open the ORCID record for R. Mari [Opens in a new window]
S. Athani*
Affiliation:
Université Grenoble–Alpes, CNRS, Laboratoire Interdisciplinaire de Physique (LIPhy), 38000 Grenoble, France
B. Metzger
Affiliation:
Aix Marseille Univ., CNRS, IUSTI, 13453 Marseille, France
Y. Forterre
Affiliation:
Aix Marseille Univ., CNRS, IUSTI, 13453 Marseille, France
R. Mari
Affiliation:
Université Grenoble–Alpes, CNRS, Laboratoire Interdisciplinaire de Physique (LIPhy), 38000 Grenoble, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

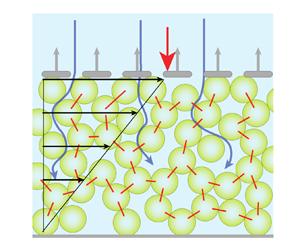
We investigate the transient dynamics of a sheared suspension of neutrally buoyant particles in pressure-imposed rheology configuration, subject to a sudden change in shear rate or external pressure. Discrete element method simulations show that, depending on the flow parameters (particle and system size, initial volume fraction), the early stress response of the suspension may differ strongly from the prediction of the suspension balance model based on the steady-state rheology. We show that a two-phase model incorporating the Reynolds-like dilatancy law of Pailha & Pouliquen (J. Fluid Mech., vol. 633, 2009, pp. 115–135), which prescribes the dilation rate of the suspension over a strain scale $\gamma _0$, captures quantitatively the suspension dilation/compaction over the whole range of parameters investigated. Together with the Darcy flow induced by the pore pressure gradient during dilation or compaction, this Reynolds-like dilatancy implies that the early stress response of the suspension is non-local, with a non-local length scale $\ell$ that scales with the particle size and diverges algebraically at jamming. In regions affected by $\ell$, the stress level is fixed, not by the steady-state rheology, but by the Darcy fluid pressure gradient resulting from the dilation/compaction rate. Our results extend the validity of the Reynolds-like dilatancy flow rule, initially proposed for jammed suspensions, to flowing suspension below the critical volume fraction at which the suspension jams, thereby providing a unified framework to describe dilation and shear-induced migration. They pave the way for understanding more complex unsteady flows of dense suspensions, such as impacts, transient avalanches or the impulsive response of shear-thickening suspensions.

JFM classification

Complex Fluids: Suspensions Low-Reynolds-number flows: Stokesian dynamics Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 949 , 25 October 2022 , A9
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Acrivos, A., Mauri, R. & Fan, X. 1993 Shear-induced resuspension in a Couette device. Intl J. Multiphase Flow 19 (5), 797–802.CrossRefGoogle Scholar
Athani, S., Forterre, Y., Metzger, B. & Mari, R. 2021 Transients in pressure-imposed shearing of dense granular suspensions. In EPJ Web of Conferences, vol. 249, article no. 09009. EDP Sciences.CrossRefGoogle Scholar
Bouchut, F., Fernández-Nieto, E.D., Mangeney, A. & Narbona-Reina, G. 2016 A two-phase two-layer model for fluidized granular flows with dilatancy effects. J.Fluid Mech. 801, 166–221.CrossRefGoogle Scholar
Bougouin, A. & Lacaze, L. 2018 Granular collapse in a fluid: different flow regimes for an initially dense-packing. Phys. Rev. Fluids 3 (6), 064305.CrossRefGoogle Scholar
Boyer, F., Guazzelli, É. & Pouliquen, O. 2011 Unifying suspension and granular rheology. Phys. Rev. Lett. 107 (18), 188301.CrossRefGoogle ScholarPubMed
Boyer, F., Sandoval-Nava, E., Snoeijer, J.H., Dijksman, J.F. & Lohse, D. 2016 Drop impact of shear thickening liquids. Phys. Rev. Fluids 1 (1), 013901.CrossRefGoogle Scholar
Brady, J.F. & Bossis, G. 1988 Stokesian dynamics. Annu. Rev. Fluid Mech. 20 (1), 111–157.CrossRefGoogle Scholar
Brassard, M.-A., Causley, N., Krizou, N., Dijksman, J.A. & Clark, A.H. 2021 Viscous-like forces control the impact response of shear-thickening dense suspensions. J. Fluid Mech. 923, A38.Google Scholar
Chacko, R.N., Mari, R., Cates, M.E. & Fielding, S.M. 2018 a Dynamic vorticity banding in discontinuously shear thickening suspensions. Phys. Rev. Lett. 121 (10), 108003.CrossRefGoogle ScholarPubMed
Chacko, R.N., Mari, R., Fielding, S.M. & Cates, M.E. 2018 b Shear reversal in dense suspensions: the challenge to fabric evolution models from simulation data. J. Fluid Mech. 847, 700–734.CrossRefGoogle Scholar
Corté, L., Gerbode, S.J., Man, W. & Pine, D.J. 2009 Self-organized criticality in sheared suspensions. Phys. Rev. Lett. 103 (24), 248301.CrossRefGoogle ScholarPubMed
Cundall, P.A. & Strack, O.D.L. 1979 A discrete numerical model for granular assemblies. Gèotechnique 29 (1), 47–65.CrossRefGoogle Scholar
d'Ambrosio, E., Blanc, F. & Lemaire, E. 2021 Viscous resuspension of non-Brownian particles: determination of the concentration profiles and particle normal stresses. J. Fluid Mech. 911, A22.CrossRefGoogle Scholar
Davis, R.H. & Acrivos, A. 1985 Sedimentation of noncolloidal particles at low Reynolds numbers. Annu. Rev. Fluid Mech. 17 (1), 91–118.CrossRefGoogle Scholar
Deboeuf, A., Gauthier, G., Martin, J., Yurkovetsky, Y. & Morris, J.F. 2009 Particle pressure in a sheared suspension: a bridge from osmosis to granular dilatancy. Phys. Rev. Lett. 102 (10), 108301.CrossRefGoogle Scholar
DeGiuli, E., Düring, G., Lerner, E. & Wyart, M. 2015 Unified theory of inertial granular flows and non-Brownian suspensions. Phys. Rev. E 91 (6), 062206.CrossRefGoogle ScholarPubMed
Denn, M.M. & Morris, J.F. 2014 Rheology of non-Brownian suspensions. Annu. Rev. Chem. Biomol. Engng. 5 (1), 203–228.CrossRefGoogle ScholarPubMed
Gadala-Maria, F. & Acrivos, A. 1980 Shear-induced structure in a concentrated suspension of solid spheres. J. Rheol. 24 (6), 799–814.CrossRefGoogle Scholar
Gallier, S., Lemaire, E., Peters, F. & Lobry, L. 2014 Rheology of sheared suspensions of rough frictional particles. J. Fluid Mech. 757, 514–549.CrossRefGoogle Scholar
Gillissen, J.J.J. & Wilson, H.J. 2018 Modeling sphere suspension microstructure and stress. Phys. Rev. E 98 (3), 033119.CrossRefGoogle Scholar
Goyon, J., Colin, A., Ovarlez, G., Ajdari, A. & Bocquet, L. 2008 Spatial cooperativity in soft glassy flows. Nature 454 (7200), 84–87.CrossRefGoogle ScholarPubMed
Grishaev, V., Iorio, C.S., Dubois, F. & Amirfazli, A. 2015 Complex drop impact morphology. Langmuir 31 (36), 9833–9844.CrossRefGoogle ScholarPubMed
Guazzelli, É. & Pouliquen, O. 2018 Rheology of dense granular suspensions. J. Fluid Mech. 852, P1.CrossRefGoogle Scholar
Han, E., Peters, I.R. & Jaeger, H.M. 2016 High-speed ultrasound imaging in dense suspensions reveals impact-activated solidification due to dynamic shear jamming. Nat. Commun. 7 (1), 12243.CrossRefGoogle ScholarPubMed
Han, E., Wyart, M., Peters, I.R. & Jaeger, H.M. 2018 Shear fronts in shear-thickening suspensions. Phys. Rev. Fluids 3 (7), 073301.CrossRefGoogle Scholar
Iverson, R.M. 2012 Elementary theory of bed-sediment entrainment by debris flows and avalanches. J. Geophys. Res. 117 (F3), F03006.Google Scholar
Iverson, R.M., Reid, M.E., Iverson, N.R., LaHusen, R.G., Logan, M., Mann, J.E. & Brien, D.L. 2000 Acute sensitivity of landslide rates to initial soil porosity. Science 290 (5491), 513–516.CrossRefGoogle ScholarPubMed
Jackson, R. 1997 Locally averaged equations of motion for a mixture of identical spherical particles and a Newtonian fluid. Chem. Engng Sci. 52 (15), 2457–2469.CrossRefGoogle Scholar
Jackson, R. 2000 The Dynamics of Fluidized Particles. Cambridge University Press.Google Scholar
Jeffrey, D.J. 1992 The calculation of the low Reynolds number resistance functions for two unequal spheres. Phys. Fluids A 4 (1), 16–29.CrossRefGoogle Scholar
Jerome, J.J.S., Vandenberghe, N. & Forterre, Y. 2016 Unifying impacts in granular matter from quicksand to cornstarch. Phys. Rev. Lett. 117 (9), 098003.CrossRefGoogle ScholarPubMed
Jørgensen, L., Forterre, Y. & Lhuissier, H. 2020 Deformation upon impact of a concentrated suspension drop. J. Fluid Mech. 896, R2.CrossRefGoogle Scholar
Kamrin, K. & Koval, G. 2012 Nonlocal constitutive relation for steady granular flow. Phys. Rev. Lett. 108 (17), 178301.CrossRefGoogle ScholarPubMed
Kulkarni, S.D., Metzger, B. & Morris, J.F. 2010 Particle-pressure-induced self-filtration in concentrated suspensions. Phys. Rev. E 82 (1), 010402.CrossRefGoogle ScholarPubMed
Lee, C.-H. 2021 Two-phase modelling of submarine granular flows with shear-induced volume change and pore-pressure feedback. J. Fluid Mech. 907, A31.CrossRefGoogle Scholar
Mari, R., Seto, R., Morris, J.F. & Denn, M.M. 2014 Shear thickening, frictionless and frictional rheologies in non-Brownian suspensions. J. Rheol. 58 (6), 1693–1724.CrossRefGoogle Scholar
Mari, R., Seto, R., Morris, J.F. & Denn, M.M. 2015 Nonmonotonic flow curves of shear thickening suspensions. Phys. Rev. E 91 (5), 052302.CrossRefGoogle ScholarPubMed
Metzger, B. & Butler, J.E. 2012 Clouds of particles in a periodic shear flow. Phys. Fluids 24 (2), 021703.CrossRefGoogle Scholar
Montellà, E.P., Chauchat, J., Chareyre, B., Bonamy, C. & Hsu, T.J. 2021 A two-fluid model for immersed granular avalanches with dilatancy effects. J. Fluid Mech. 925, A13.CrossRefGoogle Scholar
Morris, J.F. & Boulay, F. 1999 Curvilinear flows of noncolloidal suspensions: the role of normal stresses. J. Rheol. 43 (5), 1213–1237.CrossRefGoogle Scholar
Morris, J.F. & Brady, J.F. 1998 Pressure-driven flow of a suspension: buoyancy effects. Intl J. Multiphase Flow 24 (1), 105–130.CrossRefGoogle Scholar
Ness, C., Seto, R. & Mari, R. 2022 The physics of dense suspensions. Annu. Rev. Condens. Matter Phys. 13 (1), 97–117.CrossRefGoogle Scholar
Nicolas, M. 2005 Spreading of a drop of neutrally buoyant suspension. J. Fluid Mech. 545, 271–280.CrossRefGoogle Scholar
Nott, P.R. & Brady, J.F. 1994 Pressure-driven flow of suspensions: simulation and theory. J. Fluid Mech. 275, 157–199.CrossRefGoogle Scholar
Nott, P.R., Guazzelli, E. & Pouliquen, O. 2011 The suspension balance model revisited. Phys. Fluids 23 (4), 043304.CrossRefGoogle Scholar
Pailha, M., Nicolas, M. & Pouliquen, O. 2008 Initiation of underwater granular avalanches: influence of the initial volume fraction. Phys. Fluids 20 (11), 111701.CrossRefGoogle Scholar
Pailha, M. & Pouliquen, O. 2009 A two-phase flow description of the initiation of underwater granular avalanches. J. Fluid Mech. 633, 115–135.CrossRefGoogle Scholar
Peters, F., Giovanni, G., Gallier, S., Blanc, F., Lemaire, E. & Lobry, L. 2016 Rheology of non-Brownian suspensions of rough frictional particles under shear reversal: a numerical study. J. Rheol. 60 (4), 715–732.CrossRefGoogle Scholar
Peters, I.R., Xu, Q. & Jaeger, H.M. 2013 Splashing onset in dense suspension droplets. Phys. Rev. Lett. 111 (2), 028301.CrossRefGoogle ScholarPubMed
Pham, P., Butler, J.E. & Metzger, B. 2016 Origin of critical strain amplitude in periodically sheared suspensions. Phys. Rev. Fluids 1 (2), 022201.CrossRefGoogle Scholar
Pine, D.J., Gollub, J.P., Brady, J.F. & Leshansky, A.M. 2005 Chaos and threshold for irreversibility in sheared suspensions. Nature 438 (7070), 997–1000.CrossRefGoogle ScholarPubMed
Ren, J., Dijksman, J.A. & Behringer, R.P. 2013 Reynolds pressure and relaxation in a sheared granular system. Phys. Rev. Lett. 110 (1), 018302.CrossRefGoogle Scholar
Reynolds, O. 1885 LVII. On the dilatancy of media composed of rigid particles in contact. With experimental illustrations. Lond. Edinb. Dublin Philos. Mag. J. Sci. 20 (127), 469–481.CrossRefGoogle Scholar
Richardson, J.F. & Zaki, W.N. 1954 Sedimentation and fluidisation: part I. Trans. Inst. Chem. Engrs 32, 35–53.Google Scholar
Rondon, L., Pouliquen, O. & Aussillous, P. 2011 Granular collapse in a fluid: role of the initial volume fraction. Phys. Fluids 23 (7), 073301.CrossRefGoogle Scholar
Roux, S. & Radjaï, F. 1998 Texture-dependent rigid-plastic behavior. In Physics of Dry Granular Media, NATO ASI Series (ed. H.J. Herrmann, J.P. Hovi & S. Luding), pp. 229–236. Springer.CrossRefGoogle Scholar
Roux, S. & Radjaï, F. 2002 Statistical Approach to the Mechanical Behavior of Granular Media. Springer.CrossRefGoogle Scholar
Saint-Michel, B., Manneville, S., Meeker, S., Ovarlez, G. & Bodiguel, H. 2019 X-ray radiography of viscous resuspension. Phys. Fluids 31 (10), 103301.CrossRefGoogle Scholar
Sarabian, M., Firouznia, M., Metzger, B. & Hormozi, S. 2019 Fully developed and transient concentration profiles of particulate suspensions sheared in a cylindrical Couette cell. J. Fluid Mech. 862, 659–671.CrossRefGoogle Scholar
Schaarsberg, M.H.K., Peters, I.R., Stern, M., Dodge, K., Zhang, W.W. & Jaeger, H.M. 2016 From splashing to bouncing: the influence of viscosity on the impact of suspension droplets on a solid surface. Phys. Rev. E 93 (6), 062609.CrossRefGoogle Scholar
Seto, R., Mari, R., Morris, J.F. & Denn, M.M. 2013 Discontinuous shear thickening of frictional hard-sphere suspensions. Phys. Rev. Lett. 111 (21), 218301.CrossRefGoogle ScholarPubMed
Snook, B., Butler, J.E. & Guazzelli, É. 2016 Dynamics of shear-induced migration of spherical particles in oscillatory pipe flow. J. Fluid Mech. 786, 128–153.CrossRefGoogle Scholar
Terzaghi, K. 1943 Theoretical Soil Mechanics. John Wiley & Sons, Inc.CrossRefGoogle Scholar
Topin, V., Monerie, Y., Perales, F. & Radjaï, F. 2012 Collapse dynamics and runout of dense granular materials in a fluid. Phys. Rev. Lett. 109 (18), 188001.CrossRefGoogle Scholar
Waitukaitis, S.R. & Jaeger, H.M. 2012 Impact-activated solidification of dense suspensions via dynamic jamming fronts. Nature 487 (7406), 205–209.CrossRefGoogle ScholarPubMed
Wood, D.M. 1990 Soil Behaviour and Critical State Soil Mechanics. Cambridge University Press.Google Scholar
Wyart, M. & Cates, M.E. 2014 Discontinuous shear thickening without inertia in dense non-Brownian suspensions. Phys. Rev. Lett. 112 (9), 098302.CrossRefGoogle ScholarPubMed